Processing Uncertainties (DP IB Physics): Revision Note
Processing uncertainties
What is uncertainty?
Uncertainty is a quantitative indication of the quality of the result
It is the difference between the actual reading taken (caused by the equipment or techniques used) and the true value
It is a range of values around a measurement within which the true value is expected to lie, and is an estimate
Uncertainties are not the same as errors
Errors arise from equipment or practical techniques that cause a reading to be different from the true value
Uncertainties in measurements are recorded as a range (±) to an appropriate level of precision
Table showing different uncertainties
| Uncertainty |
|---|---|
in a reading | ± half the smallest division |
in a measurement | at least ±1 smallest division |
in repeated data | half the range |
in digital readings | ± the last significant digit |
Types of uncertainty
Uncertainty can be expressed in one of three main forms
Absolute uncertainty
The actual amount by which the measurement is uncertain
e.g.if
= 5.0 ± 0.1 cm, the absolute uncertainty in
is
= 0.1 cm
Fractional uncertainty
The uncertainty in the measurement expressed as a fraction
e.g.if
= 5.0 ± 0.1 cm, the fractional uncertainty in
is
Percentage uncertainty
The uncertainty in the measurement expressed as a percentage
e.g.if
= 5.0 ± 0.1 cm, the percentage uncertainty in
is
= 2%
In general, it is calculated using the following formula:
How to calculate absolute, fractional and percentage uncertainty
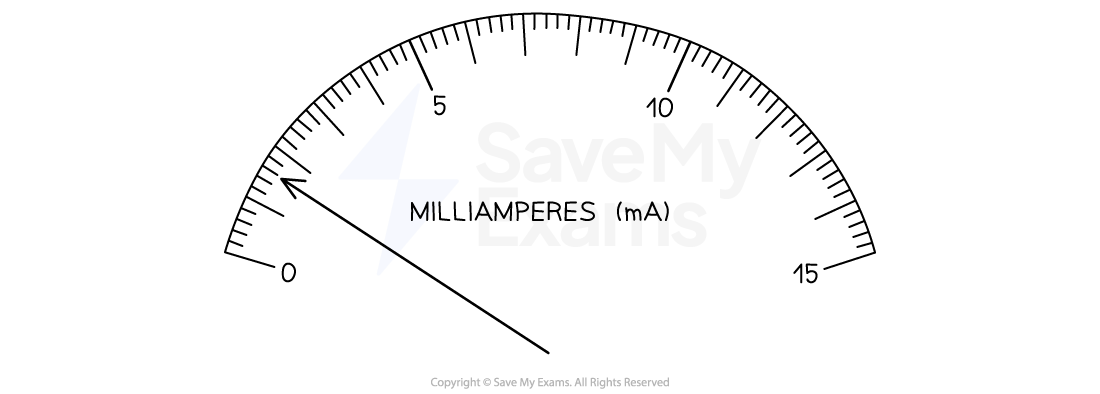
The uncertainties in this reading are:
Absolute
Uncertainty =
= 0.1 mA
Reading = 1.6 ± 0.1 mA
Fractional
Uncertainty =
Reading = 1.6 mA ±
Percentage
Uncertainty =
Reading = 1.6 mA ± 6%
Propagating uncertainties in processed data
Uncertainty propagates in different ways depending on the type of calculation involved
When combining uncertainties, the rules are as follows:
Operation | Example | Propagation Rule |
|---|---|---|
Addition & Subtraction | The sum of the absolute uncertainties | |
Multiplication & Division |
| The sum of the fractional uncertainties |
Power | The magnitude of n times the fractional uncertainty |
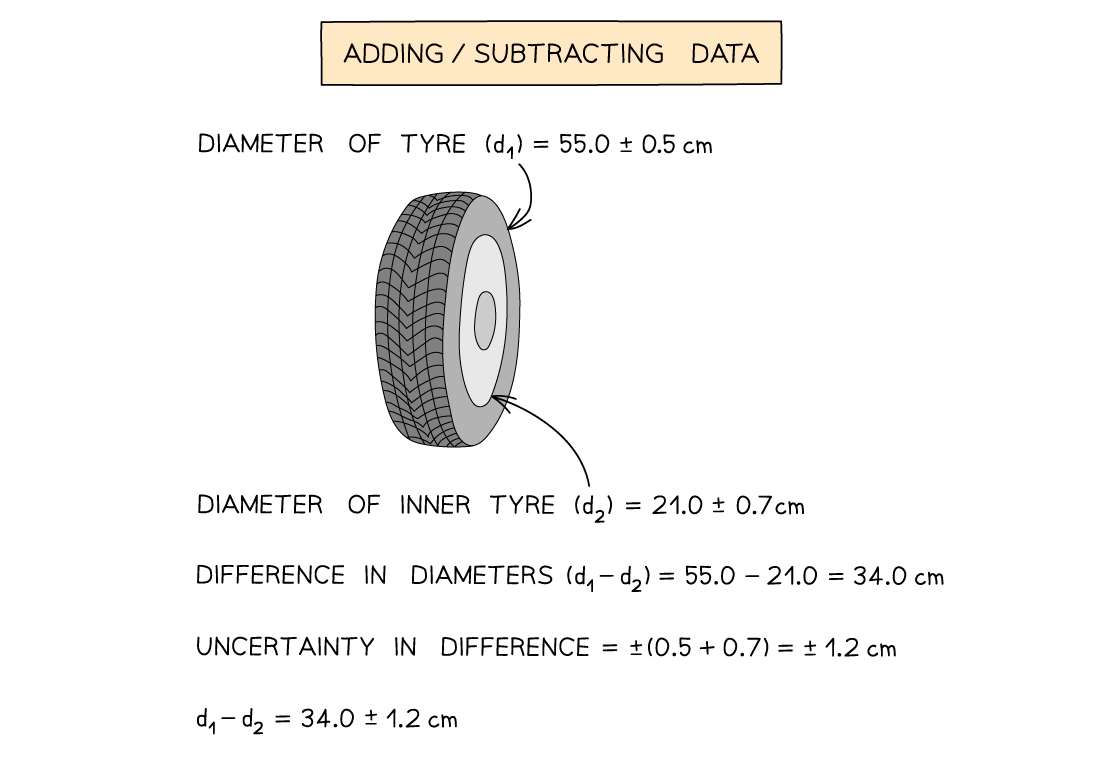
Adding or subtracting measurements
Add together the absolute uncertainties
Multiplying or dividing measurements
Add the percentage or fractional uncertainties

Measurements raised to a power
Multiply the percentage uncertainty by the power

Examiner Tips and Tricks
Remember:
Absolute uncertainties (denoted by Δ) have the same units as the quantity
Percentage uncertainties have no units
The uncertainty in constants, such as π, is taken to be zero
Uncertainties in trigonometric and logarithmic functions will not be tested in the exam, so just remember these rules and you’ll be fine!

Unlock more, it's free!
Did this page help you?