Revision NotesExam QuestionsFlashcards
IBPhysicsHLRevision NotesThe Particulate Nature of MatterGreenhouse EffectEnergy Balance Problems

Syllabus Edition

First teaching 2023

First exams 2025

|

Energy Balance Problems (HL IB Physics)

Revision Note

Katie M

Author

Katie M

Last updated

Energy Balance Problems

  • It is useful to consider Earth’s energy balance in terms of how much incoming energy from the Sun is used and how much is returned to space
  • If incoming and outgoing energy are in balance, the Earth’s temperature will remain constant
  • This can be used to create models which can help climate scientists predict temperature fluctuations based on current and increased concentrations of greenhouse gases
    • At it’s simplest, the model involves a one-layer atmosphere above the Earth’s surface

Worked example

The diagram below shows a simple energy balance climate model in which the atmosphere and the Earth’s surface are treated as two bodies.

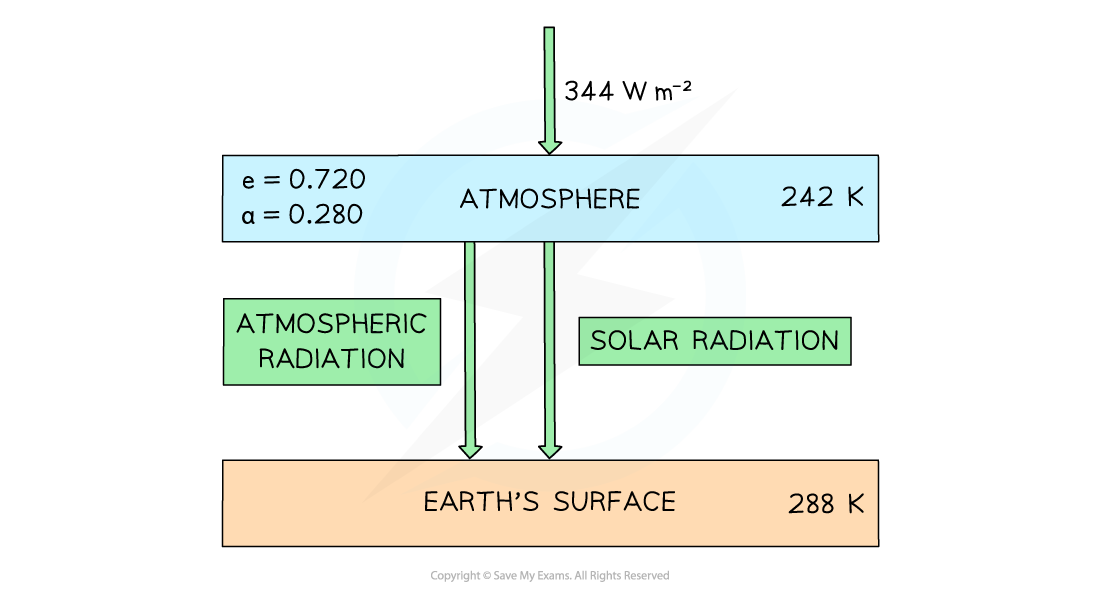
8-2-5-we-energy-system_sl-physics-rn

The Earth’s surface receives both solar radiation and radiation emitted from the atmosphere.

At current atmospheric greenhouse gas concentrations, the temperature of Earth’s atmosphere is set to increase by 6 K.

Data for this model:

  • Current mean temperature of the Earth’s atmosphere = 242 K
  • Current mean temperature of the Earth’s surface = 288 K
  • Solar intensity per unit area at top of the atmosphere = 344 W m–2
  • Emissivity of the atmosphere, e = 0.720
  • Albedo of the atmosphere, a = 0.280

Use this data to estimate the increase in temperature of the Earth’s surface.

Answer:

Step 1: List the known quantities

  • Solar intensity above atmosphere, Ia = 344 W m–2
  • Emissivity of the atmosphere, e = 0.720
  • Emissivity of the surface, e = 1
  • New temperature of Earth’s atmosphere, Ta = 242 + 6 = 248 K
  • Stefan-Boltzmann constant, σ = 5.67 × 10–8 W m–2 K–4
  • Intensity absorbed at the Earth’s surface = Is
  • New temperature of Earth’s surface = Ts

Step 2: Calculate the solar intensity absorbed at the Earth’s surface

  • This can be calculated using the emissivity and the solar intensity above the atmosphere

Is = e × Ia

Is = 0.720 × 344 = 247.68 = 248 W m–2

Step 3: Write the equation for the power per unit area emitted by a body

  • Since intensity = power per unit area

I = eσT4

Step 4: Calculate the new intensity radiated by the atmosphere

I = 0.720 × (5.67 × 10–8) × 2484 = 154.43 = 154 W m–2

Step 5: Calculate the new intensity absorbed by the Earth’s surface

  • The intensity absorbed by the Earth’s surface is a sum of the solar radiation that reaches the surface plus the intensity radiated by the atmosphere

New intensity, Is = 248 + 154 = 402 W m–2

Step 6: Calculate the new temperature of the Earth’s surface

  • The Earth’s surface can be assumed to be a black body, hence e = 1

Is = σTs4

400 = (5.67 × 10–8) × Ts4

Ts = fourth root of fraction numerator 400 over denominator 5.67 cross times 10 to the power of negative 8 end exponent end fraction end root = 290 K

Step 7: Determine the increase in temperature

ΔT = 290 – 288 = 2 K

Examiner Tip

In simplified climate models, you can generally assume the Earth’s surface and the atmosphere:

  • Act as black bodies - this means the emissivity of the surface will be equal to 1!
  • Remain at a constant temperature

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:The Greenhouse EffectNext:Gas Pressure
    Katie M

    Author: Katie M

    Expertise: Physics

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.