Revision NotesExam QuestionsFlashcards
IBPhysicsHLRevision NotesSpace, Time & MotionRigid Body MechanicsAngular Momentum (HL)

Syllabus Edition

First teaching 2023

First exams 2025

|

Angular Momentum (HL) (HL IB Physics)

Revision Note

Katie M

Author

Katie M

Last updated

Angular Momentum

  • Angular momentum is the rotational equivalent of linear momentum, which is defined by mass × velocity, or

space p space equals space m v

  • Therefore, angular momentum L is defined by

L space equals space I omega

  • Where:
    • L = angular momentum (kg m2 rad s−1)
    • I = moment of inertia (kg m2)
    • omega = angular velocity (rad s−1)

Angular Momentum of a Point Mass

  • The moment of inertia of a rotating point mass m which is a distance r from an axis of rotation is equal to

I space equals space m r squared

  • The angular velocity of the point mass is given by

omega space equals space v over r

  • Therefore, the angular momentum of the point mass is equal to

L space equals space I omega space equals space open parentheses m r squared close parentheses cross times v over r space equals space m v r

Worked example

A horizontal rigid bar is pivoted at its centre so that it is free to rotate. A point particle of mass 3M is attached at one end of the bar and a container is attached at the other end, both are at a distance of R from the central pivot. 

A point particle of mass M moves with velocity v at right angles to the rod as shown in the diagram.

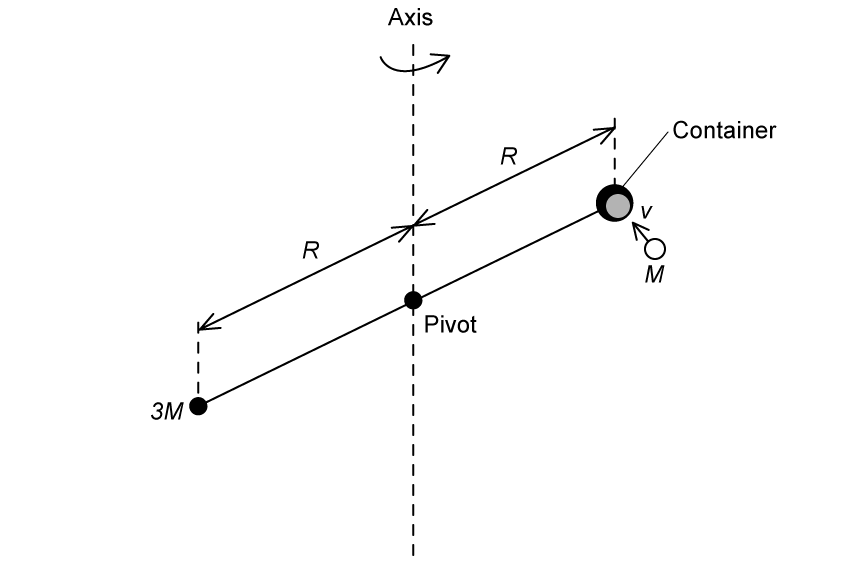
1-4-7-angular-momentum-of-a-system-worked-example-ib-2025-physics

The particle collides with the container and stays within it as the system starts to rotate about the vertical axis with angular velocity ω.

The mass of the rod and the container are negligible.

Write an expression for the angular momentum of the system about the vertical axis:

(a)
just before the collision, in terms of M, v and R
(b)
just after the collision, in terms of M, R and ω.
 

Answer:

(a) Just before the collision:

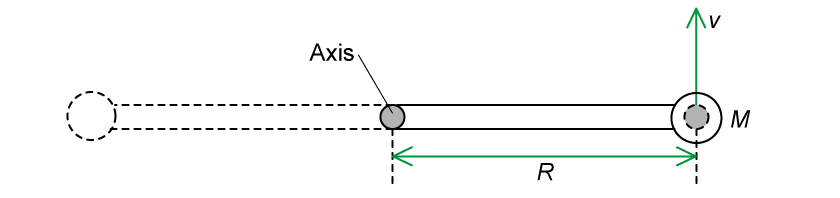
1-4-7-angular-momentum-of-a-system-worked-example-ma1-ib-2025-physics

  • Angular momentum is equal to:

L space equals space I omega

  • The moment of inertia of a point particle is

I space equals space m r squared

  • Linear velocity is related to angular velocity by

v space equals space omega r

  • The rod, container and 3M mass are all stationary before the collision, so we only need to consider the angular momentum of the point particle
  • Where:
    • Mass of the particle, m space equals space M
    • Distance of the particle from the axis, r space equals space R
    • Angular velocity of the particle, omega space equals space v over R
  • Therefore, the angular momentum of the system before the collision is:

L space equals space open parentheses M R squared close parentheses cross times v over R space equals space M v R

(b) After the collision:

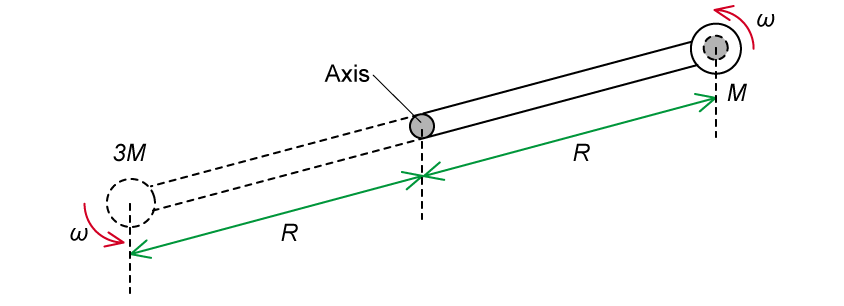
1-4-7-angular-momentum-of-a-system-worked-example-ma2-ib-2025-physics

  • The whole system rotates with an angular velocity of ω
  • We are considering the rod and the container as massless, so we only need to consider the angular momentum of the two masses M and 3M
  • Therefore, the angular momentum of the system after the collision is:

L space equals space open parentheses M R squared close parentheses omega space plus space open parentheses 3 M R squared close parentheses omega

L space equals space 4 M R squared omega

Examiner Tip

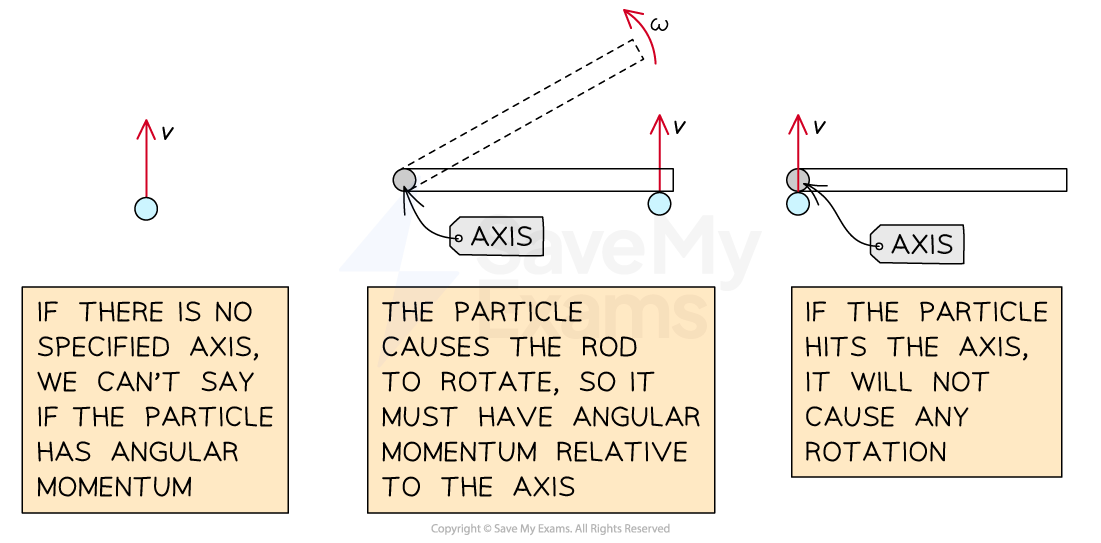
You should know that objects travelling in straight lines can have angular momentum - just make sure you understand that it all depends on the position of the object in relation to the axis of rotation being considered

1-4-7-angular-momentum-particle-in-a-straight-line-ib-2025-physics

Conservation of Angular Momentum

  • As with linear momentum, angular momentum is always conserved 
  • The principle of conservation of angular momentum states:

The angular momentum of a system always remains constant, unless a net torque is acting on the system

  • This conservation law has many real-world applications, for example
    • A person on a spinning chair spins faster while their arms and legs are contracted and slower while extended
    • Objects in elliptical orbits travel faster nearer the object they orbit and slower when further away
    • Ice skaters can change their rotation velocity by extending or contracting their arms
    • Tornados spin faster as their radius decreases

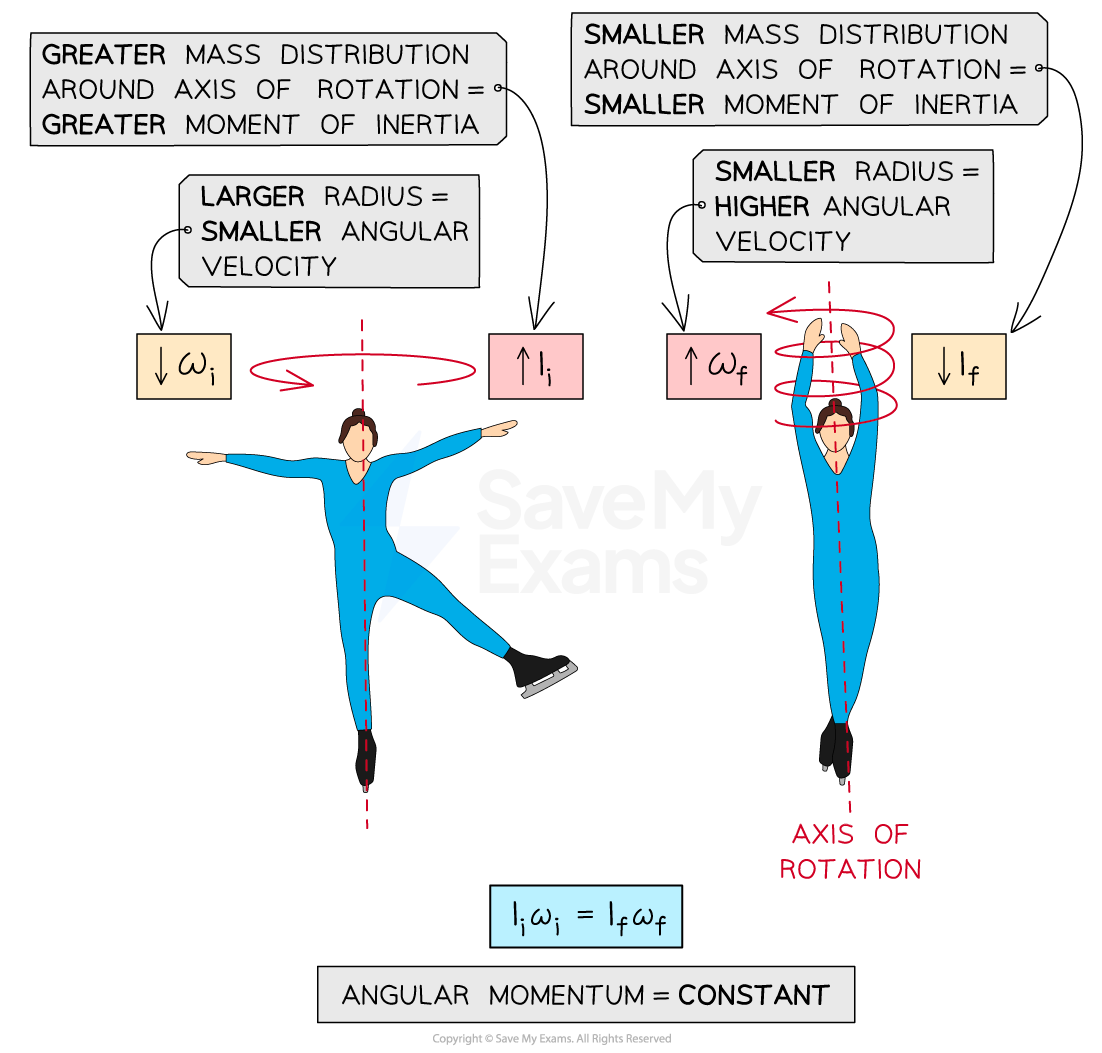
1-4-7-conservation-of-angular-momentum-ice-skater

Ice skaters can change their moment of inertia by extending or contracting their arms and legs. Due to conservation of angular momentum, this allows them to spin faster or slower

  • Problems involving a constant angular momentum can be solved using the equation:

I subscript i omega subscript i space equals space I subscript f omega subscript f

  • Where:
    • I subscript i = initial moment of inertia (kg m2)
    • omega subscript i = initial angular velocity (rad s−1)
    • I subscript f = final moment of inertia (kg m2)
    • omega subscript f = final angular velocity (rad s−1)

Worked example

The diagram shows the different positions of a diver between jumping off a springboard and entering the water.

During their fall, the diver pulls their arms and legs into a tight tuck position while in the air and straightens them before entering the water.

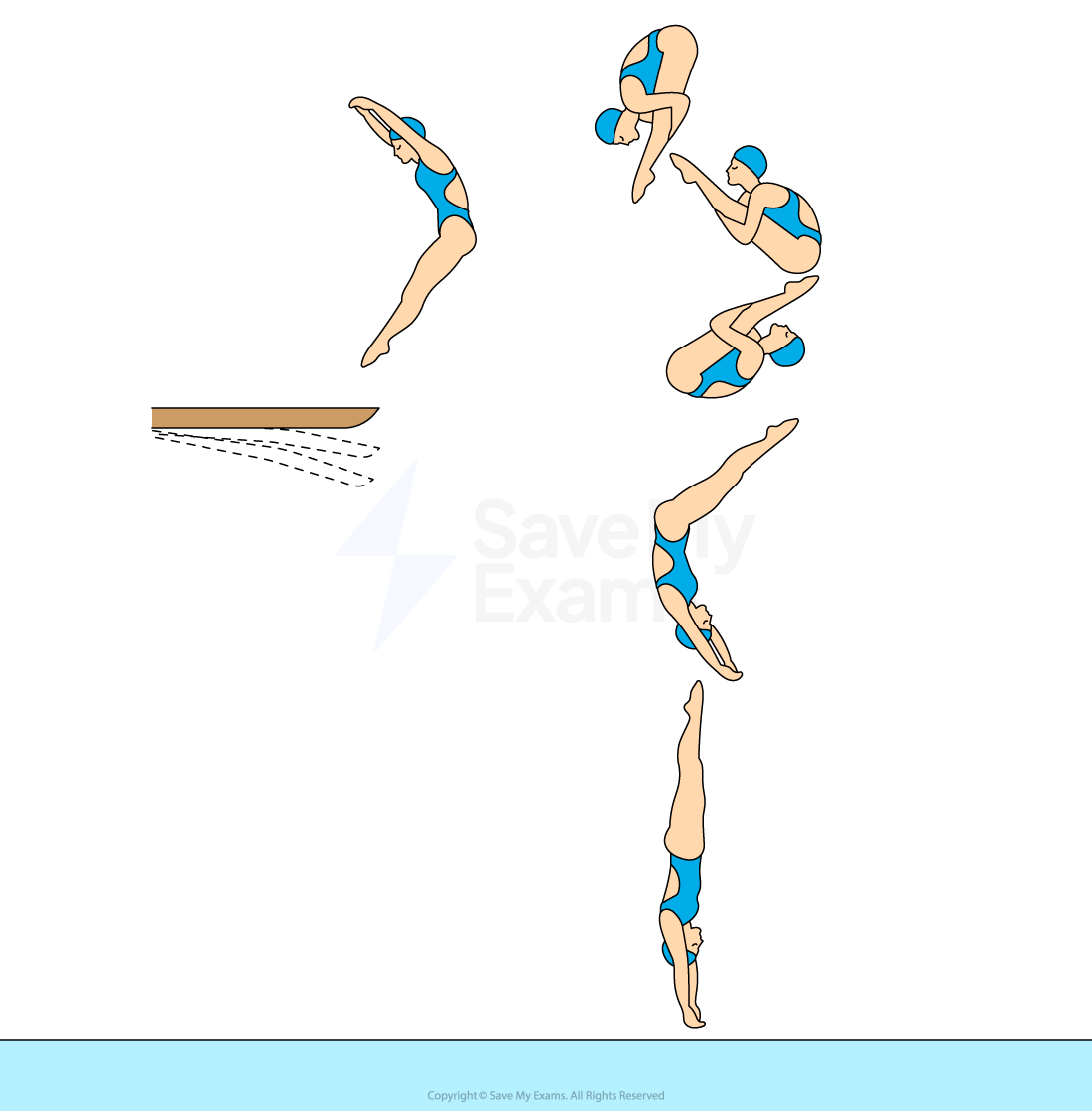
8YT3mwKp_1-4-7-conservation-of-angular-momentum-diver-mcq-worked-example

Which row correctly describes the changes to the diver's moment of inertia and angular velocity as they bring their limbs closer to their body?

  moment of inertia angular velocity
A. increases increases
B. decreases increases
C. increases decreases
D. decreases decreases

Answer:  B

  • After the diver leaves the springboard, there is no longer a resultant torque acting on them
    • This means their angular momentum remains constant throughout the dive
  • Due to the conservation of angular momentum:

I subscript i omega subscript i space equals space I subscript f omega subscript f space equals space c o n s t a n t

  • When the diver tucks their arms and legs in closer to their body, they decrease their moment of inertia
    • This eliminates options A & C
  • Therefore, to conserve angular momentum, when the diver's moment of inertia decreases, their angular velocity must increase

Worked example

A spherical star of mass M and radius R rotates about its axis. The star explodes, ejecting mass in space radially and symmetrically. The remaining star is left with a mass of 1 over 10 M and a radius of 1 over 50 R.

Calculate the ratio of the star’s final angular velocity to its initial angular velocity.

The moment of inertia of a sphere is 2 over 5 M R squared

Answer:

  • Before the star explodes:
    • Initial moment of inertia, I subscript i space equals space 2 over 5 M R squared
    • Initial angular velocity = omega subscript i
  • After the star explodes:
    • Final moment of inertia, I subscript f space equals space 2 over 5 open parentheses 1 over 10 M close parentheses open parentheses 1 over 50 R close parentheses squared
    • Final angular velocity = omega subscript f
  • From the conservation of angular momentum:

I subscript i omega subscript i space equals space I subscript f omega subscript f

open parentheses 2 over 5 M R squared close parentheses omega subscript i space equals space open parentheses 2 over 5 cross times 1 over 10 M cross times open parentheses 1 over 50 close parentheses squared R squared close parentheses omega subscript f

open parentheses up diagonal strike 2 over 5 M R squared end strike close parentheses space omega subscript i space equals space open parentheses up diagonal strike 2 over 5 M R squared end strike close parentheses space fraction numerator 1 over denominator 25 space 000 end fraction omega subscript f

  • Therefore, the ratio of the star’s final angular velocity to its initial angular velocity is:

omega subscript f over omega subscript i space equals space 25 space 000

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:Newton’s Second Law for Rotation (HL)Next:Angular Impulse (HL)
    Katie M

    Author: Katie M

    Expertise: Physics

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.