Space-Time Diagrams (HL) | HL IB Physics Revision Notes 2025

Space-Time Diagrams

Spacetime (or Minkowski) diagrams represent an object's motion in spacetime
They help to visualise
- Time dilation
- Length contraction
- Simultaneity

Since 4D (x, y, z, t) diagrams cannot be drawn on a 2D page, we collapse 3D space (x, y, z) into 1 spacial dimension and keep time as its own dimension
This gives a spacetime diagram
- Lines drawn on a spacetime diagram are called world lines
Instead of the usual distance-time graphs, we plot
- The horiztonal axis as x
- The vertical axis as ct

Objects moving slower are represented by a steeper gradient on a spacetime gradient

Note that both axes have dimensions of length
- This makes it easy to compare values on one axis and another
This means the worldlines have a gradient of
- $\frac{c ∆ t}{∆ x} = \frac{c}{v}$ where the velocity is $v = \frac{∆ x}{∆ t}$
This means:
- The steeper the gradient, the slower the object is moving
- The shallower the gradient, the faster the object is moving
ct was first seen when the spacetime interval was introduced, and c is chosen deliberately so our diagram is oriented around the speed of light
- ct is a sort of 'distance in time'

Worldline at Rest

1-5-10-space-time-at-rest-ib-2025-physics

A worldline for an object at rest

P is an object at rest
- It has an infinite slope meaning v = 0
It is only moving in time, not in space

Worldline at Constant Velocity

1-5-10-space-time-at-constant-velocity-ib-2025-physics

A worldline at constant velocity has to have a gradient steeper than 1

Q is an object at constant velocity
The object moves some distance over some time
For an object moving at the speed of light, c (e.g. a massless photon), its worldline has a gradient of $\frac{c}{v} = \frac{c}{c} =$ 1 (a 45^o angle)
Since nothing can travel faster than the speed of light (i.e. v cannot be greater than c), then objects can only have a gradient greater than 1
- Therefore, a gradient of 1 is the lowest possible gradient
Q's motion does not need to start at the origin. As long as it has a gradient of less than 1, it can start anywhere on the x-axis

Worldline accelerating

1-5-10-space-time-accelerating

All points on a worldline representing an object accelerating must have a gradient steeper than 1

R is an object with a varying velocity that represents possible motion
- It has a small gradient (larger velocity), which increases (decreasing velocity)
- Its gradient never gets less than 1
S is an object with a varying velocity that represents impossible motion
- At one point, it has a gradient of less than 1 implying a velocity greater than c
- Even though it does not physically cross the v = c gradient line, it is still not possible because a portion of the line has a gradient of less than one

Multiple Reference Frames

Every point on a spacetime diagram represents an event, for example, an object moving
More than one inertial reference frame can be represented on a spacetime diagram
- ct and x represent the co-ordinate axes for an observer in frame S
- ct' and x' represent the co-ordinate axes for an observer in frame S' (moving at speed v with respect to frame S)
Therefore, we can combine two separate spacetime diagrams for different inertial reference frames moving at constant speed relative to each other
- The axes for the ct' and x' are at an angle
The worldline T shows the equivalent worldline of P, but now in the S' reference frame
- This represents an object at rest in its own co-ordinate system

Worldline of a particle at rest in the reference frame S'

The axes are tilted because the ct' reference frame is travelling at speed v relative to the ct reference frame
- The x' axis must also be tilted in order for the speed of light (the dashed line in the middle) to be the same in both reference frames
The scales on the time axes ct and ct′ and on the space axes x and x′ of two inertial reference frames moving relative to one another are not the same and are defined by lines of constant spacetime interval
If an event occurs (such as a flash of light), both reference frames will measure a different time and different position with respect to each other
- This can be seen on a spacetime diagram

1-5-10-moving-reference-frame-event

An event is shown in reference frames S and S' with differing values of distance and time

The event in the S reference frame occurs at (X, cT)
The event in the S' reference frame occurs at (X', cT')
The co-ordinates in the S' reference frame are determined by lines 1 and 2
- Line 1 is a line parallel to the x' axis
- Line 2 is a line parallel to the ct' axis
The clocks in both frames show zero at the origins where two frames collide i.e. both observers start their clocks at the same time to measure any time intervals

Simultaneity

We can now see that simultaneous events in one frame are not simultaneous in another moving inertial reference frame
Let's go back to Observers A and B in Simultaneity in Special Relativity
- We can see that Observer B sees the light reach points X and Y at the same time, whilst Observer A (in the ct'–x' co-ordinate system) sees the light from the lamp reach point X before point Y on a spacetime diagram

1-5-10-simultaneity-space-time-diagrams

Simultaneity is not possible for two reference frames moving relative to each other

Time Dilation

Consider two flashes of light at x = 0 in the S reference frame that occur one after another
When these flashes are observed in the S' frame, we can see the time between the flashes is longer
- The time between them has increased (dilated)

1-5-10-time-dilation-space-time-diagrams

Spacetime diagrams representing time dilation

Another difference is that in the S' reference frame, the first flash now occurs on the -x axis
- This just means it takes place to the left of the observer

Length Contraction

Consider a rod measured in the S reference frame where the rod moving relative to S
The rod has the same speed as it does in the S' reference frame (which is also moving relative to S)

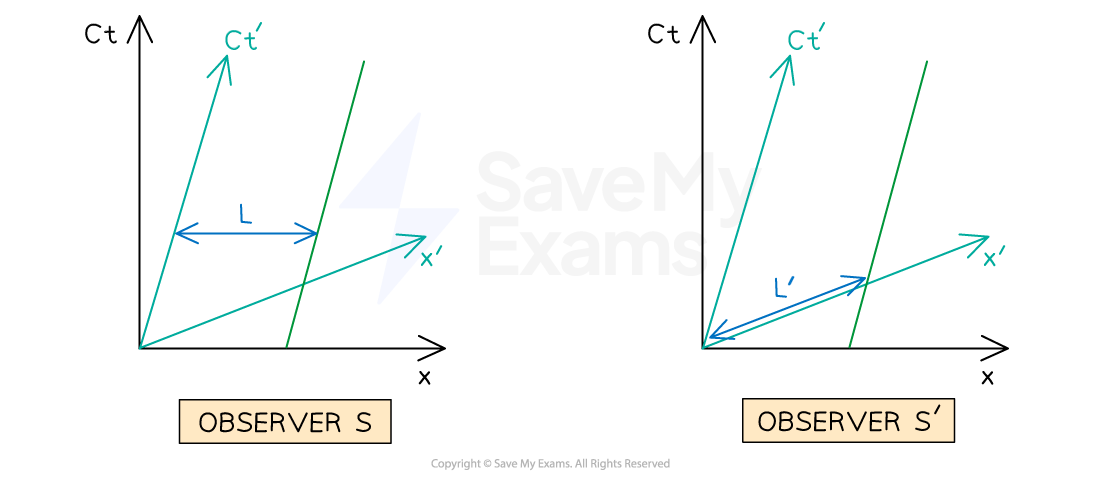
1-5-10-length-contraction-space-time-diagrams-ib-2025-physics

Spacetime diagrams representing length contraction

The length of the rod is measured by measuring each side at the same time
- The observer in frame S will measure a length L
When the observer in frame S' measures the rod, they see the rod as stationary (as it is moving at the same speed as the observer)
- The observer in frame S will measure a length L'
L is shorter than L', which means that the length has been shortened (contracted) when measured by Observer S, who is moving relative to the rod
- Although it is the rod that is moving, remember, it is at rest in its own reference frame and Observer S is moving relative to it
This occurs from the fact that measurements that are simultaneous in one reference frame are not simultaneous in another

Worked example

The spacetime diagram shows the axes of an inertial reference frame S and the axes of a second inertial reference frame S′ that moves relative to S with speed 0.6432c. When clocks in both frames show zero the origins of the two frames coincide.

1-5-10-spacetime-we

Event E has co-ordinates x = 1.5 m and ct = 0 in frame S.

(a)

Label, on the diagram,

(i) the space co-ordinate of event E in the S′ frame. Label this event with the letter Q.

(ii) the event that has co-ordinates x′ = 1.5 m and ct′ = 0. Label this event with the letter R.

(b)

A rod at rest in frame S has a proper length of 1.5 m. At t = 0, the left-hand end of the rod is at x = 0 and the right-hand end is at x = 1.5 m.

Using the spacetime diagram, outline without calculation, why observers in frame S′ measure the length of the rod to be less than 1.5 m.

Answer:

(a)

(i) Draw a line parallel to the ct' axis

(ii)

Step 1: List the known quantities

Speed of the spacecraft, v = 0.6432c
Position of event in frame S, x = 1.5 m

Step 1: Calculate the x' co-ordinate of point Q

To convert between a position (or time) from one co-ordinate system and another, we can use Lorentz transformations

$x' = γ (x - v t)$

$x' = \frac{1}{\sqrt{1 - \frac{{(0.6432 c)}^{2}}{c^{2}}}} (1.5 - 0) = 1.959 = 2.0 m$

Since there are no other objects involved, speed v = 0

Step 2: Label this point on the axes as R

The co-ordinates are x′ = 1.5 m and ct′ = 0
Point R (at 1.5 m) is roughly $\frac{2}{3}$ of the distance of Q (at 2.0 m)

1-5-10-spacetime-ms-2-ib-2025-physics

(b)

Step 1: Outline why observers in frame S′ measure the length of the rod to be less than 1.5 m

The ends of the rod must be recorded at the same time in frame S'
This is shown on the spacetime diagram:

1-5-10-spacetime-ms-3

The right-hand side of the rod intersects the x' axis at a co-ordinate that is less than 1.5 m

1-5-10-spacetime-ms-4-ib-2025-physics

Examiner Tip

This all might sound counter-intuitive because we're used to thinking of position versus time with distance-time graphs, rather than time versus position. Remember, now the gradient is $\frac{1}{v e l o c i t y}$ instead of equating to the velocity.

The important thing about worldlines is not their value but their gradient. Where they start doesn't matter, whether at the origin or along the x axis, their gradients cannot be less than 1.

Make sure you never write c', as there is no such thing. c is the same in all reference frames.

Notice that reading from the ct' and x' co-ordinate axis is actually no different reading from ct and x, it's just that they're slanted so it looks a bit different, but the principles are still the same.

Exam questions will generally have the units of ct and x in light years (ly), so make sure you're comfortable with this definition.

Velocity on a Space-Time Diagram

The worldline for a moving particle on a spacetime diagram using the x-ct axis is a diagonal line

1-5-11-spacetime-velocity

The velocity of the particle can be calculated by the angle of the moving particle's worldline with the ct axis
When we are using the x-ct axis, we can see that:

$\tan θ = \frac{o p p o s i t e}{a d j a c e n t} = \frac{∆ x}{c ∆ t}$

From mechanics, we know that velocity is the rate of change of displacement:

$\frac{∆ x}{∆ t} = v$

Therefore:

$\tan θ = \frac{v}{c}$

Where:
- θ = angle between the world line and the ct axis (°)
- v = velocity of the object (m s^–1)
- c = speed of light

Worked example

A spaceship travels away from Earth in the direction of a nearby planet. A spacetime diagram for the Earth's reference frame shows the worldline of the spaceship. Assume the clock on the Earth, the clock on the planet, and the clock on the spaceship were all synchronized when ct = 0.

1-5-11-spacetime-velocity-we-ib-2025-physics

Show, using the spacetime diagram, that the speed of the spaceship relative to the Earth is 0.80c.

Answer:

Method 1: Using the gradient

Step 1: Choose a co-ordinate pair

Choose any corresponding value of ct and x e.g. ct = 10, x = 8

Step 2: Calculate the gradient

The gradient of a spacetime graph is $\frac{c}{v}$

$g r a d i e n t = \frac{c}{v} = \frac{10}{8}$

Step 3: Calculate the velocity, v

$v = \frac{8}{10} c = 0.8 c$

Method 2: Measure the angle

Step 1: Measure angle θ using a protractor

1-5-11-spacetime-velocity-ms-2

θ = 39°

Step 2: Substitute into the velocity equation

$\tan θ = \tan (39) = 0.8 = \frac{v}{c}$

$v = 0.8 c$

Examiner Tip

Any discussion of world lines of moving particles will only be limited to constant velocity in your exam.

Make sure you have your protractor with you in your exam. An exam question could ask you to measure the angle θ yourself from the diagram on your exam paper.

Space-Time Diagrams (HL) (HL IB Physics)

Revision Note