The Bohr Model of Hydrogen (HL) (HL IB Physics)

• Hydrogen is the simplest atom in existence, making it ideal for experiments investigating the nature of electron energy levels
• Line spectra produced by hydrogen atoms showed that
  
  • Electrons are able to jump, or transition, between specific energy levels producing specific energy photons
  • Different transitions can be categorised into series, or families, of lines

• The Lyman series converges on the ground state (n = 1) for electrons
  • The Balmer series converges on the second energy level (n = 2)
  • The Ritz-Paschen converges on the third energy level (n = 3) and so on

The transitions observed in hydrogen line spectra can be classed into series

• The Lyman series photons will have the highest energies since they have the shortest wavelength
  • These transitions tend to produce ultraviolet photons

• The Pfund series photons will have the lowest energies since they have the longest wavelength
  • These transitions tend to produce infrared photons

Representing the electron jumps in the hydrogen spectra

• The finding of these electron transitions helped scientists to understand how electrons work to produce photons of specific wavelength and energy
• This led to the development of the Bohr model of hydrogen, which states that
  • Electrons can only move in fixed orbits
  • The orbital radius of electrons is restricted to certain values

• The discrete energy of the transitions in the Bohr model for hydrogen are described by the equation:

• Where
  • E = photon energy (J)
  • n = an integer 1, 2, 3 etc. to describe the energy level of an atom

• Angular momentum is a property of any spinning or rotating body, very similar to linear momentum
  • In linear motion, momentum is the product of mass and velocity
  • In rotational motion, the momentum is the product of moment of inertia and angular speed

• Angular momentum is a vector, which means:
  • The magnitude is equal to the momentum of the particle times its radial distance from the centre of its circular orbit
  • The direction of the angular momentum vector is normal to the plane of its orbit with the direction being given by the corkscrew rule

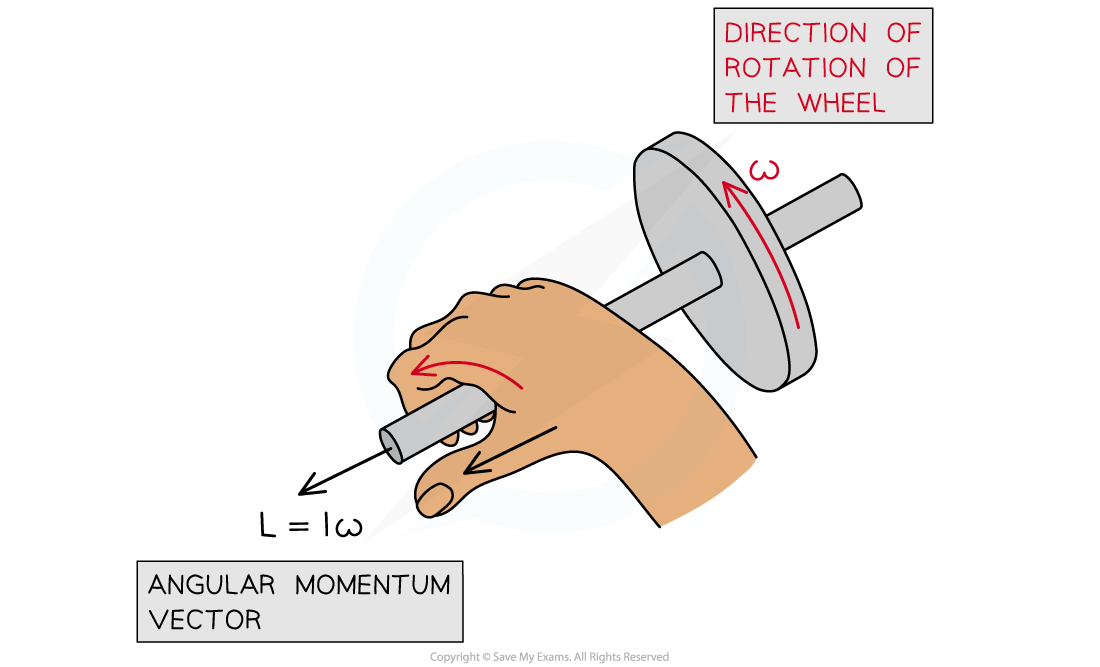
Angular momentum acts at right angles to the direction of rotation

• Niels Bohr proposed that the angular momentum L of an electron in an energy level is quantised in integer multiples of Planck's constant over 2π:

• Where:
  • n = an integer (n = 1, 2, 3...)
  • h = Planck’s constant

• Hence the angular momentum for an electron in a circular orbit is constant

• De Broglie proposed that an electron with momentum p = mv has a wavelength λ given by

• For an electron moving in a straight line, the matter wave takes a familiar wave shape consisting of peaks and troughs
  • Although the electron itself isn't oscillating up and down, only the matter wave is

de Broglie matter wave for an electron moving in a straight line at constant speed

• For the same electron moving in a circle, the matter wave still has a sinusoidal shape but is wrapped into a circle

de Broglie matter wave for an electron moving in a circular orbit at constant speed

• As the electron continues to orbit in a circle two possibilities may occur:

 1. On completing one oscillation, the waves overlap in phase

• • The waves will continue in phase over many orbits giving rise to constructive interference and a standing wave 

de Broglie matter wave where n = 3. Here the circumference of the circular orbit is 3λ

• • In other words, peak overlaps with peak, trough with trough
  • This means that where the waves overlap, destructive interference occurs and as a result, no such electron orbit is allowed

de Broglie matter wave where 3λ is less than the orbit's circumference

• Hence, the circumference of the orbit  must equal an integer number of wavelengths  for a standing wave to form:

• Using the de Broglie relation:

• Since momentum is equal to :

• Rearranging for angular momentum :

• This is known as the Bohr Condition 
• Where:
  • n = integer number of energy level
  • h = Planck's constant (J s)
  • m = mass of an electron (kg)
  • v = velocity of electron (m s^–1)
  • r = radius of orbit (m)