Energy Released in Fusion Reactions

When two small nuclei undergo a fusion reaction, the single larger nucleus produced as a result will have a higher binding energy per nucleon than the original two nuclei
As a result of the mass defect between the parent nuclei and the daughter nucleus, energy is released

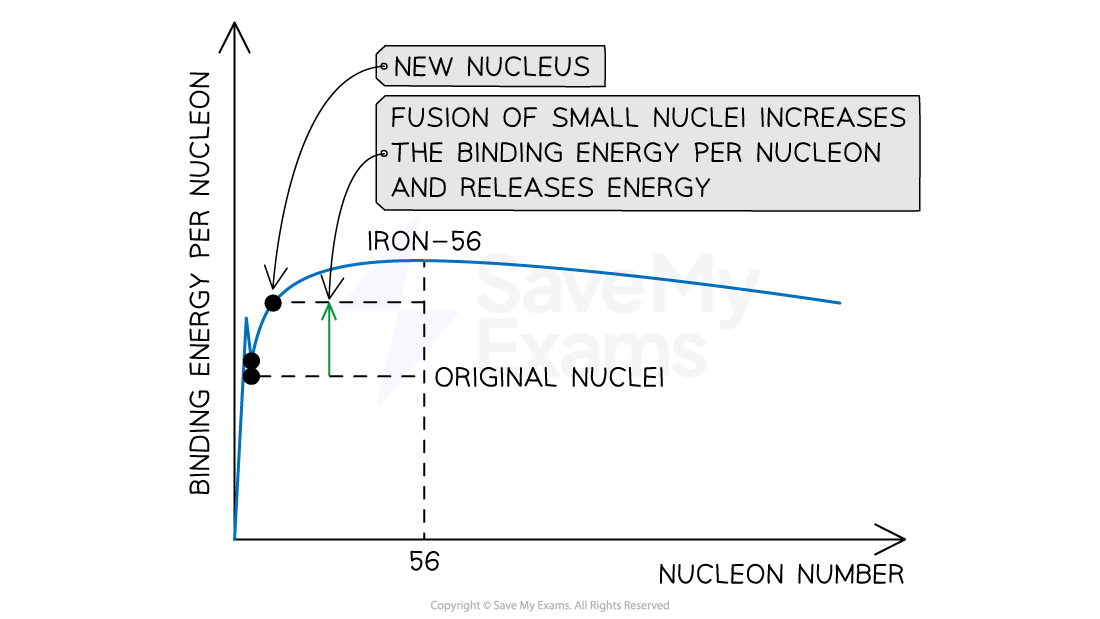
5-5-2-energy-released-in-fusion-reactions-graph

The energy released from fusion reactions is due to the mass defect between parent and daughter nuclei

When two protons fuse, the element deuterium is produced
In the centre of stars, the deuterium combines with a tritium nucleus to form a helium nucleus, plus the release of energy, which provides fuel for the star to continue burning

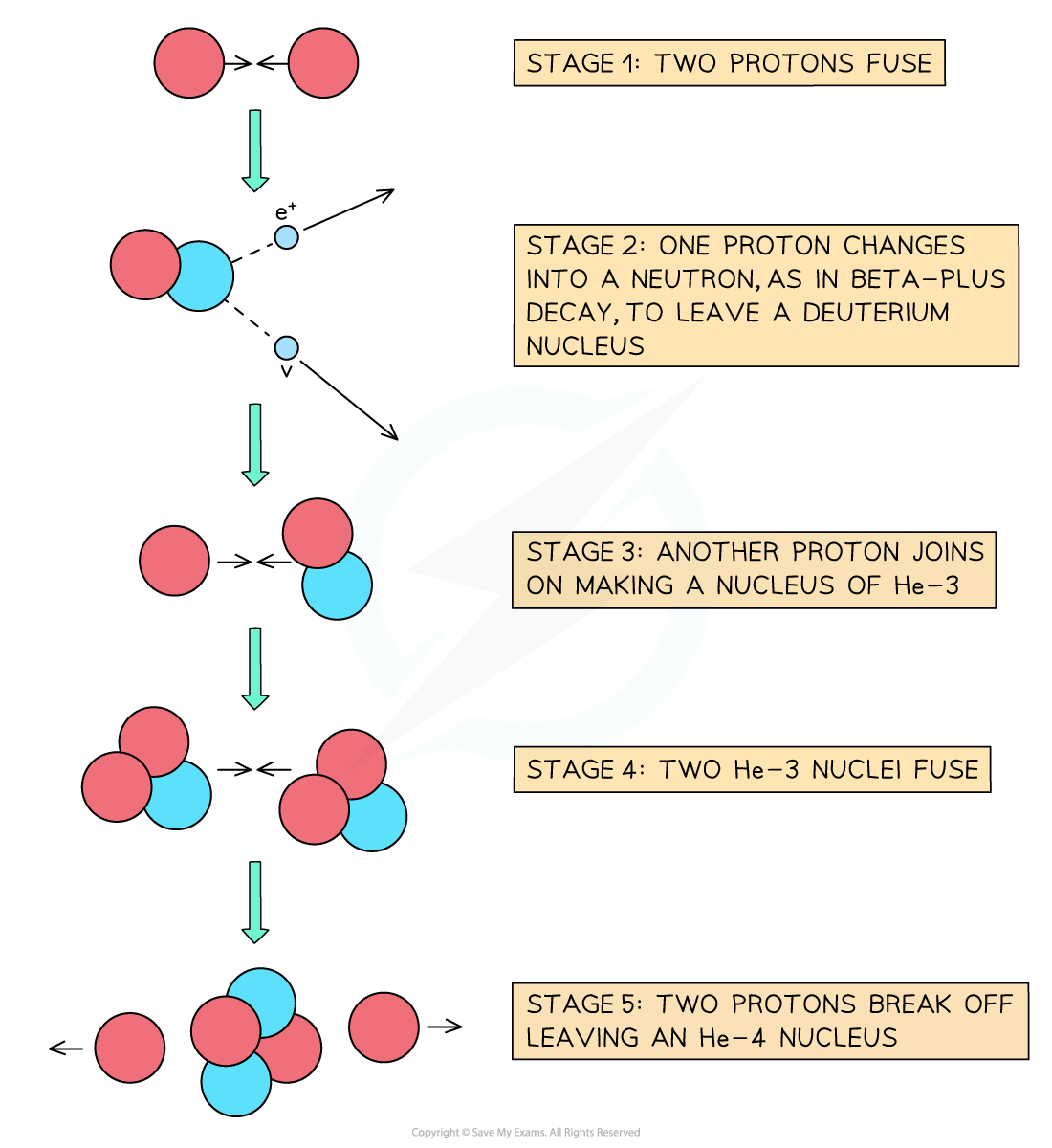
11-4-nuclear-fusion-and-binding-energy_edexcel-al-physics-rn

The five-stage process of fusion in stars

Worked example

In the Sun, fusion occurs via a process known as the proton-proton cycle.

It is predicted that 80% of the total power output of the Sun is produced through the following cycle:

$\begin{array}{r} {}_{1}^{1}H + {}_{1}^{1}H \to {}_{1}^{2}H + {}_{1}^{0}e^{+} + ν_{e} \\ {}_{1}^{1}H + {}_{1}^{2}H \to {}_{1}^{2}{He} + γ \\ {}_{2}^{3}{He} + {}_{2}^{3}{He} \to {}_{2}^{4}{He} + {}_{1}^{1}H + {}_{1}^{1}H \end{array}\} 4 {}_{1}^{1}H \to {}_{2}^{4}{He} + 2 {}_{1}^{0}e^{+} + 2 ν_{e}$ (overall reaction)

nucleus	rest mass / u
hydrogen-1	1.007825
helium-4	4.002603

The neutrinos produced in the first step carry away 2% of the energy released by the process.

Determine the mass of hydrogen-1 that must be fused each second to produce this output.

Luminosity of the Sun = 3.85 × 10²⁶ W.

Answer:

Step 1: Determine the energy released per overall fusion reaction

In the overall reaction, 4 hydrogen-1 nuclei fuse into a helium-4 nucleus, so the mass defect is

mass defect: Δm = 4(1.007825u) − 4.002603u = 0.028697u

Where atomic mass unit, u = 1.66 × 10⁻²⁷ kg
Using mass-energy equivalence, the energy released by one reaction is

ΔE = Δmc² = 0.028697 × (1.66 × 10⁻²⁷) × (3 × 10⁸)²

energy released: ΔE = 4.287 × 10⁻¹² J

Step 2: Determine the energy released minus the energy that is carried away by neutrinos

Per reaction, neutrinos carry away 2% of 4.287 × 10⁻¹² J, so 98% of the energy contributes to the luminosity of the Sun

energy released: ΔE = 0.98 × (4.287 × 10⁻¹²) = 4.201 × 10⁻¹² J

Step 3: Determine the number of fusion reactions that happen each second

This process accounts for 80% of the luminosity of the Sun,
So, the total power output of the reaction = 0.8 × (3.85 × 10²⁶) W

The number of fusion reactions each second is:

number of reactions = $\frac{p o w e r o u t p u t}{e n e r g y r e l e a s e d p e r r e a c t i o n} = \frac{0.8 \times (3.85 \times 10^{26})}{4.201 \times 10^{- 12}}$

number of reactions = 7.332 × 10³⁷ s⁻¹

Step 4: Determine the mass of hydrogen that fuses each second

Every reaction fuses 4 hydrogen-1 nuclei, so the mass per reaction is 4 × 1.007825u
The mass of hydrogen-1 that fuses each second in this process is

= 4u × number of reactions

mass of hydrogen-1 = 4 × 1.007825 × (1.66 × 10⁻²⁷) × (7.332 × 10³⁷)

mass of hydrogen-1 = 4.91 × 10¹¹ kg s⁻¹

Energy Released in Fusion Reactions (HL IB Physics)

Revision Note

Energy Released in Fusion Reactions

Worked example

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Katie M