Gravitational Field Lines (DP IB Physics: HL): Revision Note

Point Mass Approximation

• For a point outside a uniform sphere, the mass of the sphere may be considered to be a point mass at its centre

  • A uniform sphere is one where its mass is distributed evenly

• The gravitational field lines around a uniform sphere are therefore identical to those around a point mass

• An object can be regarded as a point mass when:

A body covers a very large distance compared to its size, so, to study its motion, its size or dimensions can be neglected

• This applies to celestial bodies such as stars, planets, and moons

• For example, the field lines around a planet meet at its centre of mass

Gravitational field lines around a uniform sphere are identical to those on a point mass

• Radial fields are considered non-uniform fields

  • So, the gravitational field strength g is different depending on how far an object is from the centre of mass of the sphere

• Newton’s universal law of gravitation is extended to spherical masses of uniform density by assuming that their mass is concentrated at their centre i.e. point masses

Representing Gravitational Fields

• Gravitational fields represent the action of gravitational forces between masses, the direction of these forces can be shown using vectors

  • The direction of the vector shows the direction of the gravitational force that would be exerted on a mass if it were placed at that position in the field

  • These vectors are known as field lines (or 'lines of force')

• The direction of a gravitational field is represented by gravitational field lines 

  • Therefore, gravitational field lines also show the direction of acceleration of a mass placed in the field

• Gravitational field lines are always directed toward the centre of mass of a body

  • This is because gravitational forces are attractive only (they are never repulsive)

  • Therefore, masses always attract each other via the gravitational force

• The gravitational field around a point mass will be radial in shape, and the field lines will always point towards the centre of mass

The direction of the gravitational field is shown by the vector field lines

• The gravitational field lines around a point mass are radially inwards

• The gravitational field lines of a uniform field, where the field strength is the same at all points, are represented by equally spaced parallel lines

  • For example, the field lines on the Earth’s surface

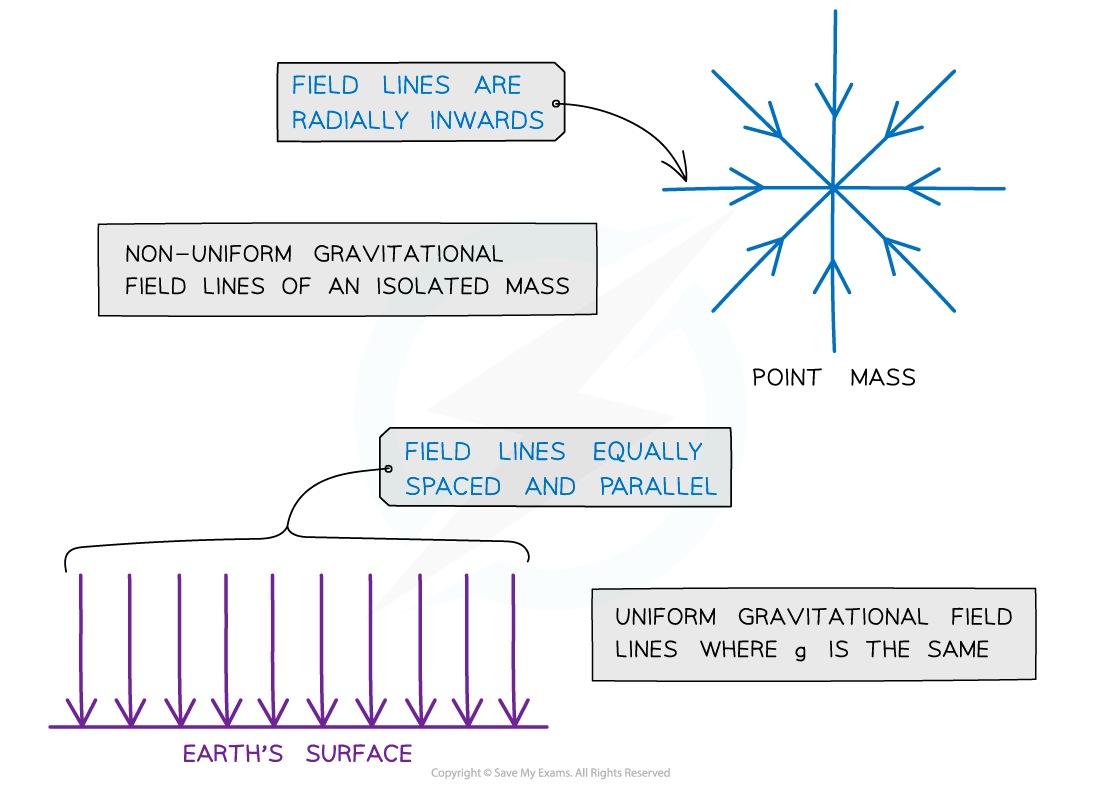
Gravitational field lines for a point mass and a uniform gravitational field

• Radial fields are considered non-uniform fields

  • The gravitational field strength g is different depending on how far you are from the centre

• Parallel field lines on the Earth’s surface are considered a uniform field

  • The gravitational field strength g is the same throughout