Did this video help you?
The Binomial Distribution (DP IB Maths: AI SL): Revision Note
Properties of Binomial Distribution
What is a binomial distribution?
- A binomial distribution is a discrete probability distribution
- A discrete random variable
follows a binomial distribution if it counts the number of successes when an experiment satisfies the following conditions:
- There are a fixed finite number of trials (n)
- The outcome of each trial is independent of the outcomes of the other trials
- There are exactly two outcomes of each trial (success or failure)
- The probability of success is constant (p)
- If
follows a binomial distribution then it is denoted
- n is the number of trials
- p is the probability of success
- The probability of failure is 1 - p which is sometimes denoted as q
- The formula for the probability of r successful trials is given by:
for
where
- You will be expected to use the distribution function on your GDC to calculate probabilities with the binomial distribution
What are the important properties of a binomial distribution?
- The expected number (mean) of successful trials is
-
- You are given this in the formula booklet
- The variance of the number of successful trials is
-
- You are given this in the formula booklet
- Square root to get the standard deviation
- The distribution can be represented visually using a vertical line graph
- If p is close to 0 then the graph has a tail to the right
- If p is close to 1 then the graph has a tail to the left
- If p is close to 0.5 then the graph is roughly symmetrical
- If p = 0.5 then the graph is symmetrical


Did this video help you?
Modelling with Binomial Distribution
How do I set up a binomial model?
- Identify what a trial is in the scenario
- For example: rolling a dice, flipping a coin, checking hair colour
- Identify what the successful outcome is in the scenario
- For example: rolling a 6, landing on tails, having black hair
- Identify the parameters
- n is the number of trials and p is the probability of success in each trial
- Make sure you clearly state what your random variable is
- For example, let
be the number of students in a class of 30 with black hair
- For example, let
What can be modelled using a binomial distribution?
- Anything that satisfies the four conditions
- For example: let
be the number of times a fair coin lands on tails when flipped 20 times:
- A trial is flipping a coin: There are 20 trials so n = 20
- We can assume each coin flip does not affect subsequent coin flips: they are independent
- A success is when the coin lands on tails: Two outcomes - tails or not tails (heads)
- The coin is fair: The probability of tails is constant with p = 0.5
- Sometimes it might seem like there are more than two outcomes
- For example: let
be the number of yellow cars that are in a car park full of 100 cars
- Although there are more than two possible colours of cars, here the trial is whether a car is yellow so there are two outcomes (yellow or not yellow)
would still need to fulfil the other conditions in order to follow a binomial distribution
- For example: let
- Sometimes a sample may be taken from a population
- For example: 30% of people in a city have blue eyes, a sample of 30 people from the city is taken and
is the number of them with blue eyes
- As long as the population is large and the sample is random then it can be assumed that each person has a 30% chance of having blue eyes
- For example: 30% of people in a city have blue eyes, a sample of 30 people from the city is taken and
What can not be modelled using a binomial distribution?
- Anything where the number of trials is not fixed or is infinite
- The number of emails received in an hour
- The number of times a coin is flipped until it lands on heads
- Anything where the outcome of one trial affects the outcome of the other trials
- The number of caramels that a person eats when they eat 5 sweets from a bag containing 6 caramels and 4 marshmallows
- If you eat a caramel for your first sweet then there are less caramels left in the bag when you choose your second sweet
- Anything where there are more than two outcomes of a trial
- A person's shoe size
- The number a dice lands on when rolled
- Anything where the probability of success changes
- The number of times that a person can swim a length of a swimming pool in under a minute when swimming 50 lengths
- The probability of swimming a lap in under a minute will decrease as the person gets tired
- The probability is not constant
- The number of times that a person can swim a length of a swimming pool in under a minute when swimming 50 lengths
- The number of caramels that a person eats when they eat 5 sweets from a bag containing 6 caramels and 4 marshmallows
Examiner Tip
- An exam question might involve different types of distributions so make it clear which distribution is being used for each variable
Worked example
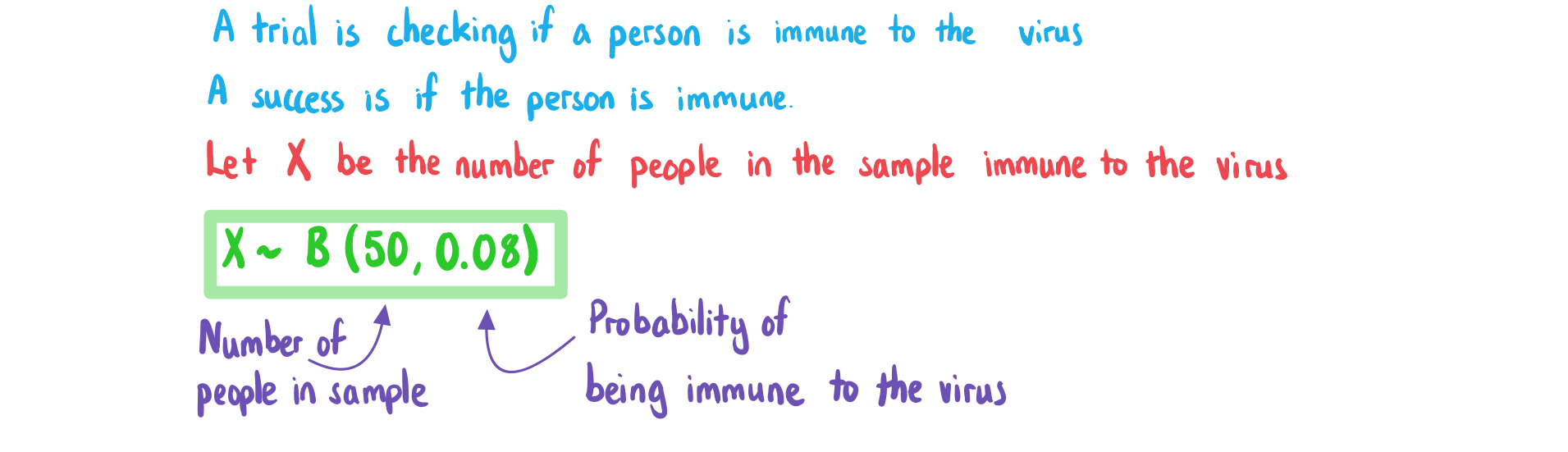
It is known that 8% of a large population are immune to a particular virus. Mark takes a sample of 50 people from this population. Mark uses a binomial model for the number of people in his sample that are immune to the virus.
a)
State the distribution that Mark uses.
b)
State two assumptions that Mark must make in order to use a binomial model.

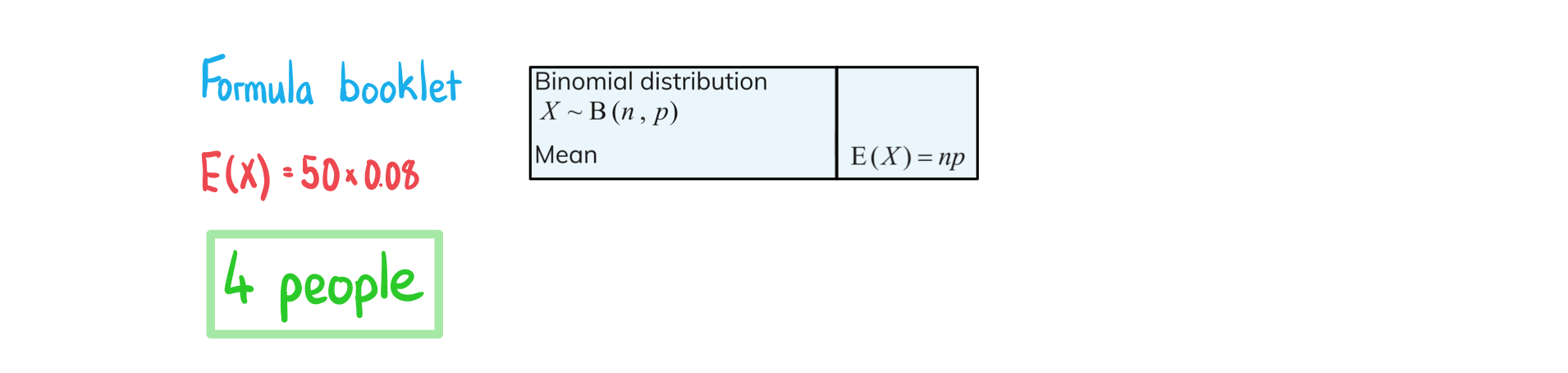
c)
Calculated the expected number of people in the sample that are immune to the virus.

You've read 0 of your 5 free revision notes this week
Sign up now. It’s free!
Did this page help you?

