Pythagoras & Right-Angled Trigonometry (DP IB Applications & Interpretation (AI)): Revision Note
Did this video help you?
Pythagoras
What is the Pythagorean theorem?
Pythagoras’ theorem is formula using the lengths of the sides of a right-angled triangle
The square of the hypotenuse is equal to the sum of the squares of the two shorter sides
The formula can be written as
and
are the lengths of the shorter sides
is the length of the hypotenuse
To find the length of the hypotenuse:
Use
To find the length of a shorter side:
Use
The converse of the theorem is true
If
Then the triangle has a right-angle

Examiner Tips and Tricks
The formula for Pythagoras' theorem is not given in the formula booklet.
Worked Example
ABCDEF is a chocolate bar in the shape of a triangular prism. The end of the chocolate bar is an isosceles triangle where AC = 3 cm and AB = BC = 5 cm. M is the midpoint of AC. This information is shown in the diagram below.
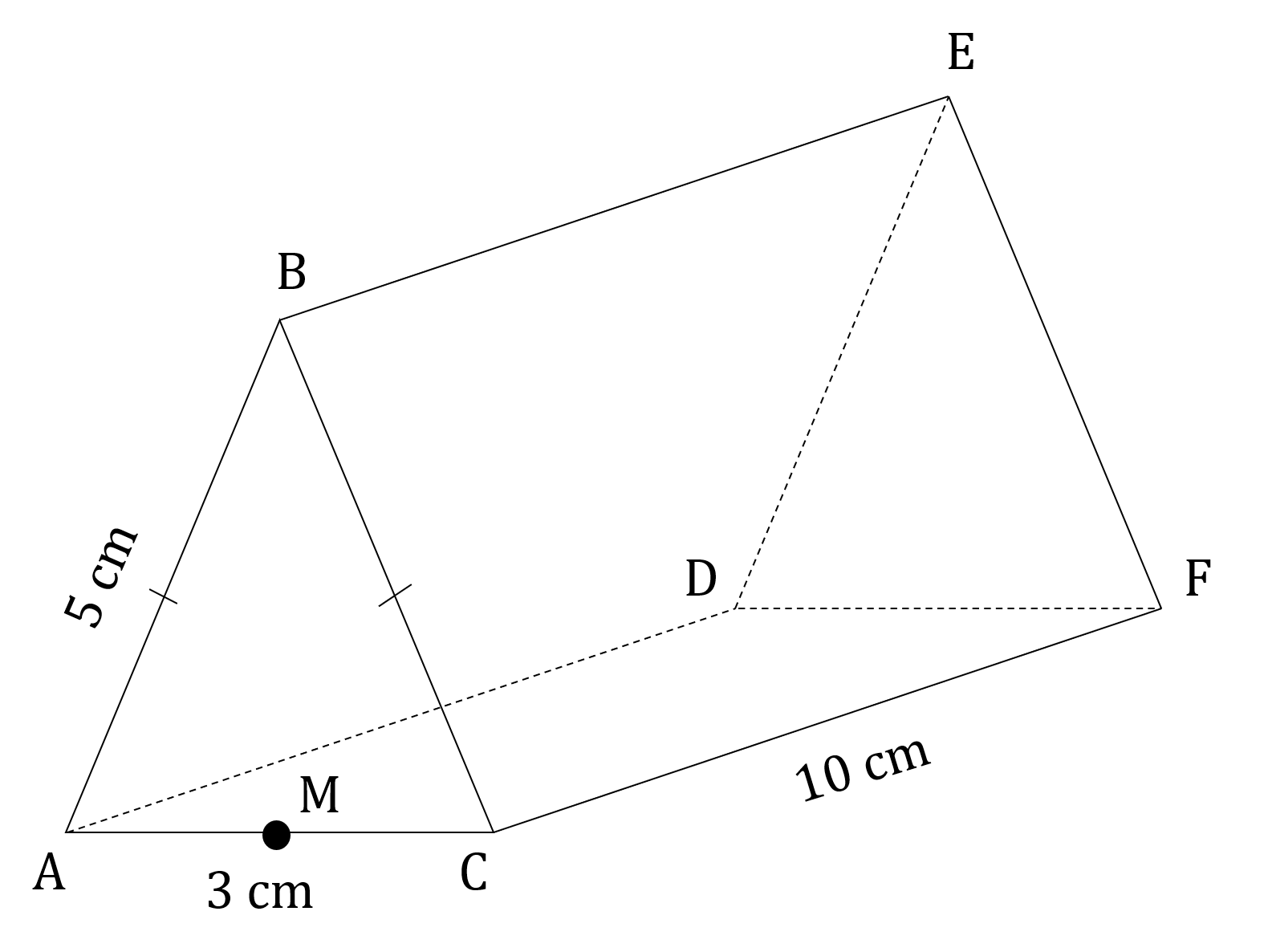
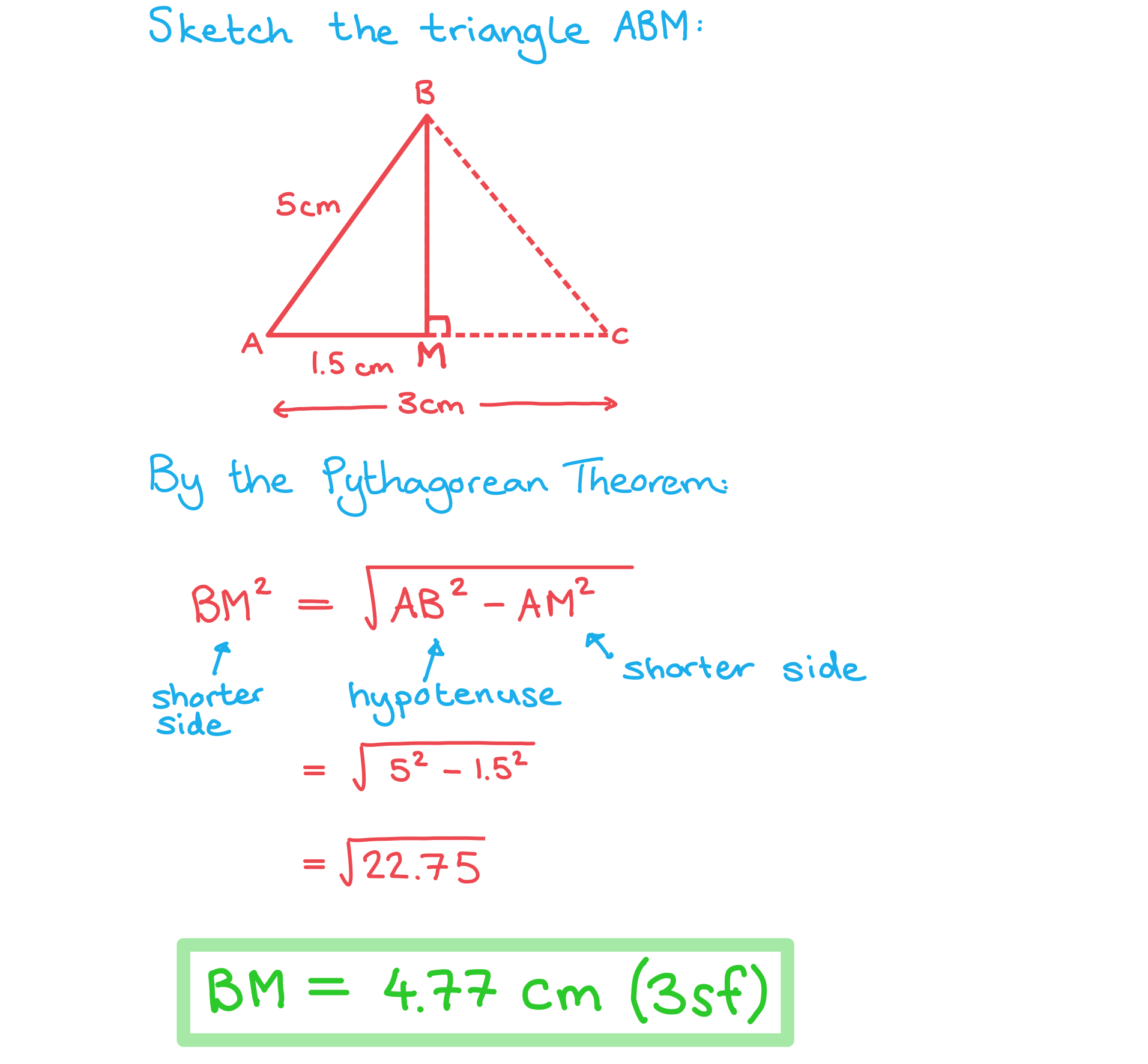
Calculate the length BM.
Did this video help you?
Right-angled trigonometry
What is right-angled trigonometry?
Right-angled trigonometry is used to find the angles and the lengths of the sides of a right-angled triangle
Three trigonometric functions are used to show the ratios between different pairs of sides
sine (sin)
cosine (cos)
tangent (tan)
What are the formulas for sin, cos and tan?
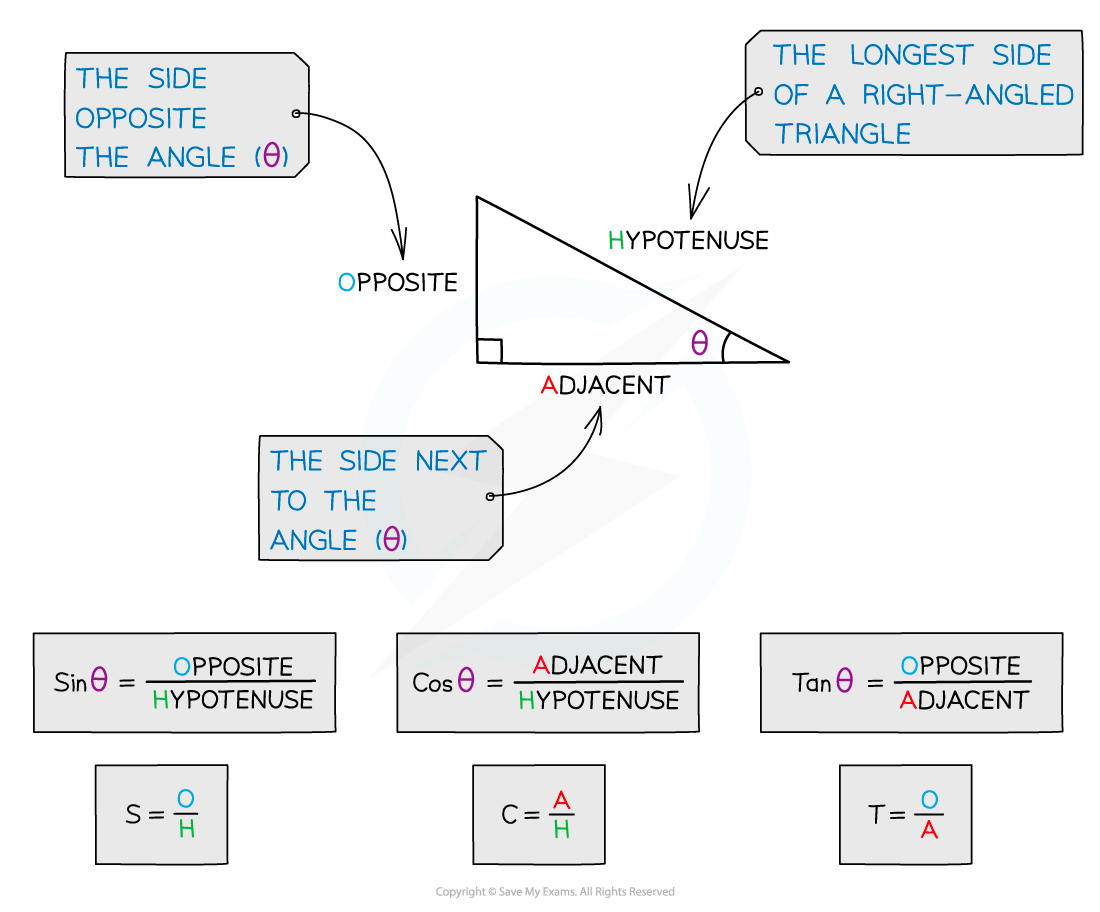
You need to label one angle and all three sides of the right-angled triangle
is one of the acute angles
is the length of the hypotenuse
is the length of the side opposite
is the length of the side adjacent to

The formulas for sin, cos and tan are:
Examiner Tips and Tricks
The mnemonic SOHCAHTOA is often used as a way of remembering the formulas. These are not given in the formula booklet.
How can I use SOHCAHTOA to find missing lengths and angles?
STEP 1
Label the sides of the triangle as H, O and ASTEP 2
Identify which trigonometric ratio to use: sin, cos or tanWrite down the letters of the length you are given or want to find
Find the two letters in SOHCAHTOA to identify which ratio to use
If you have A and H then use cos
STEP 3
Substitute the values into the relevant trigonometric formulaRemember to put brackets around the angle
or
or
STEP 4
Rearrange and solve for the unknown letterYou will either need to multiply or divide to find a missing length
leads to
leads to
You will need to use the inverse trigonometric functions to find a missing angle
leads to
Worked Example
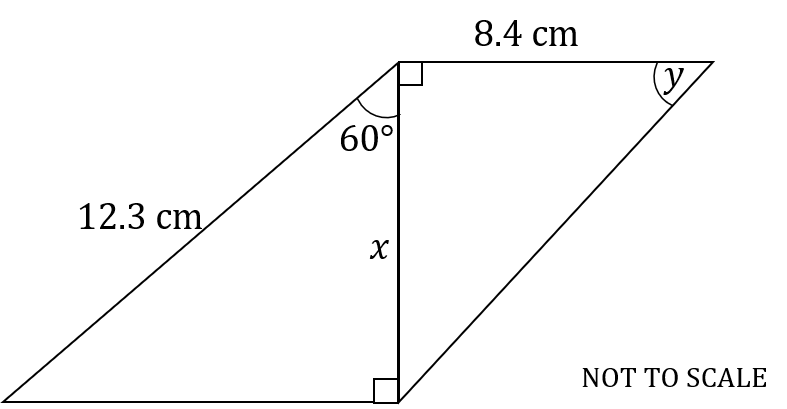
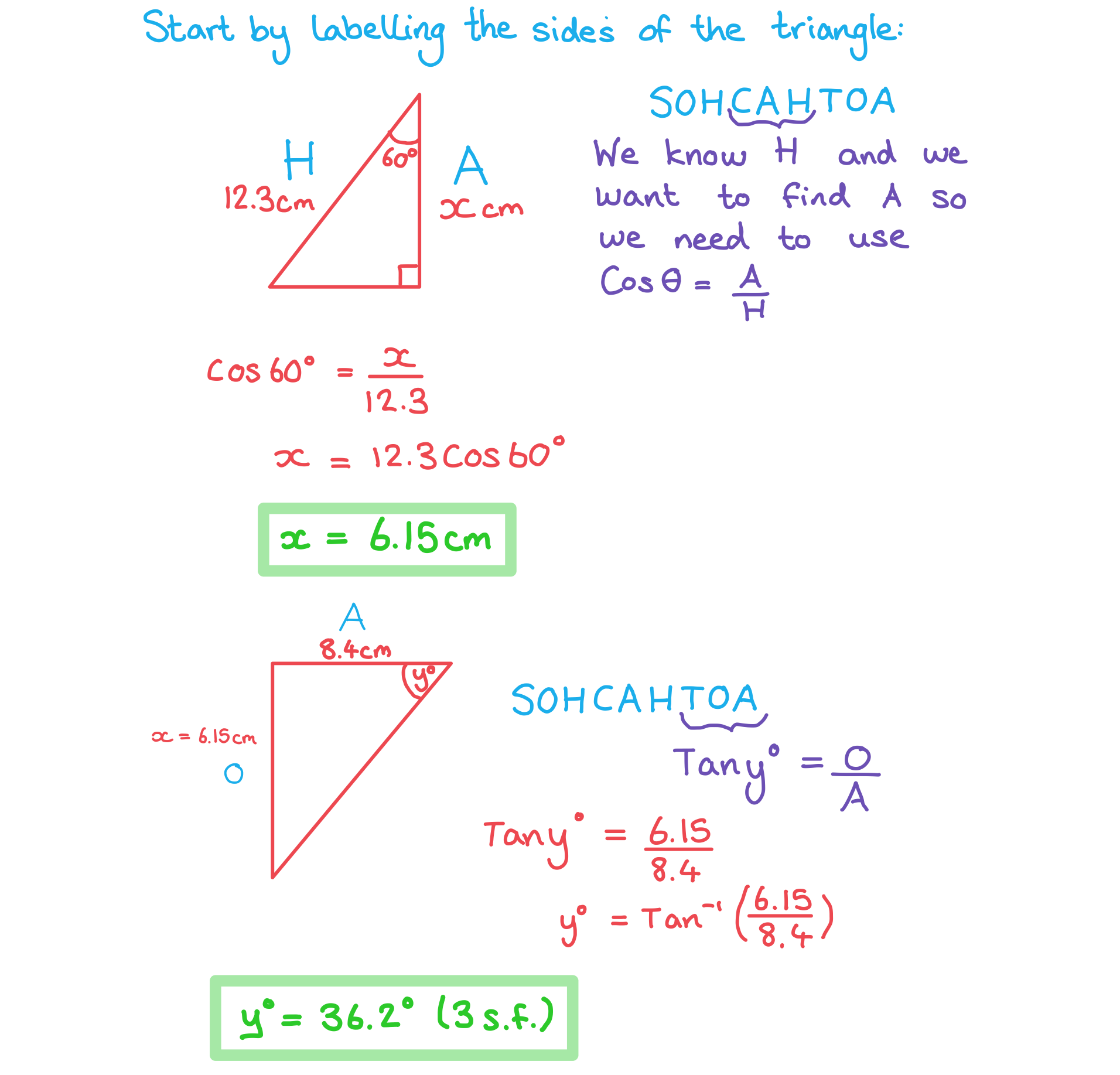
Find the values of and
in the following diagram. Give your answers to 3 significant figures.
Did this video help you?
3D problems
Can I use Pythagoras' theorem and trigonometry in 3D?
You can break a 3D problem into 2D triangles
The formula for the length of the diagonal of a cuboid with dimensions
is
This is sometimes referred to as the 3D version of Pythagoras' theorem
You can find missing angles by drawing right-angled triangles
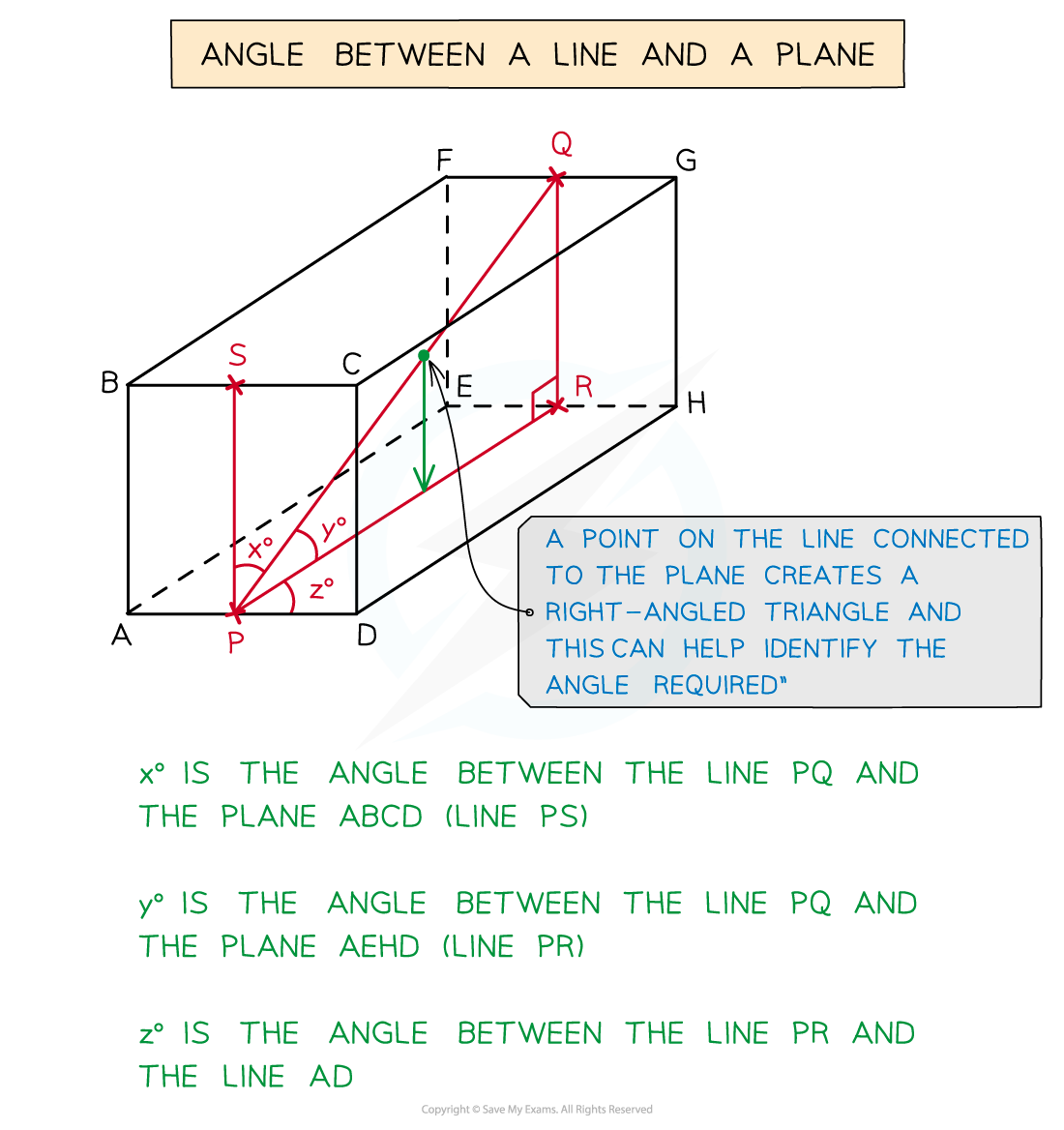
You can find angles between lines and planes
Examiner Tips and Tricks
Draw a big enough sketch so that you can clearly annotate it with missing angles and lengths.
Worked Example
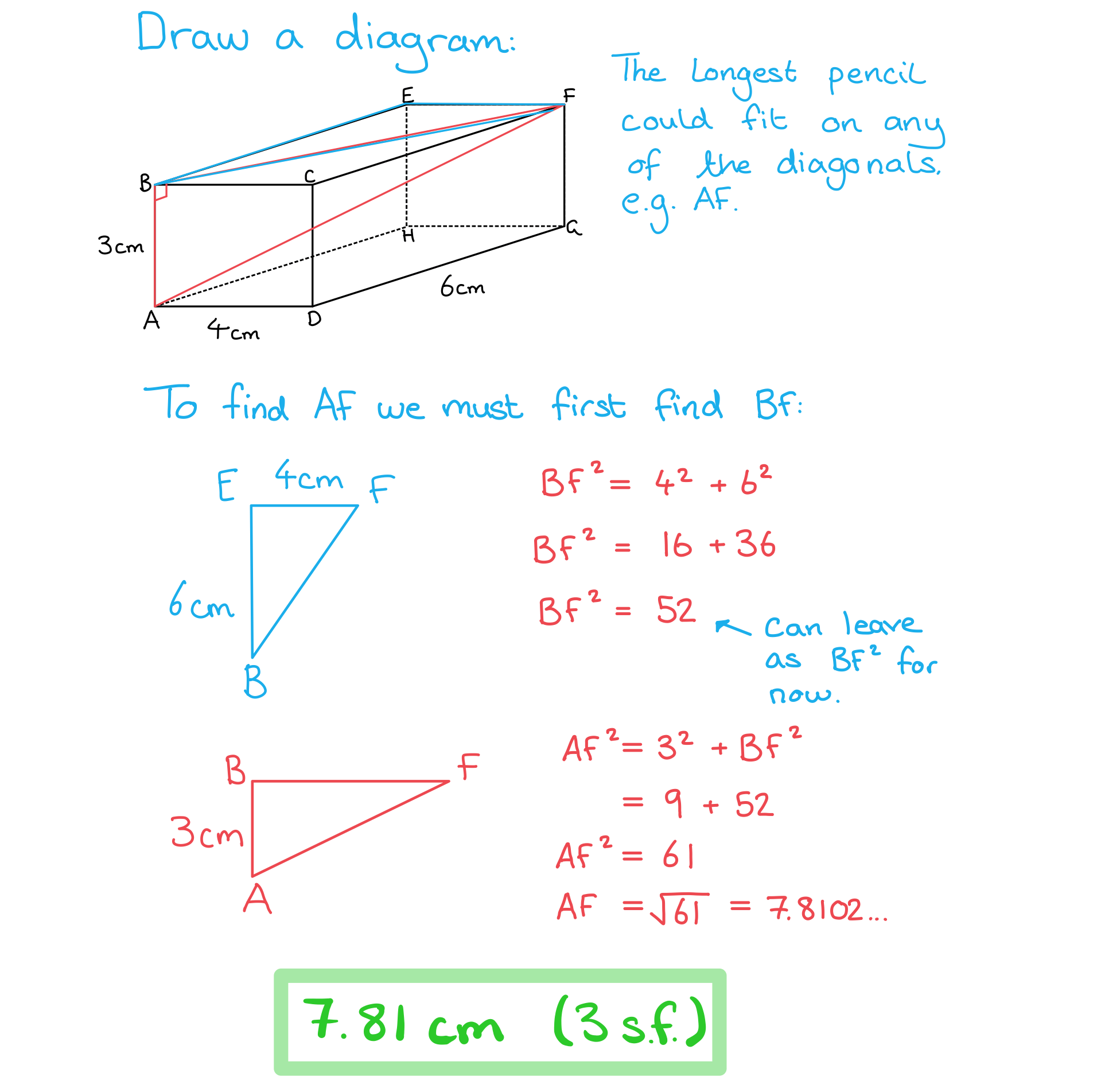
A pencil is being put into a cuboid shaped box. The base of the box has a width of 4 cm and a length of 6 cm. The height of the box is 3 cm. Find:
a) the length of the longest pencil that could fit inside the box,
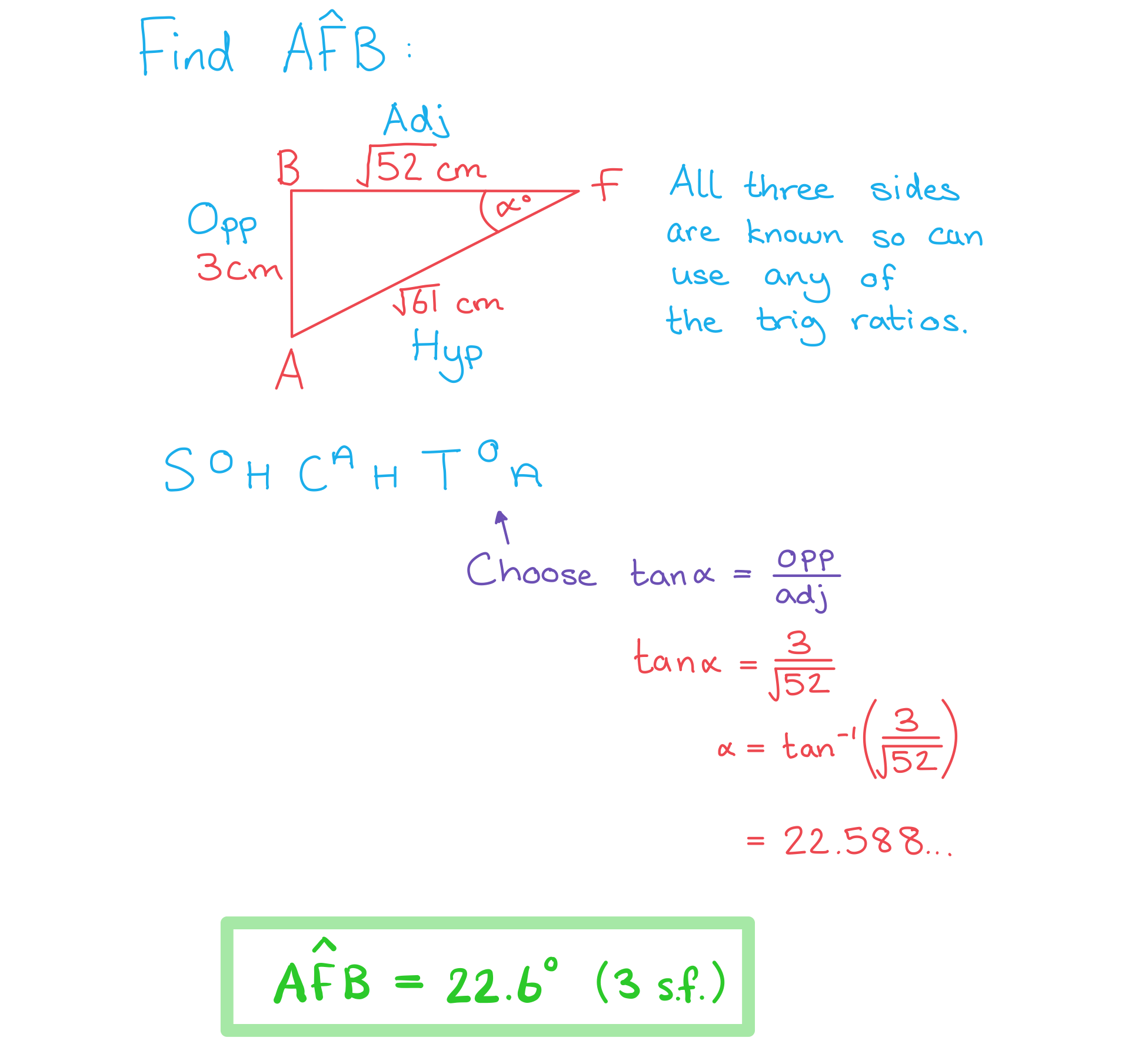
b) the angle that the pencil would make with the top of the box.

Unlock more, it's free!
Did this page help you?