Revision NotesExam QuestionsFlashcards
IBMaths: AA SLDPRevision Notes2. Functions2.4 Further Functions & Graphs2.4.4 Modelling with Functions

Modelling with Functions (DP IB Maths: AA SL)

Revision Note

Dan

Author

Dan

Last updated

Did this video help you?

Modelling with Functions

What is a mathematical model?

  • A mathematical model simplifies a real-world situation so it can be described using mathematics
    • The model can then be used to make predictions
  • Assumptions about the situation are made in order to simplify the mathematics
  • Models can be refined (improved) if further information is available or if the model is compared to real-world data

How do I set up the model?

  • The question could:
    • give you the equation of the model
    • tell you about the relationship
      • It might say the relationship is linear, quadratic, etc
    • ask you to suggest a suitable model
      • Use your knowledge of each model
      • E.g. if it is compound interest then an exponential model is the most appropriate
  • You may have to determine a reasonable domain
    • Consider real-life context
      • E.g. if dealing with hours in a day then 0 less or equal than t less than 24
      • E.g. if dealing with physical quantities (such as length) then, x greater than 0
    • Consider the possible ranges
      • If the outcome cannot be negative then you want to choose a domain which corresponds to a range with no negative values
      • Sketching the graph is helpful to determine a suitable domain

Which models might I need to use?

  • You could be given any model and be expected to use it
  • Common models include:
    • Linear
      • Arithmetic sequences
      • Linear regression
    • Quadratic
      • Projectile motion
      • The height of a cable supporting a bridge
      • Profit
    • Exponential
      • Geometric sequences
      • Exponential growth and decay
      • Compound interest
    • Logarithmic
      • Richter scale for the magnitude of earthquakes
    • Rational
      • Temperature of a cup of coffee
    • Trigonometric
      • The depth of a tide

How do I use a model?

  • You can use a model by substituting in values for the variable to estimate outputs
    • For example: Let h(t) be the height of a football t seconds after being kicked
      • h(3) will be an estimate for the height of the ball 3 seconds after being kicked
  • Given an output you can form an equation with the model to estimate the input
    • For example: Let P(n) be the profit made by selling n items
      • Solving P(n) = 100 will give you an estimate for the number of items needing to be sold to make a profit of 100
  • If your variable is time then substituting t = 0 will give you the initial value according to the model
  • Fully understand the units for the variables
    • If the units of P are measured in thousand dollars then P = 3 represents $3000
  • Look out for key words such as:
    • Initially
    • Minimum/maximum
    • Limiting value

What do I do if some of the parameters are unknown?

  • A general method is to form equations by substituting in given values
    • You can form multiple equations and solve them simultaneously using your GDC
    • This method works for all models
  • The initial value is the value of the function when the variable is 0
    • This is normally one of the parameters in the equation of the model

Worked example

The temperature, T°C, of a cup of coffee is monitored. Initially the temperature is 80°C  and 5 minutes later it is 40°C . It is suggested that the temperature follows the model:

 T left parenthesis t right parenthesis equals A straight e to the power of k t end exponent plus 16 comma space t greater or equal than 0. 

where t is the time, in minutes, after the coffee has been made.

a)
State the value of A.

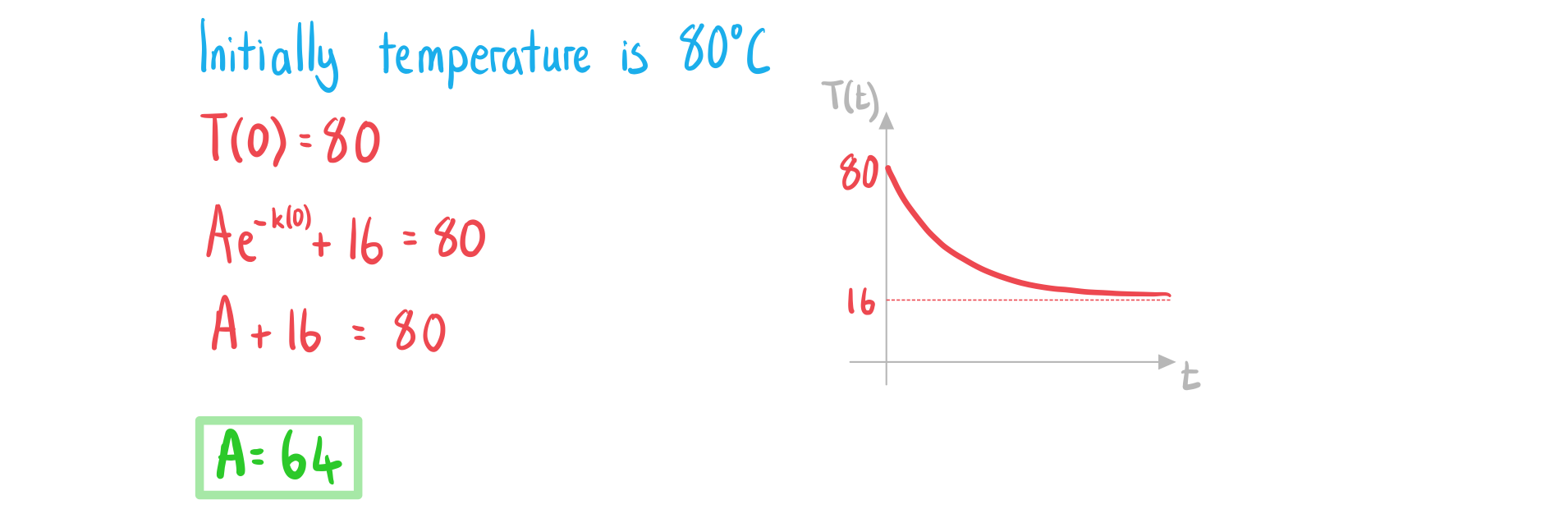
2-4-4-ib-aa-sl-modelling-func-a-we-solution

b)
Find the exact value of k.

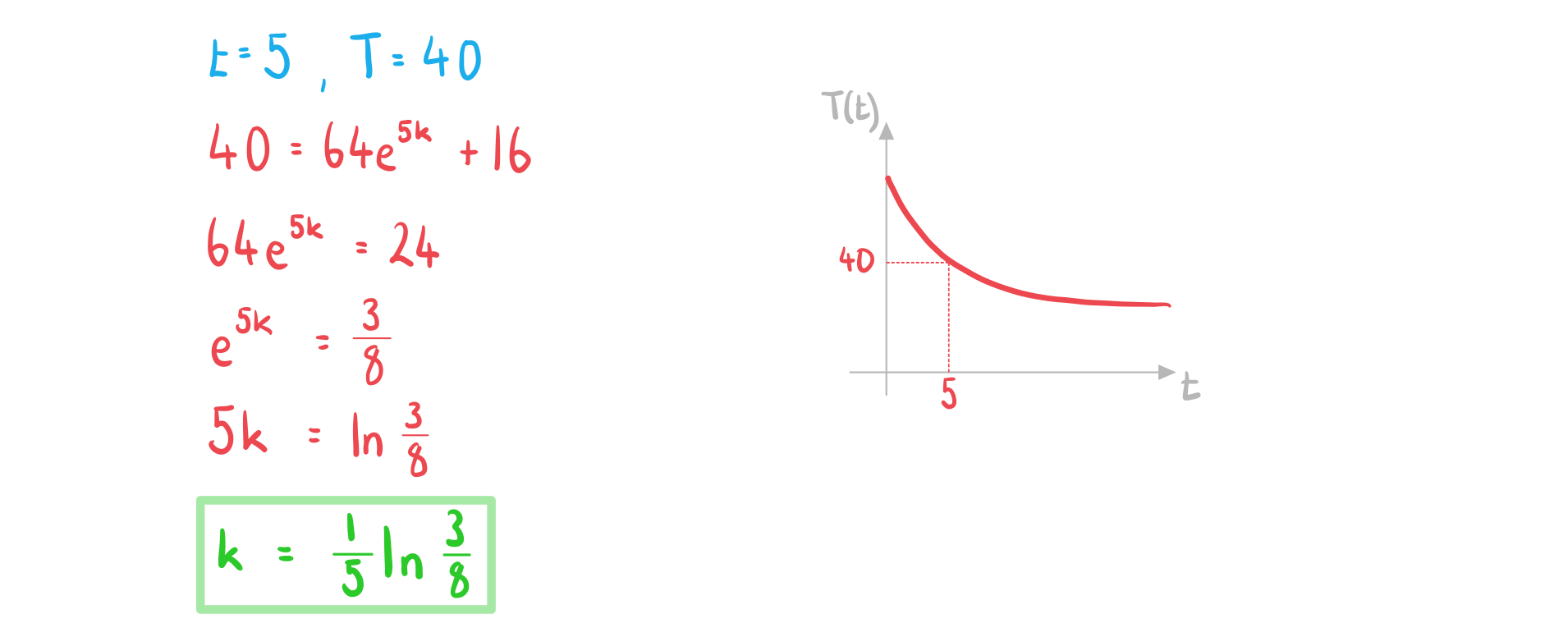
2-4-4-ib-aa-sl-modelling-func-b-we-solution

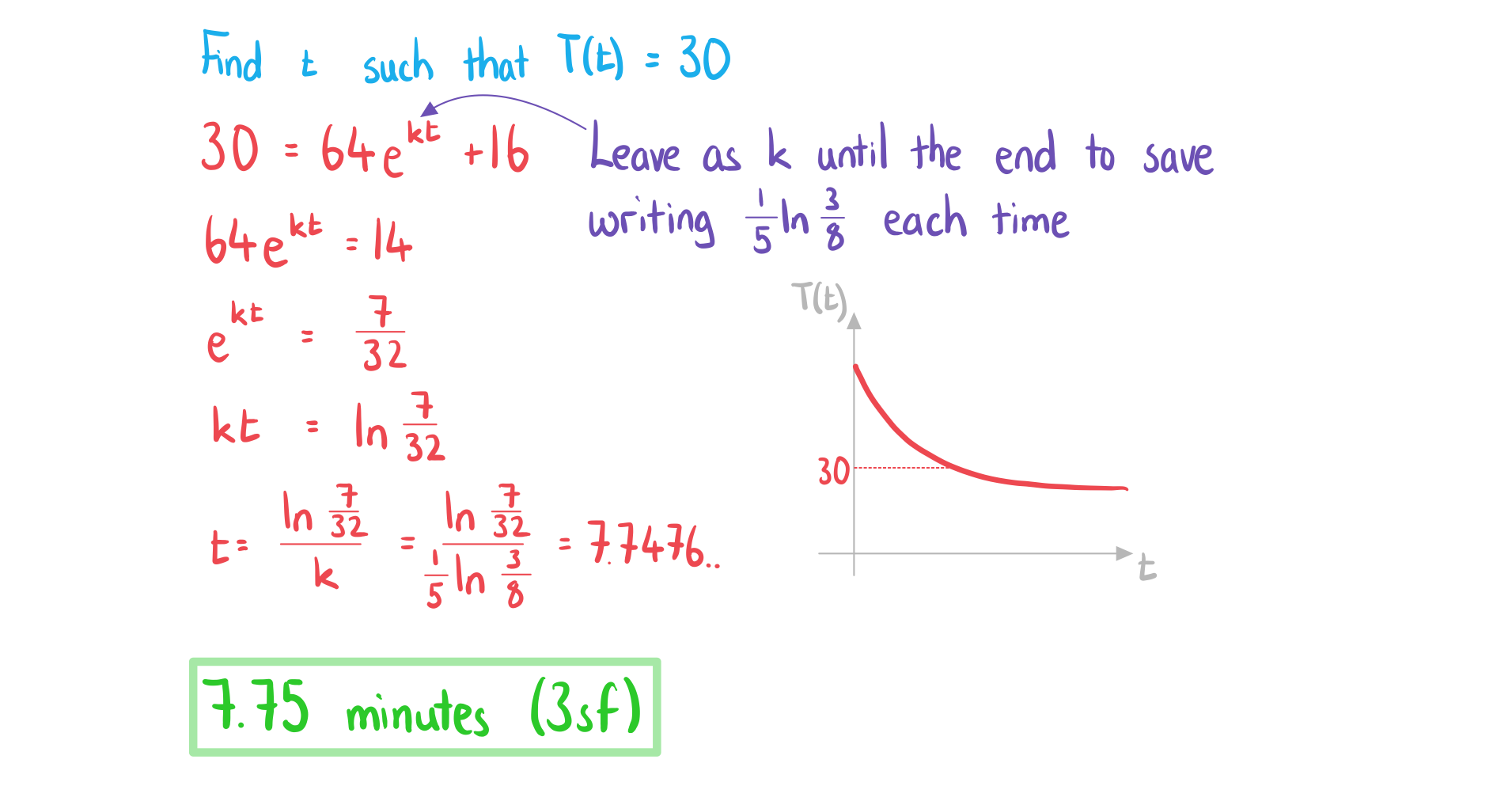
c)
Find the time taken for the temperature of the coffee to reach 30°C.

2-4-4-ib-aa-sl-modelling-func-c-we-solution

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:2.4.3 Solving EquationsNext:2.5.1 Translations of Graphs
    Dan

    Author: Dan

    Expertise: Maths

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.