Quadratic Functions | DP IB Maths: AA SL Revision Notes 2021

Quadratic Functions & Graphs

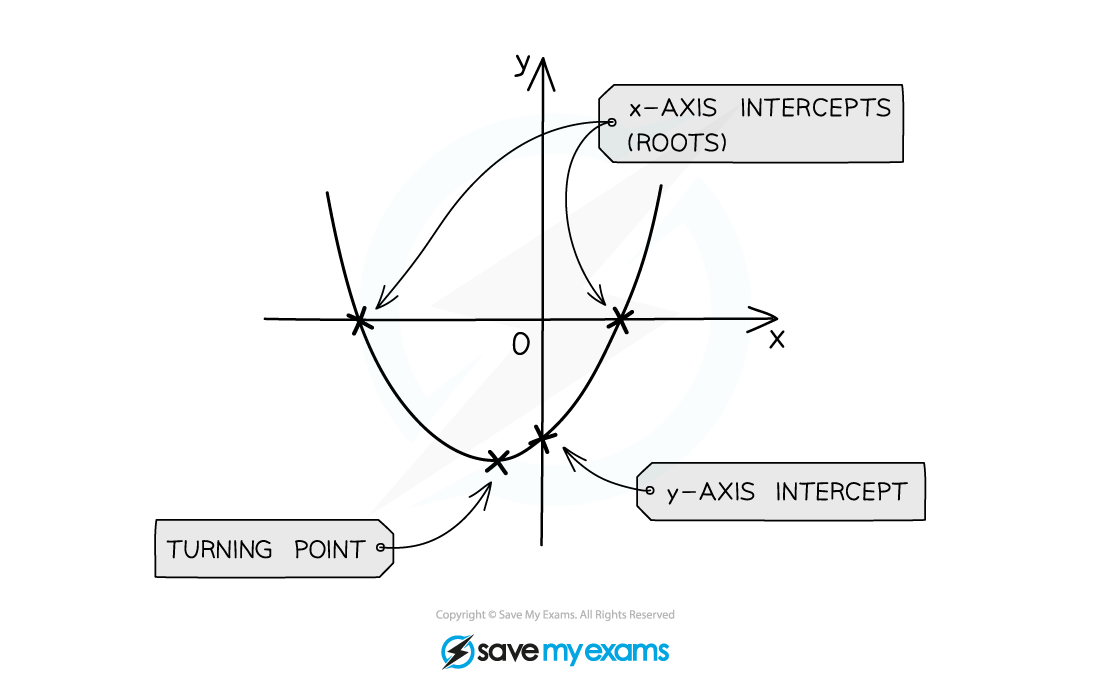
What are the key features of quadratic graphs?

A quadratic graph can be written in the form $y = a x^{2} + b x + c$ where $a \neq 0$
The value of a affects the shape of the curve
- If a is positive the shape is concave up ∪
- If a is negative the shape is concave down ∩
The y-intercept is at the point (0, c)
The zeros or roots are the solutions to $a x^{2} + b x + c = 0$
- These can be found by
  - Factorising
  - Quadratic formula
  - Using your GDC
- These are also called the x-intercepts
- There can be 0, 1 or 2 x-intercepts
  - This is determined by the value of the discriminant
There is an axis of symmetry at $x = - \frac{b}{2 a}$
- This is given in your formula booklet
- If there are two x-intercepts then the axis of symmetry goes through the midpoint of them
The vertex lies on the axis of symmetry
- It can be found by completing the square
- The x-coordinate is $x = - \frac{b}{2 a}$
- The y-coordinate can be found using the GDC or by calculating y when $x = - \frac{b}{2 a}$
- If a is positive then the vertex is the minimum point
- If a is negative then the vertex is the maximum point

What are the equations of a quadratic function?

$f (x) = a x^{2} + b x + c$
- This is the general form
- It clearly shows the y-intercept (0, c)
- You can find the axis of symmetry by $x = - \frac{b}{2 a}$
  - This is given in the formula booklet
$f (x) = a (x - p) (x - q)$
- This is the factorised form
- It clearly shows the roots (p, 0) & (q, 0)
- You can find the axis of symmetry by $x = \frac{p + q}{2}$
$f (x) = a {(x - h)}^{2} + k$
- This is the vertex form
- It clearly shows the vertex (h, k)
- The axis of symmetry is therefore $x = h$
- It clearly shows how the function can be transformed from the graph $y = x^{2}$
  - Vertical stretch by scale factor a
  - Translation by vector $(\begin{matrix} h \\ k \end{matrix})$

How do I find an equation of a quadratic?

If you have the roots x = p and x = q...
- Write in factorised form $y = a (x - p) (x - q)$
- You will need a third point to find the value of a
If you have the vertex (h, k) then...
- Write in vertex form $y = a {(x - h)}^{2} + k$
- You will need a second point to find the value of a
If you have three random points (x₁, y₁), (x₂, y₂) & (x₃, y₃) then...
- Write in the general form $y = a x^{2} + b x + c$
- Substitute the three points into the equation
- Form and solve a system of three linear equations to find the values of a, b & c

Examiner Tip

Use your GDC to find the roots and the turning point of a quadratic function
- You do not need to factorise or complete the square
- It is good exam technique to sketch the graph from your GDC as part of your working

Worked example

The diagram below shows the graph of $y = f (x)$ , where $f (x)$ is a quadratic function.

The intercept with the $y$ -axis and the vertex have been labelled.

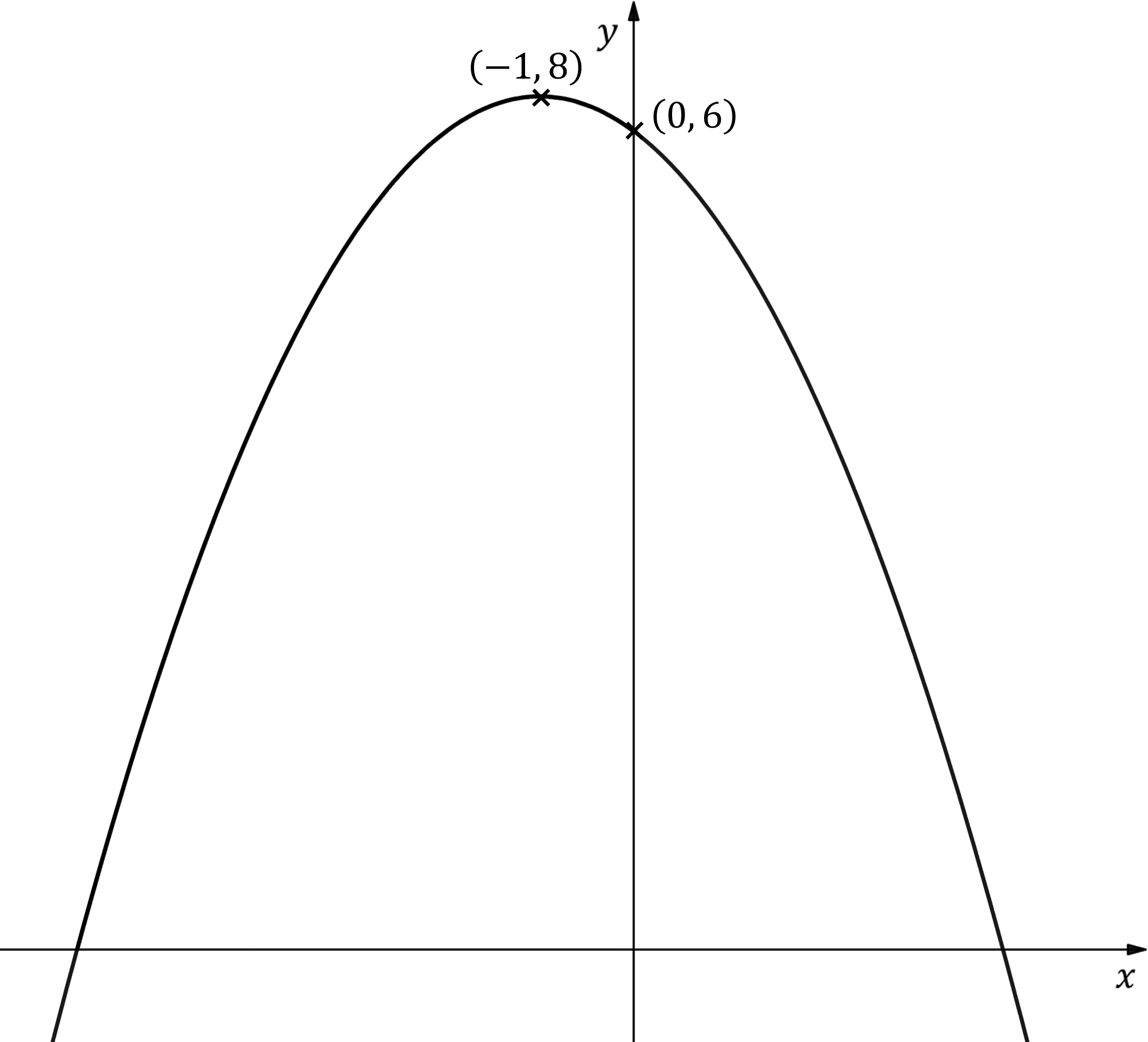
2-2-1-ib-aa-sl-we-image

Write down an expression for $y = f (x)$ .

2-2-1-ib-aa-sl-quad-function-we-solution

Quadratic Functions (DP IB Maths: AA SL)

Revision Note

What are the key features of quadratic graphs?

What are the equations of a quadratic function?

How do I find an equation of a quadratic?

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

1. Number & Algebra

1.1 Number Toolkit

1.1.1 Standard Form

1.1.2 Laws of Indices

1.2 Exponentials & Logs

1.2.1 Introduction to Logarithms

1.2.2 Laws of Logarithms

1.2.3 Solving Exponential Equations

1.3 Sequences & Series

1.3.1 Language of Sequences & Series

1.3.2 Arithmetic Sequences & Series

1.3.3 Geometric Sequences & Series

1.3.4 Applications of Sequences & Series

1.3.5 Compound Interest & Depreciation

1.4 Proof & Reasoning

1.4.1 Proof

1.5 Binomial Theorem

1.5.1 Binomial Theorem

2. Functions

2.1 Linear Functions & Graphs

2.1.1 Equations of a Straight Line

2.2 Quadratic Functions & Graphs

2.2.1 Quadratic Functions

2.2.2 Factorising & Completing the Square

2.2.3 Solving Quadratics

2.2.4 Quadratic Inequalities

2.2.5 Discriminants

2.3 Functions Toolkit

2.3.1 Language of Functions

2.3.2 Composite & Inverse Functions

2.3.3 Graphing Functions

2.4 Further Functions & Graphs

2.4.1 Reciprocal & Rational Functions

2.4.2 Exponential & Logarithmic Functions

2.4.3 Solving Equations

2.4.4 Modelling with Functions

2.5 Transformations of Graphs

2.5.1 Translations of Graphs

2.5.2 Reflections of Graphs

2.5.3 Stretches of Graphs

2.5.4 Composite Transformations of Graphs

3. Geometry & Trigonometry

3.1 Geometry Toolkit

3.1.1 Coordinate Geometry

3.1.2 Radian Measure

3.1.3 Arcs & Sectors

3.2 Geometry of 3D Shapes

3.2.1 3D Coordinate Geometry

3.2.2 Volume & Surface Area

3.3 Trigonometry

3.3.1 Pythagoras & Right-Angled Trigonometry

3.3.2 Non Right-Angled Trigonometry

3.3.3 Applications of Trigonometry & Pythagoras

3.4 Further Trigonometry

3.4.1 The Unit Circle

3.4.2 Exact Values

3.5 Trigonometric Functions & Graphs

3.5.1 Graphs of Trigonometric Functions

3.5.2 Transformations of Trigonometric Functions

3.5.3 Modelling with Trigonometric Functions

3.6 Trigonometric Equations & Identities

3.6.1 Simple Identities

3.6.2 Double Angle Formulae

3.6.3 Relationship Between Trigonometric Ratios

3.6.4 Linear Trigonometric Equations

3.6.5 Quadratic Trigonometric Equations

4. Statistics & Probability

4.1 Statistics Toolkit

4.1.1 Sampling & Data Collection

4.1.2 Statistical Measures

4.1.3 Frequency Tables

4.1.4 Linear Tranformations of Data