First Principles Differentiation

What is differentiation from first principles?

Differentiation from first principles uses the definition of the derivative of a function f(x)
The definition is

$f' (x) = \lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$\lim_{h \to 0}$ means the 'limit as h tends to zero'
When $h = 0$ , $\frac{f (x + h) - f (x)}{h} = \frac{f (x) - f (x)}{0} = \frac{0}{0}$ which is undefined
- Instead we consider what happens as h gets closer and closer to zero
Differentiation from first principles means using that definition to show what the derivative of a function is
The first principles definition (formula) is in the formula booklet

How do I differentiate from first principles?

STEP 1: Identify the function f(x) and substitute this into the first principles formula

e.g. Show, from first principles, that the derivative of 3x² is 6x

f (x) = 3 x^{2}

f' (x) = \underset{h \to 0}{l i m} \frac{f (x + h) - f (x)}{h} = \underset{h \to 0}{l i m} \frac{3 {(x + h)}^{2} - 3 x^{2}}{h^{}}

STEP 2: Expand f(x+h) in the numerator

f' (x) = \underset{h \to 0}{l i m} \frac{3 (x^{2} + 2 h x + h^{2}) - 3 x^{2}}{h}

f' (x) = \underset{h \to 0}{l i m} \frac{3 x^{2} + 6 h x + 3 h^{2} - 3 x^{2}}{h}

STEP 3: Simplify the numerator, factorise and cancel h with the denominator

f' (x) = \underset{h \to 0}{l i m} \frac{h (6 x + 3 h)}{h}

STEP 4: Evaluate the remaining expression as h tends to zero

f' (x) = \underset{h \to 0}{l i m} (6 x + 3 h) = 6 x

As h \to 0, (6 x + 3 h) \to (6 x + 0) \to 6 x

∴

The derivative of

3 x^{2}

6 x

Examiner Tip

Most of the time you will not use first principles to find the derivative of a function (there are much quicker ways!)
However, you can be asked to demonstrate differentiation from first principles
To get full marks make sure you are are writing lim h -> 0 right up until the concluding sentence!

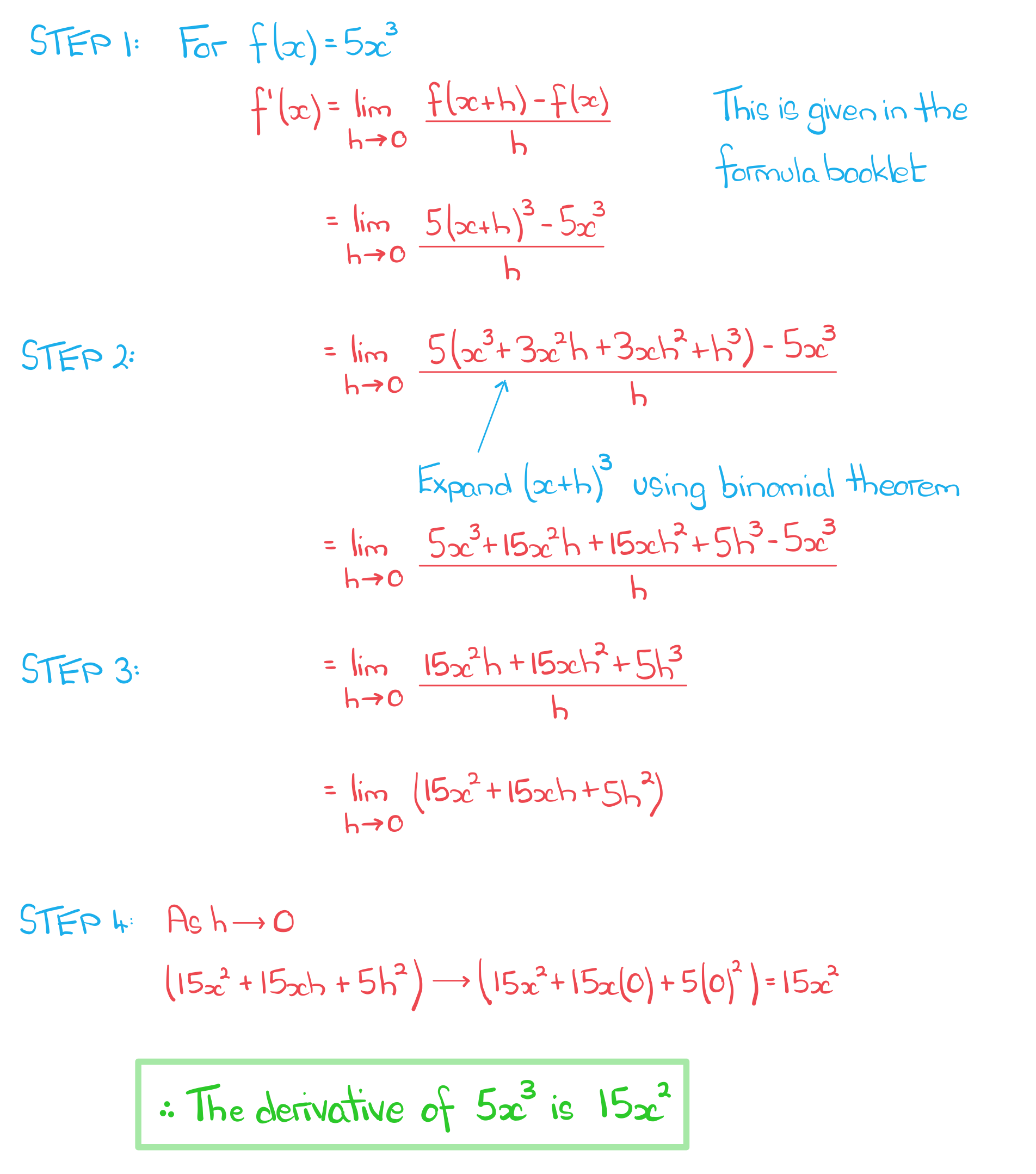
Worked example

Prove, from first principles, that the derivative of $5 x^{3}$ is $15 x^{2}$ .

first-principles-diff-corrected

First Principles Differentiation (DP IB Maths: AA HL)

Revision Note

What is differentiation from first principles?

How do I differentiate from first principles?

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

1. Number & Algebra

1.1 Number & Algebra Toolkit

1.1.1 Standard Form

1.1.2 Laws of Indices

1.1.3 Partial Fractions

1.2 Exponentials & Logs

1.2.1 Introduction to Logarithms

1.2.2 Laws of Logarithms

1.2.3 Solving Exponential Equations

1.3 Sequences & Series

1.3.1 Language of Sequences & Series

1.3.2 Arithmetic Sequences & Series

1.3.3 Geometric Sequences & Series

1.3.4 Applications of Sequences & Series

1.3.5 Compound Interest & Depreciation

1.4 Simple Proof & Reasoning

1.4.1 Proof

1.5 Further Proof & Reasoning

1.5.1 Proof by Induction

1.5.2 Proof by Contradiction

1.6 Binomial Theorem

1.6.1 Binomial Theorem

1.6.2 Extension of The Binomial Theorem

1.7 Permutations & Combinations

1.7.1 Counting Principles

1.7.2 Permutations & Combinations

1.8 Complex Numbers

1.8.1 Intro to Complex Numbers

1.8.2 Modulus & Argument

1.8.3 Introduction to Argand Diagrams

1.9 Further Complex Numbers

1.9.1 Geometry of Complex Numbers

1.9.2 Forms of Complex Numbers

1.9.3 Complex Roots of Polynomials

1.9.4 De Moivre's Theorem

1.9.5 Roots of Complex Numbers

1.10 Systems of Linear Equations

1.10.1 Systems of Linear Equations

1.10.2 Algebraic Solutions

2. Functions

2.1 Linear Functions & Graphs

2.1.1 Equations of a Straight Line

2.2 Quadratic Functions & Graphs

2.2.1 Quadratic Functions

2.2.2 Factorising & Completing the Square

2.2.3 Solving Quadratics

2.2.4 Quadratic Inequalities

2.2.5 Discriminants

2.3 Functions Toolkit

2.3.1 Language of Functions

2.3.2 Composite & Inverse Functions

2.3.3 Symmetry of Functions

2.3.4 Graphing Functions

2.4 Other Functions & Graphs

2.4.1 Exponential & Logarithmic Functions

2.4.2 Solving Equations

2.4.3 Modelling with Functions

2.5 Reciprocal & Rational Functions

2.5.1 Reciprocal & Rational Functions

2.6 Transformations of Graphs

2.6.1 Translations of Graphs

2.6.2 Reflections of Graphs

2.6.3 Stretches Graphs

2.6.4 Composite Transformations of Graphs

2.7 Polynomial Functions

2.7.1 Factor & Remainder Theorem

2.7.2 Polynomial Division

2.7.3 Polynomial Functions

2.7.4 Roots of Polynomials

2.8 Inequalities

2.8.1 Solving Inequalities Graphically

2.8.2 Polynomial Inequalities

2.9 Further Functions & Graphs