Second Order Derivatives (DP IB Analysis & Approaches (AA)): Revision Note
Did this video help you?
Second order derivatives
What is the second order derivative of a function?
If you differentiate the derivative of a function (i.e. differentiate the function a second time) you get the second order derivative of the function
There are two forms of notation for the second order derivative
(First order derivative)
(Second order derivative)
Note the position of the superscript 2’s
differentiating twice (so
) with respect to
twice (so
)
The second order derivative can be referred to simply as the second derivative
Similarly, the first order derivative can be called just the first derivative
A first order derivative is the rate of change of a function
a second order derivative is the rate of change of the rate of change of a function
i.e. the rate of change of the function’s gradient
Second order derivatives can be used to
test for local minimum and maximum points
help determine the nature of stationary points
determine the concavity of a function
help graph the derivative of a function
How do I find a second order derivative of a function?
By differentiating twice!
This may involve
rewriting fractions, roots, etc. as negative and/or fractional powers
differentiating trigonometric functions, exponentials and logarithms
using the chain rule
using the product or quotient rules
Examiner Tips and Tricks
It is easy to make mistakes with negative and/or fractional powers when finding second derivatives, so work carefully through each term.
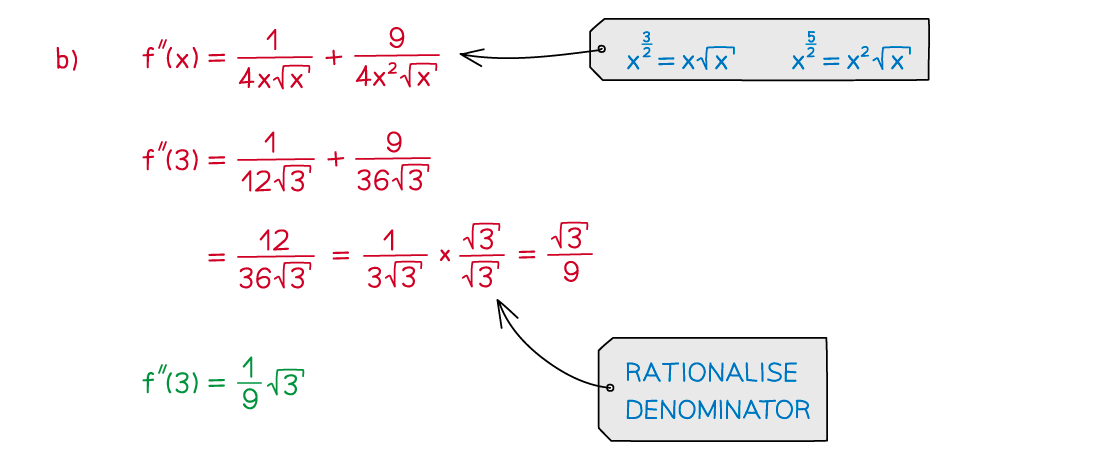
Worked Example
Given that
a) Find and
.

b) Evaluate.
Give your answer in the form , where
is an integer and
is a rational number.

Unlock more, it's free!
Did this page help you?