Differentiating Special Functions (DP IB Maths: AA HL)

Revision Note

Author

Paul

Last updated

14 February 2024

Did this video help you?

Differentiating Trig Functions

How do I differentiate sin, cos and tan?

The derivative of $y = \sin x$ is $\frac{d y}{d x} = \cos x$
The derivative of $y = \cos x$ is $\frac{d y}{d x} = - \sin x$
The derivative of $y = \tan x$ is $\frac{d y}{d x} = \sec^{2} x$
- This result can be derived using quotient rule
For the linear function $a x + b$ , where $a$ and $b$ are constants,
- the derivative of $y = \sin (a x + b)$ is $\frac{d y}{d x} = a \cos (a x + b)$
- the derivative of $y = \cos (a x + b)$ is $\frac{d y}{d x} = - a \sin (a x + b)$
- the derivative of $y = \tan (a x + b)$ is $\frac{d y}{d x} = a \sec^{2} (a x + b)$
For the general function $f (x)$ ,
- the derivative of $y = \sin (f (x))$ is $\frac{d y}{d x} = f^{'} (x) \cos (f (x))$
- the derivative of $y = \cos (f (x))$ is $\frac{d y}{d x} = - f' (x) \sin (f (x))$
- the derivative of $y = \tan (f (x))$ is $\frac{d y}{d x} = f' (x) \sec^{2} (f (x))$
These last three results can be derived using the chain rule
For calculus with trigonometric functions angles must be measured in radians
- Ensure you know how to change the angle mode on your GDC

Examiner Tip

As soon as you see a question involving differentiation and trigonometry put your GDC into radians mode

Worked example

Find

f' (x)

for the functions

$f (x) = \sin x$
$f (x) = \cos (5 x + 1)$

5-2-1-ib-hl-ai-aa-extraaa-we1a-soltn

b) A curve has equation

y = \tan (6 x^{2} - \frac{π}{4})

Find the gradient of the tangent to the curve at the point where

x = \frac{\sqrt{π}}{2}

Give your answer as an exact value.

5-2-1-ib-hl-ai-aa-extraaa-we1b-soltn-

Did this video help you?

Differentiating e^x & lnx

How do I differentiate exponentials and logarithms?

The derivative of $y = e^{x}$ is $\frac{d y}{d x} = e^{x}$ where $x \in ℝ$
The derivative of $y = \ln x$ is $\frac{d y}{d x} = \frac{1}{x}$ where $x > 0$
For the linear function $a x + b$ , where $a$ and $b$ are constants,
- the derivative of $y = e^{(a x + b)}$ is $\frac{d y}{d x} = a e^{(a x + b)}$
- the derivative of $y = \ln (a x + b)$ is $\frac{d y}{d x} = \frac{a}{(a x + b)}$
  - in the special case $b = 0$ , $\frac{d y}{d x} = \frac{1}{x}$ ( $a$ 's cancel)
For the general function $f (x)$ ,
- the derivative of $y = e^{f (x)}$ is $\frac{d y}{d x} = f' (x) e^{f (x)}$
- the derivative of $y = \ln (f (x))$ is $\frac{d y}{d x} = \frac{f' (x)}{f (x)}$
The last two sets of results can be derived using the chain rule

Examiner Tip

Remember to avoid the common mistakes:
- the derivative of $\ln k x$ with respect to $x$ is $\frac{1}{x}$ , NOT $\frac{k}{x}$
- the derivative of $e^{k x}$ with respect to $x$ is $k e^{k x}$ , NOT $k x e^{k x - 1}$

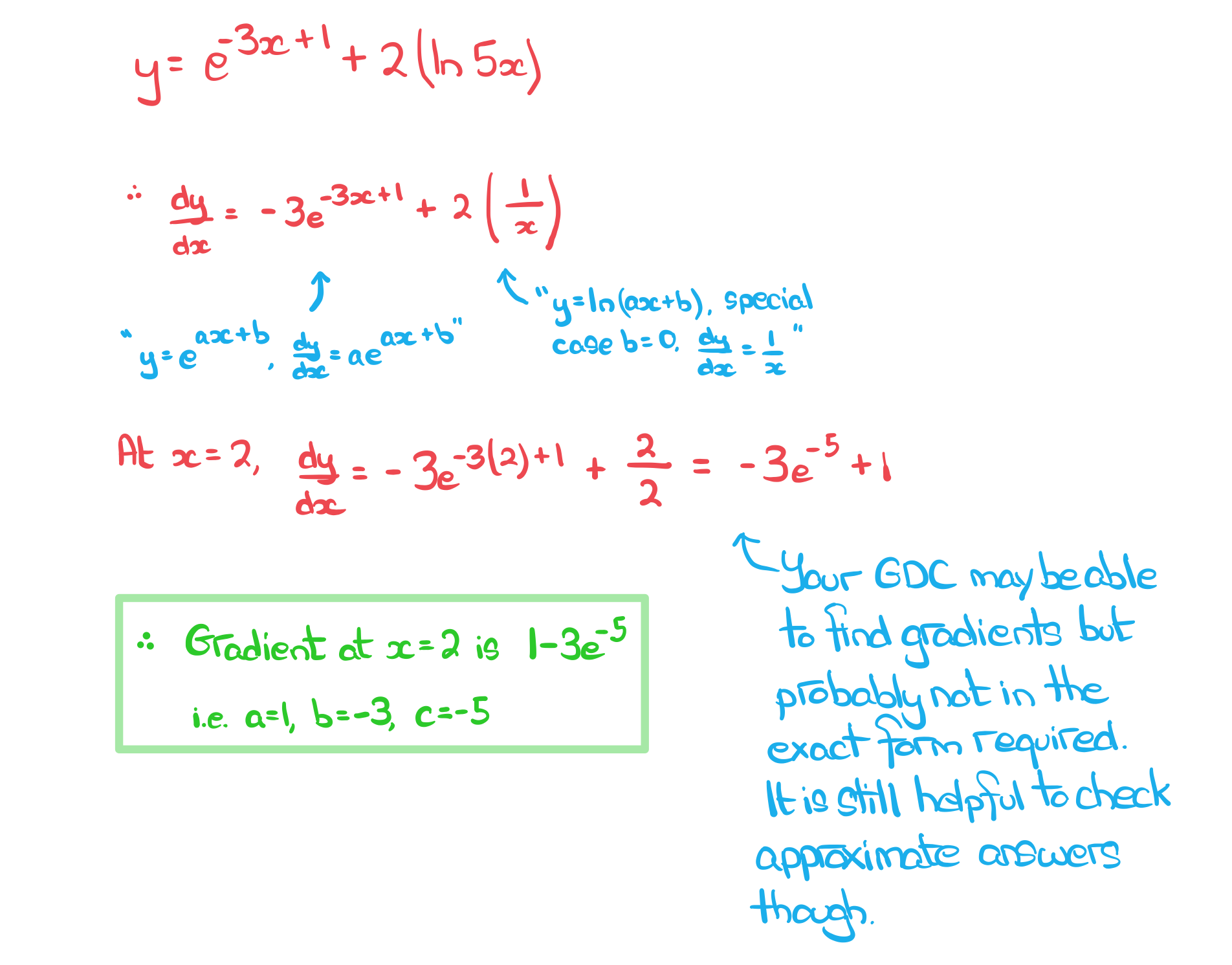
Worked example

A curve has the equation $y = e^{- 3 x + 1} + 2 \ln 5 x$ .

Find the gradient of the curve at the point where $x = 2$ giving your answer in the form $y = a + b e^{c}$ , where $a, b$ and $c$ are integers to be found.

5-2-1-ib-sl-aa-only-we2-soltn

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Test yourself Flashcards

Did this page help you?

Previous:5.1.2 Applications of DifferentiationNext:5.2.2 Techniques of Differentiation

Differentiating Special Functions (DP IB Maths: AA HL)

Revision Note

How do I differentiate sin, cos and tan?

How do I differentiate exponentials and logarithms?

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

1. Number & Algebra

1.1 Number & Algebra Toolkit

1.1.1 Standard Form

1.1.2 Laws of Indices

1.1.3 Partial Fractions

1.2 Exponentials & Logs

1.2.1 Introduction to Logarithms

1.2.2 Laws of Logarithms

1.2.3 Solving Exponential Equations

1.3 Sequences & Series

1.3.1 Language of Sequences & Series

1.3.2 Arithmetic Sequences & Series

1.3.3 Geometric Sequences & Series

1.3.4 Applications of Sequences & Series

1.3.5 Compound Interest & Depreciation

1.4 Simple Proof & Reasoning

1.4.1 Proof

1.5 Further Proof & Reasoning

1.5.1 Proof by Induction

1.5.2 Proof by Contradiction

1.6 Binomial Theorem

1.6.1 Binomial Theorem

1.6.2 Extension of The Binomial Theorem

1.7 Permutations & Combinations

1.7.1 Counting Principles

1.7.2 Permutations & Combinations

1.8 Complex Numbers

1.8.1 Intro to Complex Numbers

1.8.2 Modulus & Argument

1.8.3 Introduction to Argand Diagrams

1.9 Further Complex Numbers

1.9.1 Geometry of Complex Numbers

1.9.2 Forms of Complex Numbers

1.9.3 Complex Roots of Polynomials

1.9.4 De Moivre's Theorem

1.9.5 Roots of Complex Numbers

1.10 Systems of Linear Equations

1.10.1 Systems of Linear Equations

1.10.2 Algebraic Solutions

2. Functions

2.1 Linear Functions & Graphs

2.1.1 Equations of a Straight Line

2.2 Quadratic Functions & Graphs

2.2.1 Quadratic Functions

2.2.2 Factorising & Completing the Square

2.2.3 Solving Quadratics

2.2.4 Quadratic Inequalities

2.2.5 Discriminants

2.3 Functions Toolkit

2.3.1 Language of Functions

2.3.2 Composite & Inverse Functions

2.3.3 Symmetry of Functions

2.3.4 Graphing Functions

2.4 Other Functions & Graphs

2.4.1 Exponential & Logarithmic Functions

2.4.2 Solving Equations

2.4.3 Modelling with Functions

2.5 Reciprocal & Rational Functions

2.5.1 Reciprocal & Rational Functions

2.6 Transformations of Graphs

2.6.1 Translations of Graphs

2.6.2 Reflections of Graphs

2.6.3 Stretches Graphs

2.6.4 Composite Transformations of Graphs

2.7 Polynomial Functions

2.7.1 Factor & Remainder Theorem

2.7.2 Polynomial Division

2.7.3 Polynomial Functions

2.7.4 Roots of Polynomials

2.8 Inequalities

2.8.1 Solving Inequalities Graphically

2.8.2 Polynomial Inequalities

2.9 Further Functions & Graphs