Inverse Trigonometric Functions (DP IB Analysis & Approaches (AA)): Revision Note
Inverse trig functions
What are the inverse trig functions?
The inverse trig functions are
They are inverses when the domains are restricted
is the inverse of
when its domain is
e.g.
is the inverse of
when its domain is
e.g.
is the inverse of
when its domain is
e.g.
Examiner Tips and Tricks
Be careful when you are working outside these domains. For example, but
.
What do the graphs of the inverse trig functions look like?
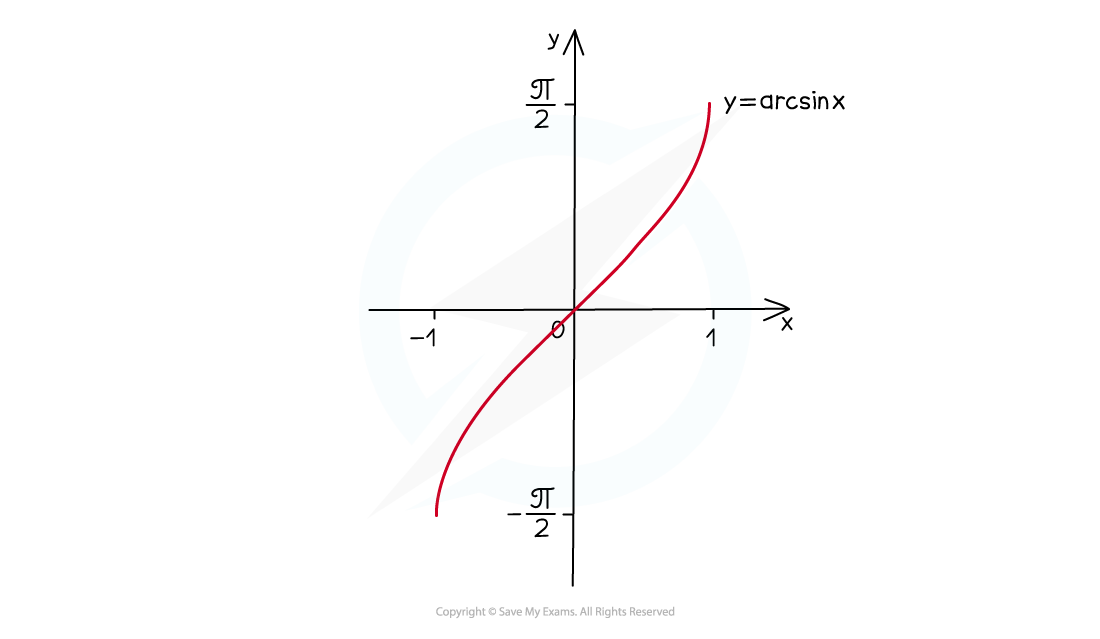
y=arcsinx
is a reflection of
in the line
when its domain is
The domain is
The range is
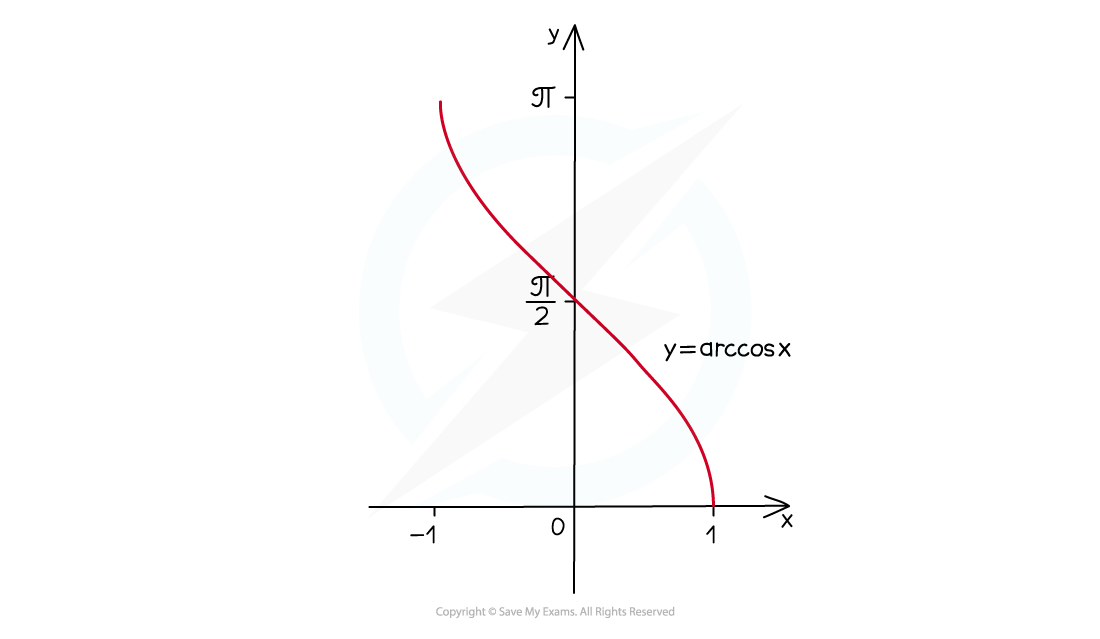
y=arccosx
is a reflection of
in the line
when its domain is
The domain is
The range is
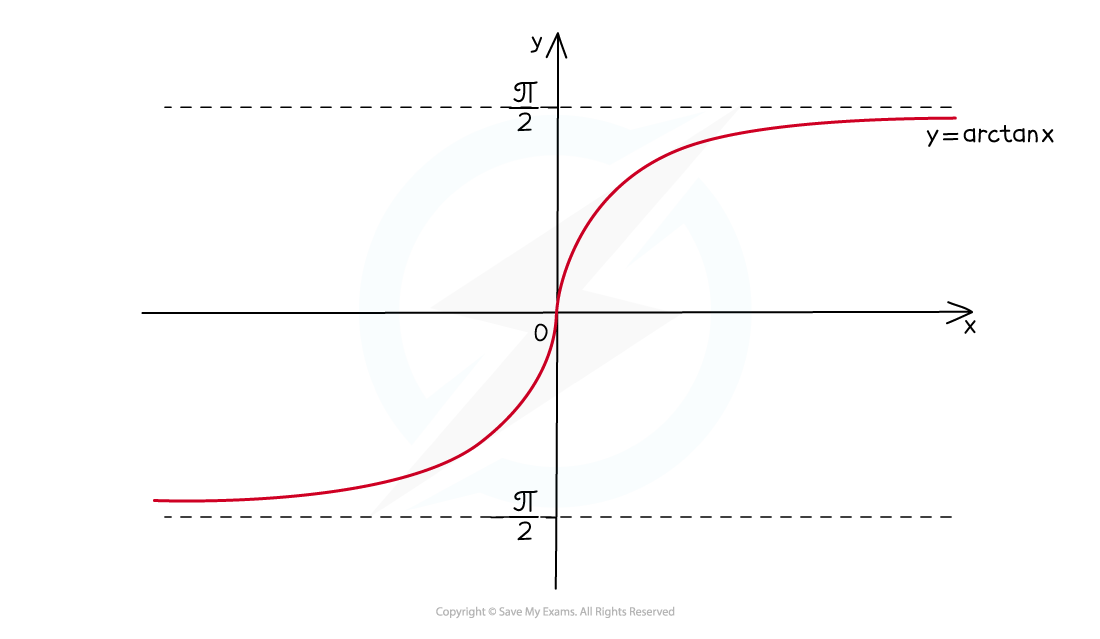
y=arctanx
is a reflection of
in the line
when its domain is
The domain is
The range is
There are horizontal asymptotes at
How can I use inverse trig functions?
You can use the inverse trig functions to help you to solve trig equations
e.g. if
then one solution is
You need to use the symmetries of the trig functions to find the other solutions
You can solve equations involving inverse trig equations
The converse is not always true
You need to use the symmetries to make the value in the trig function be within its restricted domain
e.g.
Therefore
Worked Example
Given that satisfies the equation
where
, state the range of possible values of
.
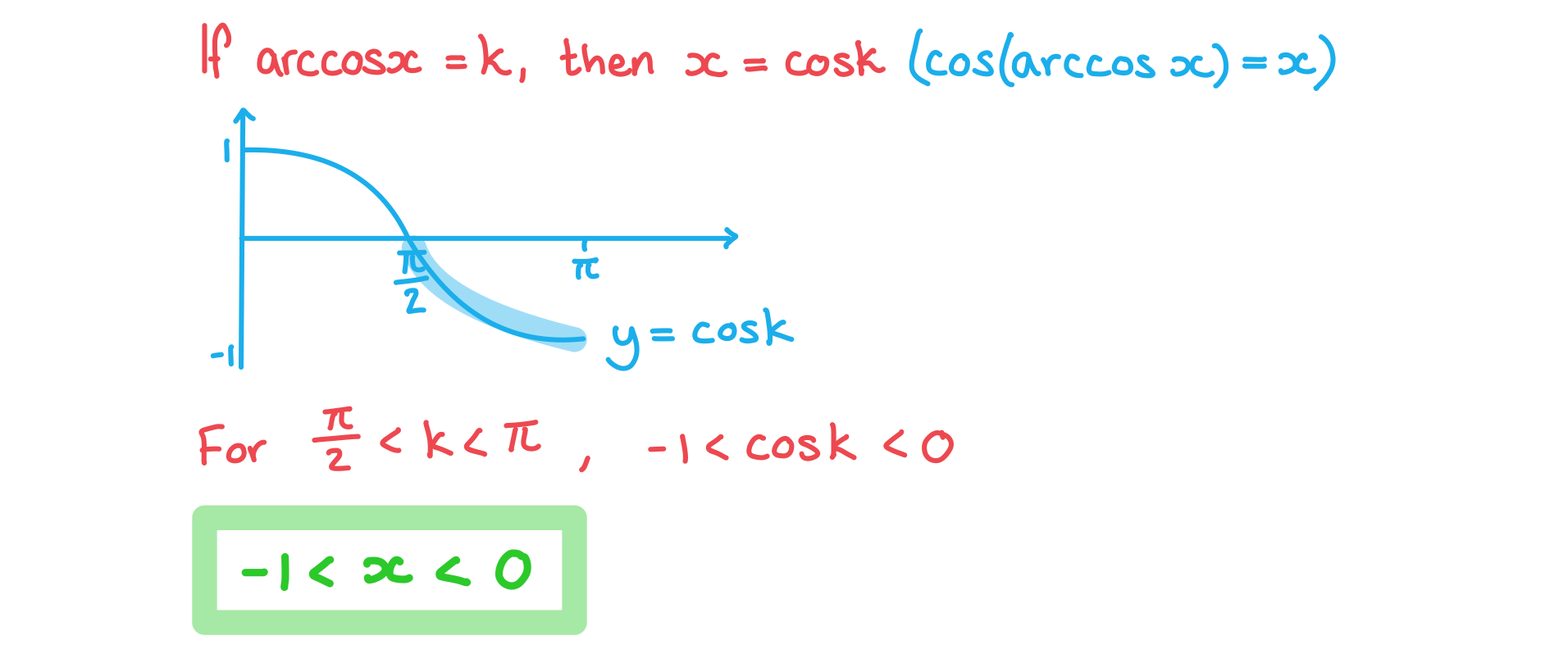

Unlock more, it's free!
Did this page help you?