IBMaths: AA HLDPRevision Notes3. Geometry & Trigonometry3.6 Trigonometric Equations & Identities3.6.6 Quadratic Trigonometric Equations

Quadratic Trigonometric Equations (DP IB Maths: AA HL)

Revision Note

Test yourselfFlashcards
Amber

Author

Amber

Last updated

Did this video help you?

Quadratic Trigonometric Equations

How are quadratic trigonometric equations solved?

  • A quadratic trigonometric equation is one that includes either sin squared space theta, cos squared space theta or tan to the power of 2 space end exponent theta
  • Often the identityspace sin squared space theta plus cos squared space theta equals 1 can be used to rearrange the equation into a form that is possible to solve
    • If the equation involves both sine and cosine then the Pythagorean identity should be used to write the equation in terms of just one of these functions
  • Solve the quadratic equation using your GDC, the quadratic equation or factorisation
    • This can be made easier by changing the function to a single letter
      • Such as changing 2 cos squared space theta minus 3 cos space theta minus 1 equals 0 to 2 c squared minus 3 c minus 1 equals 0
  • A quadratic can give up to two solutions
    • You must consider both solutions to see whether a real value exists
    • Remember that solutions for sin θ = k and cos θ = k only exist for -1 ≤ k ≤ 1  
    • Solutions for tan θ = k exist for all values of k
  • Find all solutions within the given interval
    • There will often be more than two solutions for one quadratic equation
    • The best way to check the number of solutions is to sketch the graph of the function

Examiner Tip

  • Sketch the trig graphs on your exam paper to refer back to as many times as you need to!
  • Be careful to make sure you have found all of the solutions in the given interval, being super-careful if you get a negative solution but have a positive interval

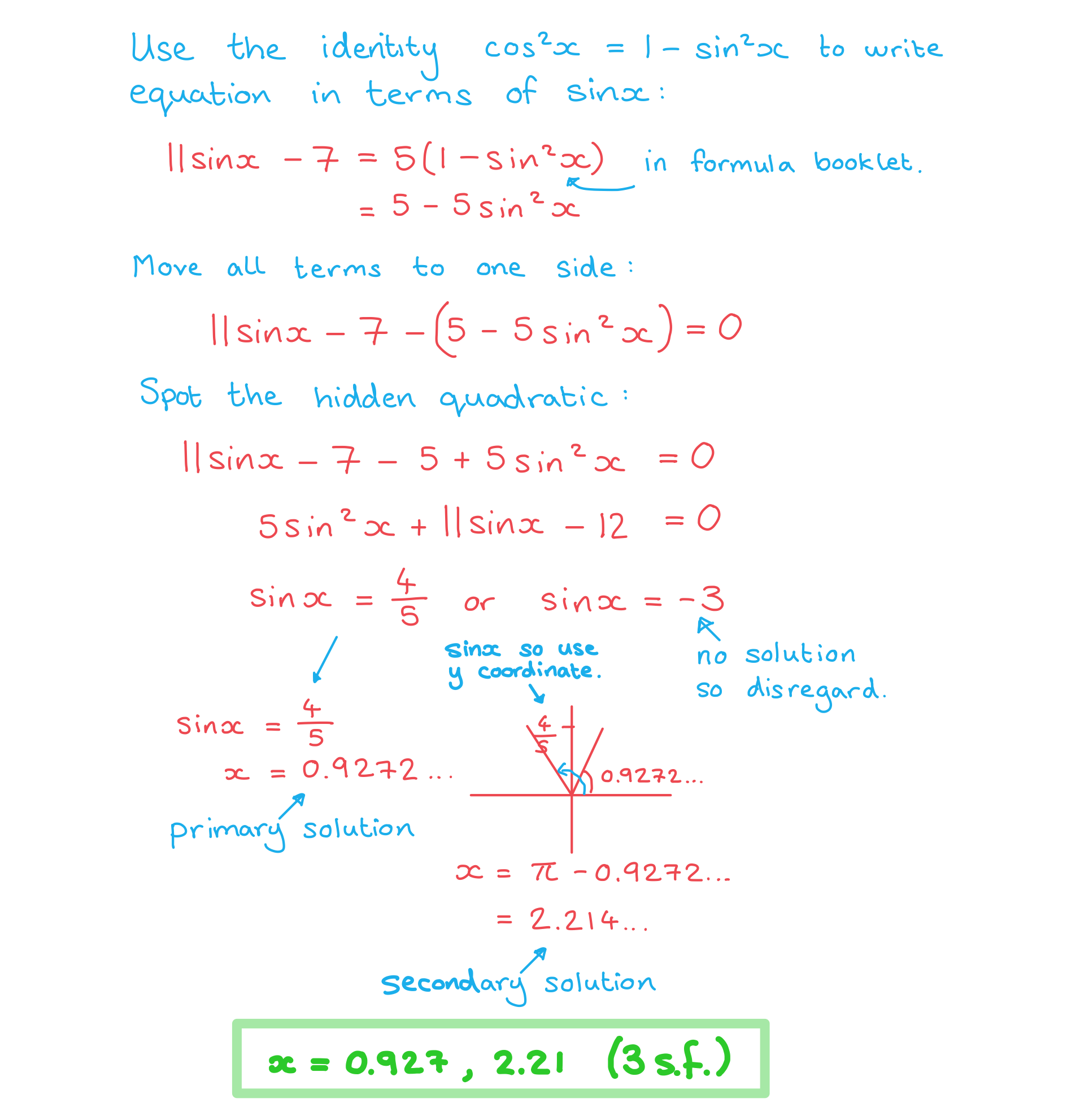
Worked example

Solve the equation 11 sin space x blank – blank 7 blank equals 5 cos squared space x, finding all solutions in the range 0 space less or equal than space x space less or equal than space 2 straight pi.

aa-sl-3-6-5-quadratic-trig-equations-we-solution

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:3.6.5 Linear Trigonometric EquationsNext:3.7.1 Reciprocal Trig Functions
    Amber

    Author: Amber

    Expertise: Maths

    Amber gained a first class degree in Mathematics & Meteorology from the University of Reading before training to become a teacher. She is passionate about teaching, having spent 8 years teaching GCSE and A Level Mathematics both in the UK and internationally. Amber loves creating bright and informative resources to help students reach their potential.