Factor & Remainder Theorem (DP IB Analysis & Approaches (AA)): Revision Note
Factor theorem
What is the factor theorem?
The factor theorem states that for any polynomial function
the following is true
If
is a factor of
then
If
then
is a factor of
For example:
so
is a factor of
so
The factor theorem connects the roots of a polynomial equation and the factors of the polynomial function
Rearrange the root to make it equal to zero to find the factor
e.g.
so
would be the factor
Make the factor equal to zero and solve to find the root
e.g.
so
would be a root
How do I use the factor theorem to find unknown values?
You might be given a polynomial equation with one or two unknown coefficients
e.g.
You will be given one or two factors of the polynomial expression
e.g.
is a factor
To find the value(s) of the unknown(s)
Set the factor equal to zero and solve for
e.g.
Substitute the value of
into the polynomial equation and simplify
e.g.
simplifies to
Solve the resulting equation(s) to find the unknown value(s)
e.g.
How do I use the factor theorem to fully factorise a polynomial?
Find a root of the polynomial equation
Test values of the form
into the polynomial where
is a factor of polynomial's constant term
is a factor of the coefficient of the polynomial's leading term
e.g. for
try
and
Use the factor theorem to identify factors
e.g.
and
Divide the polynomial by each factor
You can divide by one factor at a time
e.g.
e.g.
Or divide by the expansion of the factors
e.g.
Check to see if the new polynomial can be factorised
Examiner Tips and Tricks
Even if you tested all possible roots in the original polynomial, you still need to check if the new polynomial has any roots. It is possible that some of the roots are repeated. For example, the factors might be . Testing the original polynomial will only tell you that
and
are factors, it doesn't tell you how many times they appear.
Repeat the steps until you get a linear expression or a polynomial that cannot be factorised
The factorised form is the product of all the factors you found
e.g.
try
Worked Example
Determine whether is a factor of the following polynomials:
a) .
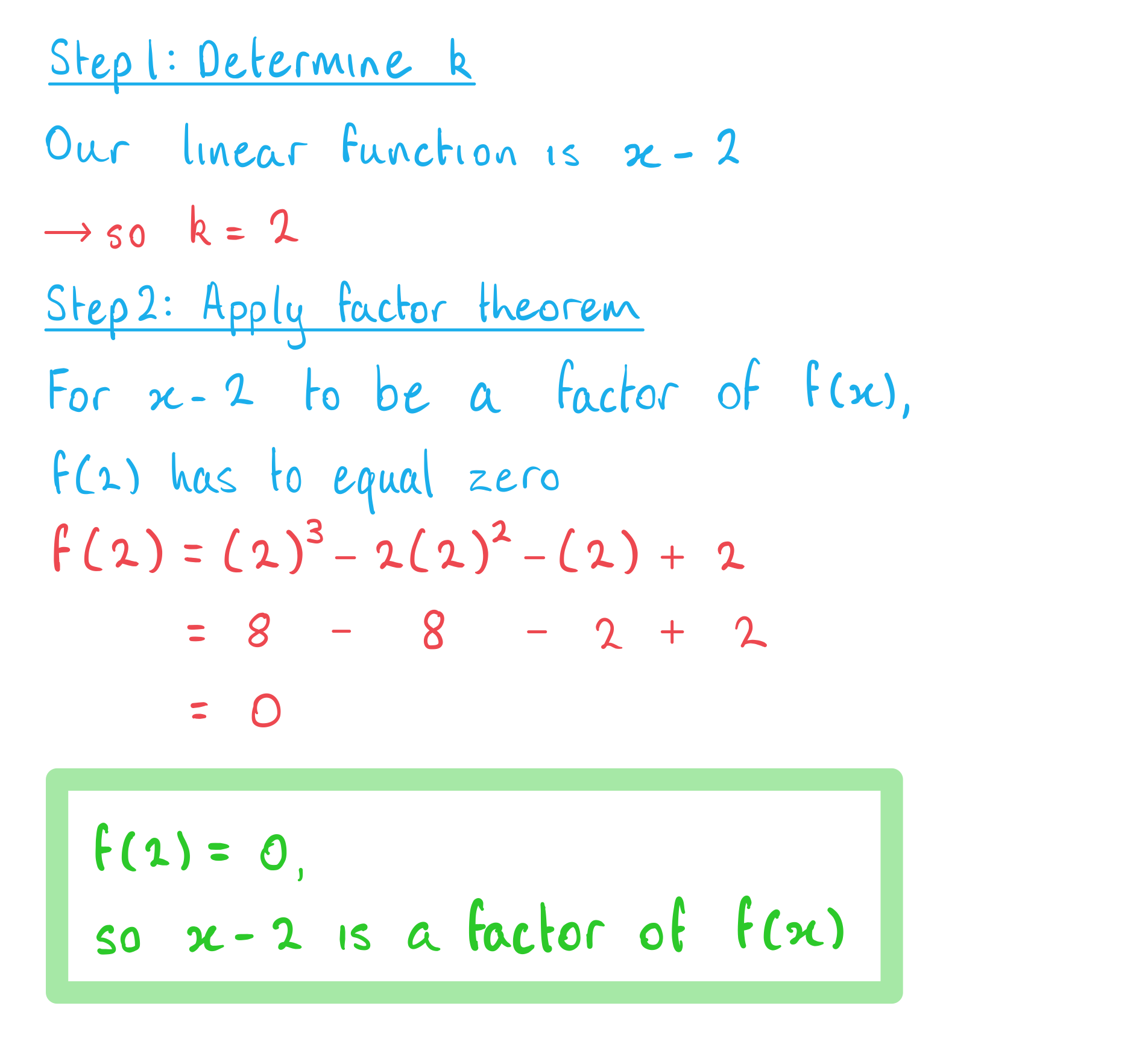
b) .

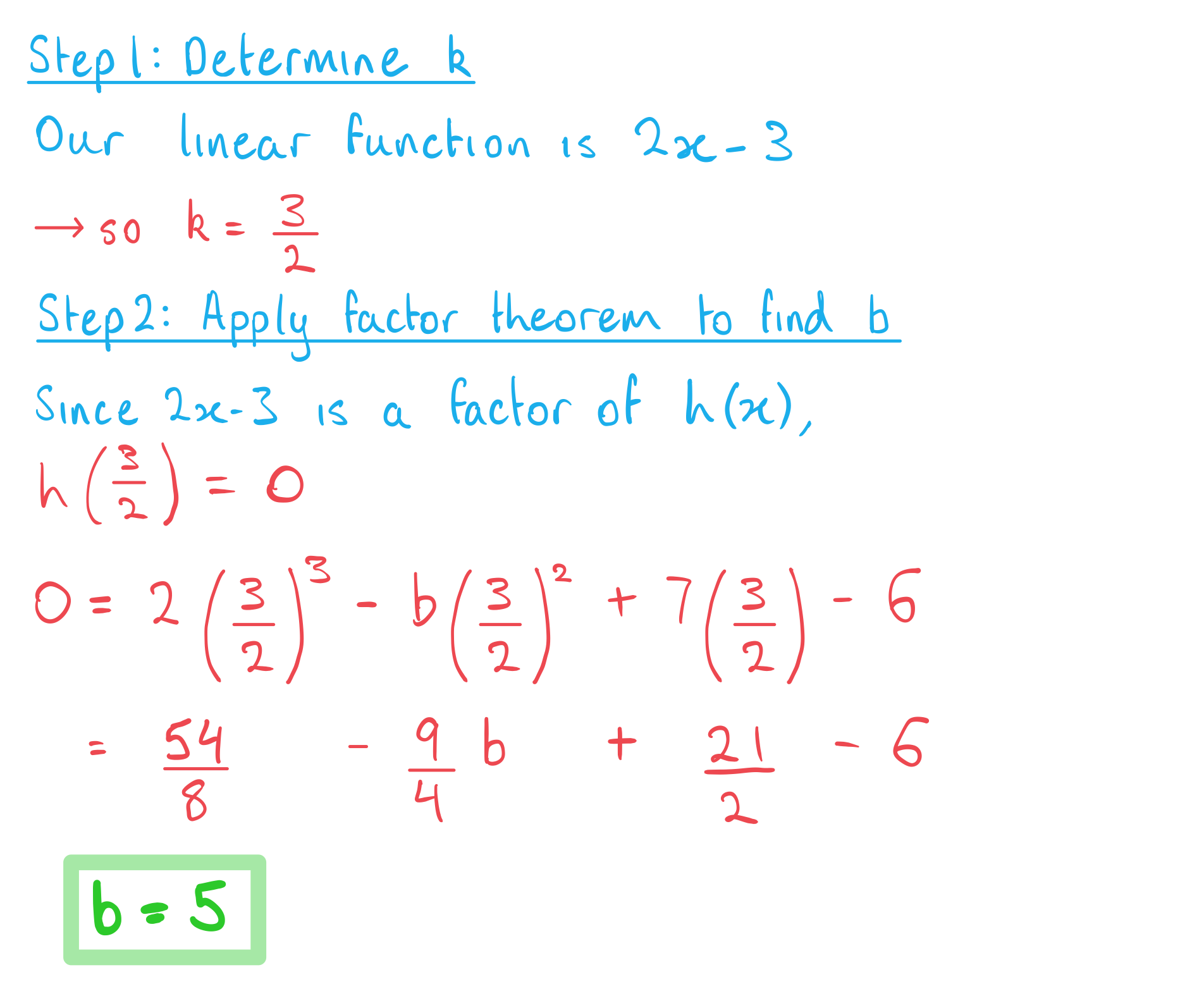
It is given that is a factor of
.
c) Find the value of .
Remainder theorem
What is the remainder theorem?
The remainder theorem states that for any polynomial function
the following is true
The remainder when
is divided by
is equal to
The remainder theorem is used to find the remainder when you divide a polynomial function by a linear function
where
is the quotient polynomial
is the remainder
This means
So
For example:
so the remainder when you divide
by
is 105
The factor theorem is a special case of the remainder theorem when the remainder is zero
How do I use the remainder theorem?
A question will involve a polynomial being divided by a linear expression
You will be asked to find one of the following:
an unknown coefficient of the polynomial
an unknown coefficient of the linear expression
the remainder
Follow these steps for all the cases:
Set the linear expression equal to zero and solve for
e.g.
Substitute the value of
into the polynomial equation
e.g.
simplifies to
This is equal to the remainder
Solve to find any unknowns
Worked Example
Let , find the remainder
when
is divided by:
a) .
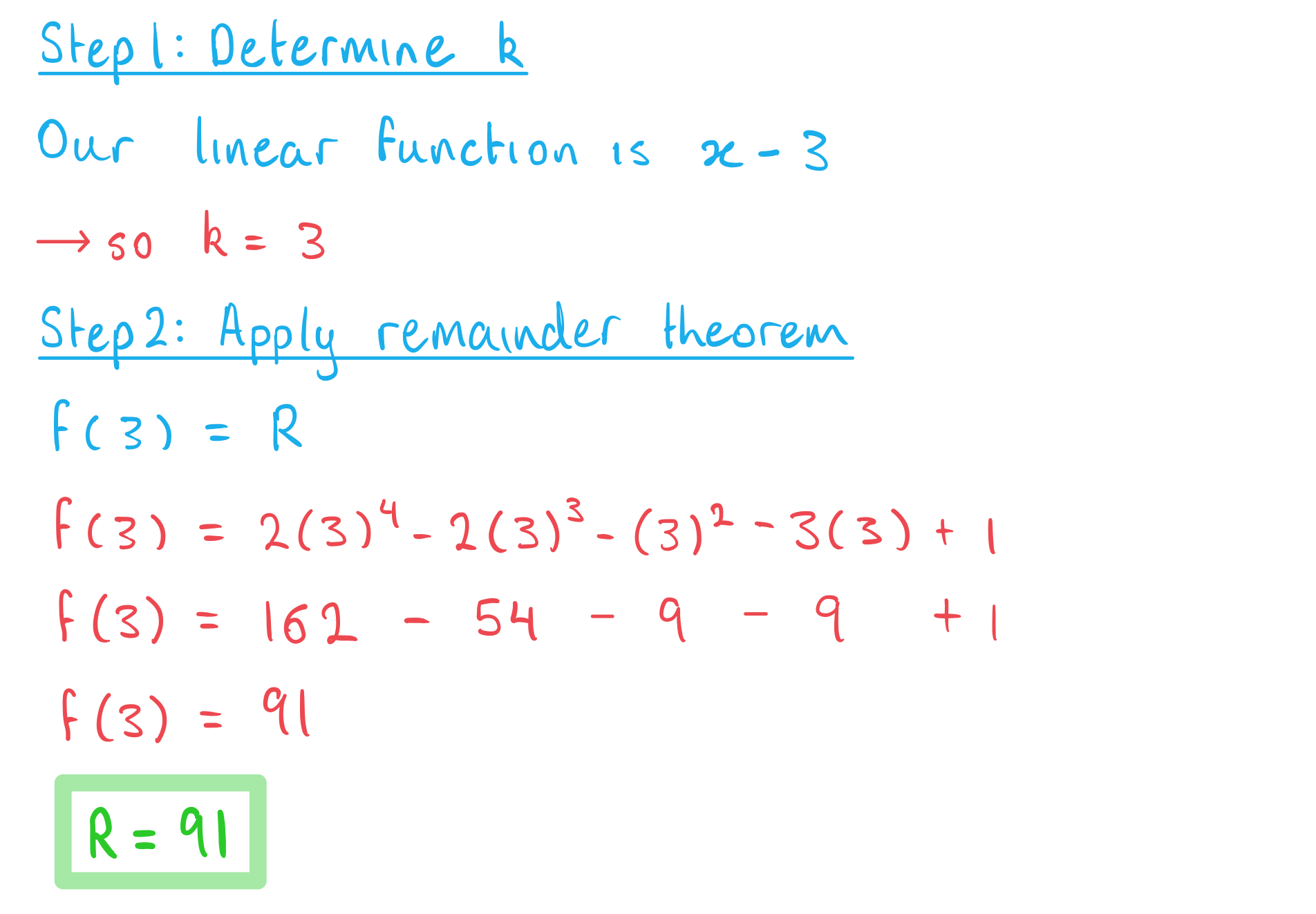
b) .

The remainder when is divided by
is
.
c) Given that , find the value of
.


Unlock more, it's free!
Did this page help you?