Rate Equations (DP IB Chemistry: HL)

• The  rate of reaction can be found by:
  • Measuring the decrease in the concentration of a reactant over time
  • Measuring the increase in the concentration of a product over time
  • The units for rate of reaction are mol dm^-3 s^-1

Rate of Reaction

• A directly proportional relationship between the rate of reaction and concentration of D is observed when the results are plotted on a graph:

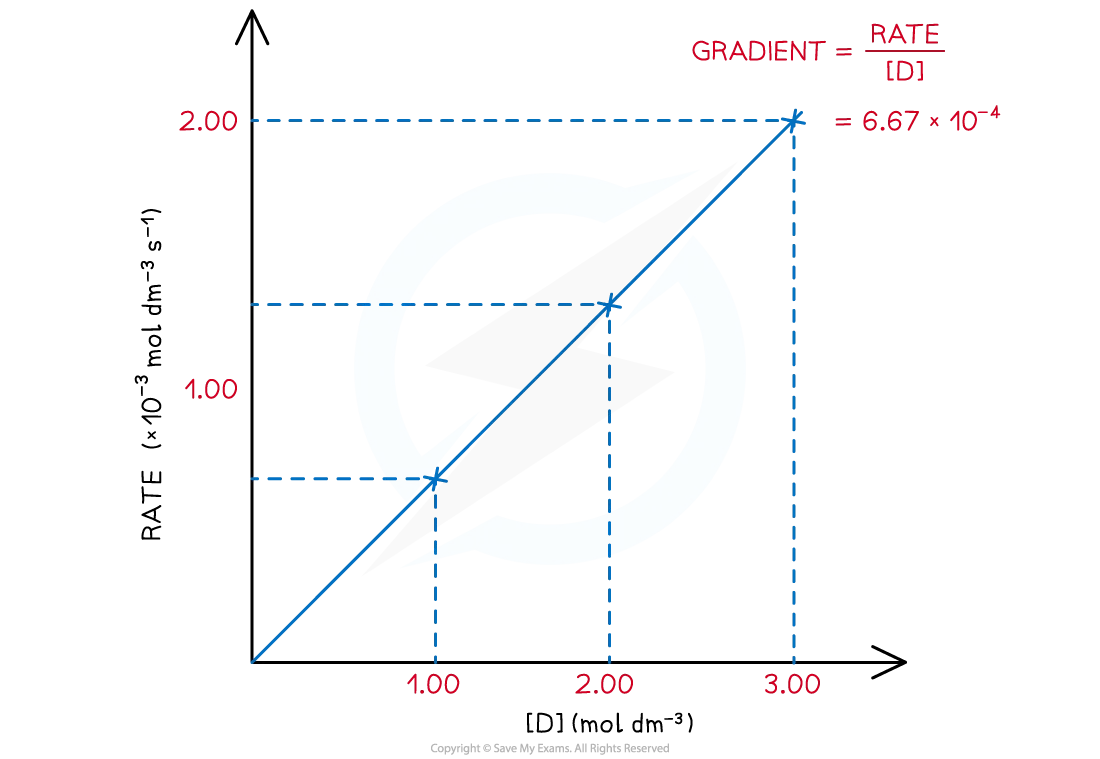
Rate of reaction over various concentrations of D

• This leads to a very common rate expression:

Rate ∝ [D]       or       Rate = k[D]

• This rate expression means that if the concentration of D is doubled, then the rate doubles
• Equally, if the concentration of D halves, then the rate halves

Rate Equations

• The following reaction will be used to discuss rate equations:

A (aq) + B (aq) → C (aq) + D (g) 

• The rate equation for this reaction is:

Rate of reaction = k [A]^m [B]ⁿ

• Rate equations can only be determined experimentally and cannot be found from the stoichiometric equations
• In the above rate equation:
  • [A] and [B] are the concentrations of the reactants
  • m and n are orders with respect to each reactant involved in the reaction

• Products and catalysts may feature in rate equations
• Intermediates do not feature in rate equations

Order of reaction

• The order of a reactant shows how the concentration of a chemical, typically a reactant, affects the rate of reaction
• It is the power to which the concentration of that reactant is raised in the rate equation
• The order can a positive, negative or fractional value
  • Orders that are a fraction suggest that the reaction involves multiple steps

• When the order of reaction with respect to a chemical is 0
  • Changing the concentration of the chemical has no effect on the rate of the reaction
  • Therefore, it is not included in the rate equation

• When the order of reaction with respect to a chemical is 1
  • The concentration of the chemical is directly proportional to the rate of reaction, e.g. doubling the concentration of the chemical doubles the rate of reaction
  • The chemical is included in the rate equation

• When the order of reaction with respect to a chemical is 2
  • The rate is directly proportional to the square of the concentration of that chemical, e.g. doubling the concentration of the chemical increases the rate of reaction by a factor of four
  • The chemical is included in the rate equation (appearing as a squared term)

• The overall order of reaction is the sum of the powers of the reactants in a rate equation

Answers

Answer 1:

• • Dinitrogen pentoxide features in the rate equation, therefore, it cannot be order zero / 0
  • The dinitrogen pentoxide is not raised to a power, which means that it cannot be order 2 / second order
  • Therefore, the order with respect to dinitrogen pentoxide must be order 1 / first order

Answer 2:

• • Since the reaction is first order, the concentration of dinitrogen pentoxide is directly proportional to the rate
  • This means that if the concentration of the dinitrogen pentoxide is tripled, then the rate of reaction will also triple

Answers

Answer 1:

• • All three reactants feature in the rate equation but they are not raised to a power, this means that the order with respect to each reactant is order 1 / first order.
  • The overall order of the reaction is 1 + 1 + 1 = 3 or third order.

Answer 2:

• • Since each reactant is first order, the concentration of each reactant is directly proportional to the effect that it has on rate
  • If the concentration of the bromate ion is doubled, then the rate of reaction will also double
  • If the concentration of the bromide ion is halved then the rate will also halve
  • Therefore, there is no overall effect on the rate of reaction - one change doubles the rate and the other change halves it

Deducing Rate Equations

• The following reaction will be used to deduce the rate equation, using experimental data

(CH₃)₃CBr  +  OH^-  →  (CH₃)₃COH  +  Br^-

Table to show the experimental data of the above reaction

• To derive the rate equation for a reaction, you must first determine all of the orders with respect to each of the reactants
• This can be done using the tabulated data provided
• Take the reactants one at a time and find the order with respect to each reactant individually

1. Identify two experiments where the concentration of one reactant changes, but the concentrations of all other reactants are constant
2. Calculate what happens to the concentration
3. Calculate what happens to the rate of reaction
4. Deduce the order of reaction with respect to that chemical
5. Repeat this for all of the reactants, one at a time, until you have determined the order with respect to all reactants

Order with respect to [(CH₃)₃CBr]

1. In experiments 1 and 2, the concentration of (CH₃)₃CBr changes while the concentration of OH^- remains constant
2. The [(CH₃)₃CBr] has doubled
3. The rate of the reaction has also doubled
4. Therefore, the order with respect to [(CH₃)₃CBr] is 1 (first order)
   • [Change in concentration]^order = change in rate
   • [2]^order = 2
   • [2]¹ = 2

Order with respect to [OH^-]

1. In experiments 1 and 3, the concentration of OH^- changes while the concentration of (CH₃)₃CBr remains constant
2. The [OH^-] has doubled
3. The rate of the reaction has increased by a factor of 4
4. Therefore, the order with respect to [OH^-] is 2 / second order
   • [Change in concentration]^order = change in rate
   • [2]^order = 4
   • [2]² = 2

Building the rate equation

• Once  the order with respect to all of the reactants is known the rate equation can be constructed
  • Zero order reactants are not included in the rate equation
  • First order reactants are included in the rate equation - they do not require a power
  • Second order reactants are included in the rate equation - they are raised to the power of 2

• So, for this reaction the rate equation will be:

Rate = k [(CH₃)₃CBr] [OH^-]²