Revision NotesExam QuestionsFlashcardsPast Papers
IBChemistryDPHLRevision NotesWhat are the Mechanisms of Chemical Change?Proton Transfer ReactionsAcid & Base Dissociation Constants (HL)

Acid & Base Dissociation Constants (HL) (DP IB Chemistry)

Revision Note

Philippa Platt

Last updated

Acid & Base Dissociation Constants

Weak acids

  • A weak acid is an acid that partially (or incompletely) dissociates in aqueous solutions

    • For example, most carboxylic acids (e.g. ethanoic acid), HCN (hydrocyanic acid), H2S (hydrogen sulfide) and H2CO3 (carbonic acid)

  • In general, the following equilibrium is established:

HA (aq) + H2O (l) ⇌  A- (aq) + H3O+ (aq)

OR

HA (aq) ⇌  A- (aq) + H+ (aq)

  • At equilibrium, the majority of HA molecules remain unreacted

  • The position of the equilibrium is more towards the left and an equilibrium is established

  • As this is an equilibrium, we can write an equilibrium constant expression for the reaction

  • This constant is called the acid dissociation constant, Ka

K subscript straight a space equals space fraction numerator stretchy left square bracket straight A to the power of minus stretchy right square bracket open square brackets H to the power of plus close square brackets over denominator stretchy left square bracket HA stretchy right square bracket end fraction

  • Carboxylic acids are weak acids

    • For example, propanoic acid, CH3CH2COOH (aq), dissociates according to the following equation which leads to the Ka expression for propanoic acid:

CH3CH2COOH (aq) + H2O (l) ⇌ CH3CH2COO– (aq) + H3O+ (aq)

OR

CH3CH2COOH (aq) ⇌ CH3CH2COO– (aq) + H+ (aq)

  • The acid dissociation constant expressions for propanoic acid:

    • K subscript straight a space equals space fraction numerator stretchy left square bracket CH subscript 3 CH subscript 2 COO to the power of minus stretchy right square bracket stretchy left square bracket straight H to the power of plus stretchy right square bracket over denominator stretchy left square bracket CH subscript 3 CH subscript 2 COOH stretchy right square bracket end fraction

  • Values of Ka are very small

    • For example, Ka  for propanoic acid = 1.34 x 10-5 

    • When writing the equilibrium expression for weak acids, we assume that the concentration of H+ (aq)  due to the ionisation of water is negligible

Weak bases

  • A weak base will also ionise in water and we can represent this with the base dissociation constant, Kb

  • In general, the equilibrium established is:

B (aq) + H2O (l) ⇌  BH+ (aq) + OH- (aq)

  • The base dissociation constant expression is:

K subscript straight b equals space fraction numerator open square brackets BH to the power of plus close square brackets open square brackets OH to the power of minus close square brackets over denominator open square brackets straight B close square brackets end fraction

  • Amines are weak bases

    • For example, 1-phenylmethanamine, C6H5CH2NH2 (aq), dissociates according to the following equation which leads to the Ka expression for 1-phenylmethanamine:

C6H5CH2NH2 (aq) + H2O (l) ⇌ C6H5CH2NH3+ (aq) + OH- (aq)

  • Base dissociation constant expression for 1-phenylmethanamine

    • K subscript straight b equals space fraction numerator stretchy left square bracket CH subscript 5 CH subscript 2 NH subscript 3 to the power of plus stretchy right square bracket stretchy left square bracket OH to the power of minus stretchy right square bracket over denominator stretchy left square bracket straight C subscript 6 straight H subscript 5 CH subscript 2 NH subscript 2 stretchy right square bracket end fraction

pKa and pKb 

  • The range of values of Ka and Kb is very wide

  • For weak acids, the values themselves are very small numbers

Table of Ka values

Acid

Ka

pKa

Methanoic acid, HCOOH

1.77 x 10–4

3.75

Ethanoic acid, CH3COOH

1.74 x 10–5

4.75

Benzoic acid, C6H5COOH

6.46 x 10–5

4.18

Carbonic acid, H2CO3

4.30 x 10-5

6.36

  • For this reason, it is easier to work with another term called pKa for acids or pKb for bases

  • In order to convert the values we need to apply the following calculations:

pKa = -logKa                 Ka= 10-pKa

pKb = -logKb                 Kb= 10-pKb

  • The range of pKa values for most weak acids lies between 3 and 7

Relative Strengths of Acids and Bases

  • The larger the Ka value, the stronger the acid

  • The larger the pKa value, the weaker the acid

  • The larger the Kb value, the stronger the base

  • The larger the pKb value, the weaker the base

Diagram showing the relationship between strong and weak acids / bases

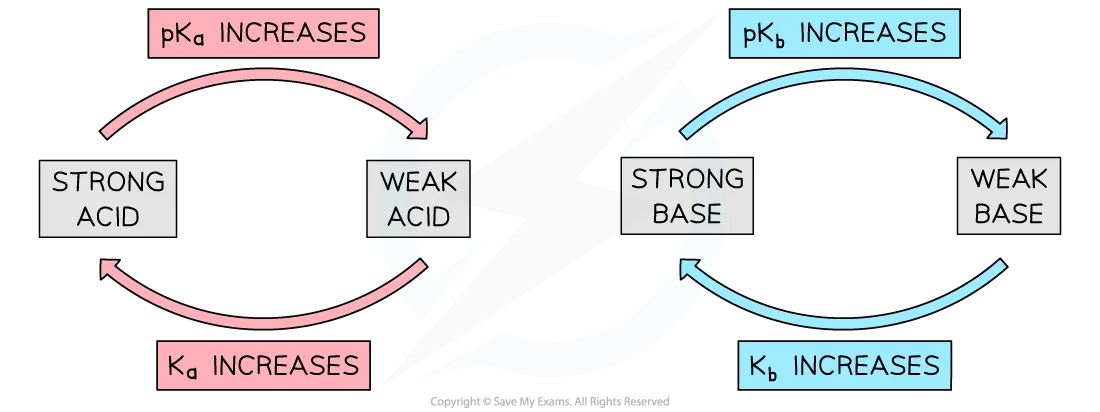
Diagram showing the relative strengths of acids and bases in terms of pKa and pKb

pKa and pKb tell us the relative strengths of acids and bases 

  • In all aqueous solutions, an equilibrium exists in water where a few water molecules dissociate into protons and hydroxide ions

  • We can derive an equilibrium constant for the reaction:

H2O (l) ⇌ H+ (aq) + OH- (aq)

  • The concentration of water is constant, so the expression for Kw is:

Kw = [H+][OH-]

  • This is a specific equilibrium constant called the ion product for water

  • The product of the two ion concentrations is 1.00 x 10-14  at 298 K

  • For conjugate acid-base pairs, Ka and Kb are related to Kw

Ka x Kb = Kw

  • The conjugate base of ethanoic acid is the ethanoate ion, CH3COO– (aq) 

   CH3COOH (aq)  ⇌ CH3COO– (aq) + H+ (aq)

acid                 conjugate base

  • We can then put this into the Ka expression

    • K subscript straight a space equals space fraction numerator stretchy left square bracket CH subscript 3 COO to the power of minus stretchy right square bracket stretchy left square bracket straight H to the power of plus stretchy right square bracket over denominator stretchy left square bracket CH subscript 3 COOH stretchy right square bracket end fraction

  • The ethanoate ion will react with water according to the following equation

CH3COO– (aq) + H2O (l) ⇌ CH3COOH (aq) + OH- (aq)

  • We can then put this into the Kb expression

    • K subscript straight b space equals space fraction numerator stretchy left square bracket CH subscript 3 COOH stretchy right square bracket open square brackets OH to the power of minus close square brackets over denominator open square brackets CH subscript 3 COO to the power of minus close square brackets end fraction

  • Now, these two expressions can be combined, which corresponds to

    • Ka x Kb = Kw

    • Ka x Kb = 10-14

  • Or we could say that

    • pKa + pKb = pKw

    • pKa + pKb = 14

    • This makes the numbers much more easy to deal with as using Ka Kb = 10-14 will give very small numbers

  • Combining the Ka and Kb expressions:

    • K subscript straight a space cross times space space K subscript straight b space equals space fraction numerator open square brackets CH subscript 3 COO to the power of minus close square brackets open square brackets straight H to the power of plus close square brackets over denominator open square brackets CH subscript 3 COOH close square brackets end fraction cross times fraction numerator open square brackets CH subscript 3 COOH close square brackets open square brackets OH to the power of minus close square brackets over denominator open square brackets CH subscript 3 COO to the power of minus close square brackets end fraction K subscript straight a space cross times space K subscript straight b space equals open square brackets straight H to the power of plus close square brackets open square brackets OH to the power of minus close square brackets space equals space K subscript straight w

  • Or rearranging these:

    • K subscript straight a space equals space K subscript straight w over K subscript straight b

    • K subscript straight b space equals space K subscript straight w over K subscript straight a

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourselfFlashcards

    Did this page help you?

    Previous:The pOH Scale (HL)Next:Solving Acid-Base Dissociation Problems (HL)
    Philippa Platt

    Author: Philippa Platt

    Expertise: Chemistry

    Philippa has worked as a GCSE and A level chemistry teacher and tutor for over thirteen years. She studied chemistry and sport science at Loughborough University graduating in 2007 having also completed her PGCE in science. Throughout her time as a teacher she was incharge of a boarding house for five years and coached many teams in a variety of sports. When not producing resources with the chemistry team, Philippa enjoys being active outside with her young family and is a very keen gardener