Statistical Testing (AQA AS Psychology)

Probability & significance

• Psychologists are interested in finding out if the results of their studies show real differences or correlations, or if the results are due to chance factors

• To determine whether results are significant and not due to chance factors, researchers use a measure of the level of significance 

• Researchers must decide how large an effect or relationship is required to conclude that the observed result is unlikely to be due to chance

  • This decision is reflected in the level of significance applied to the data

• The level of significance is expressed as a decimal value where 'p' stands for the probability that chance factors are responsible for the results

• For most purposes in psychology, the 5% level of significance is appropriate which is expressed as p < 0.05 (i.e. the probability of chance factors producing the observed result is less than or equal to 5%)

• The research will then use statistical tables to find the critical value which will determine whether or not they can reject the null hypothesis

Use of statistical tables & the critical value

• Once the researcher has conducted their research and carried out a statistical test they then have an observed value which is used to determine whether the results of their study are significant  

• The observed value needs to be compared to the critical value in the statistical table

• Each statistical test has its own critical values table

  • The critical values table for the Mann-Whitney test is different to the table for the Wilcoxon test

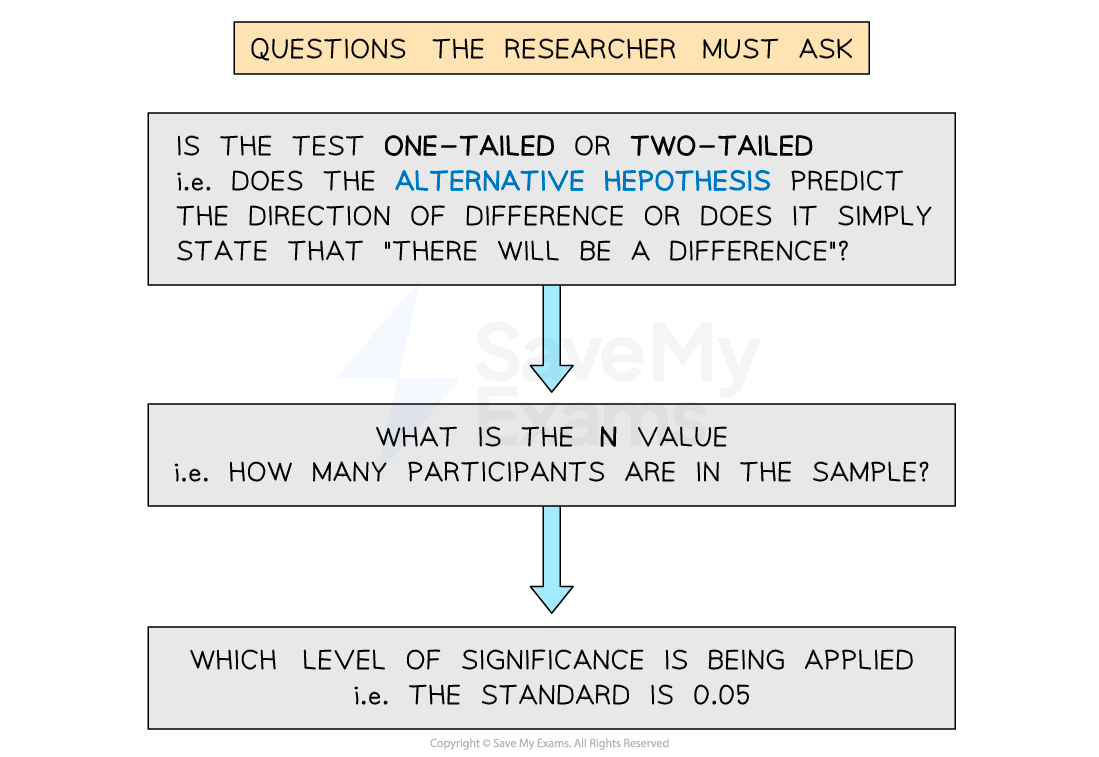
• To find out whether or not the observed value is significant the researcher must ask the following questions which will help them to use the critical values table properly:

  • Is it a test of difference (i.e. a lab experiment) or a test of association (i.e. a correlation)?

  • Is the experimental design independent measures, repeated measures or matched pairs (tests of difference only)?

  • Is the data nominal, ordinal or interval?

• Once the above questions have been answered, the researcher consults the critical values tables to find the value at which a significant result can be claimed e.g.

  • using the probability level of 0.05, if the observed value is 15 and the critical value is 17 then (for some of the statistical tests) the researcher can claim a significant result

    • This is due to the observed value being lower than the critical value at the 0.05 level (for some tests the observed value must be higher than the critical value)

• If significance is found then the researcher can reject the null hypothesis and accept their alternative hypothesis; the results are not due to chance