Progressive Waves (Cambridge (CIE) AS Physics)

Wave motion

• Progressive waves transfer energy without transferring matter; they move through a medium or the vacuum of space

• This is opposed to standing waves which have no net energy transfer and oscillate with fixed nodes and antinodes

• Energy is transferred through moving oscillations or vibrations.

  • These can be seen in vibrations of ropes or springs

Vibrations of ropes or springs

Waves can be shown through vibrations in ropes or springs

• The oscillations / vibrations can be perpendicular or parallel to the direction of wave travel:

  • When they are perpendicular, they are transverse waves

  • When they are parallel, they are longitudinal waves

Ripple tanks

• Ripple tanks may be used to demonstrate the wave properties of reflection, refraction and diffraction.

  • The paddle produces a combination of transverse and longitudinal waves

A ripple tank

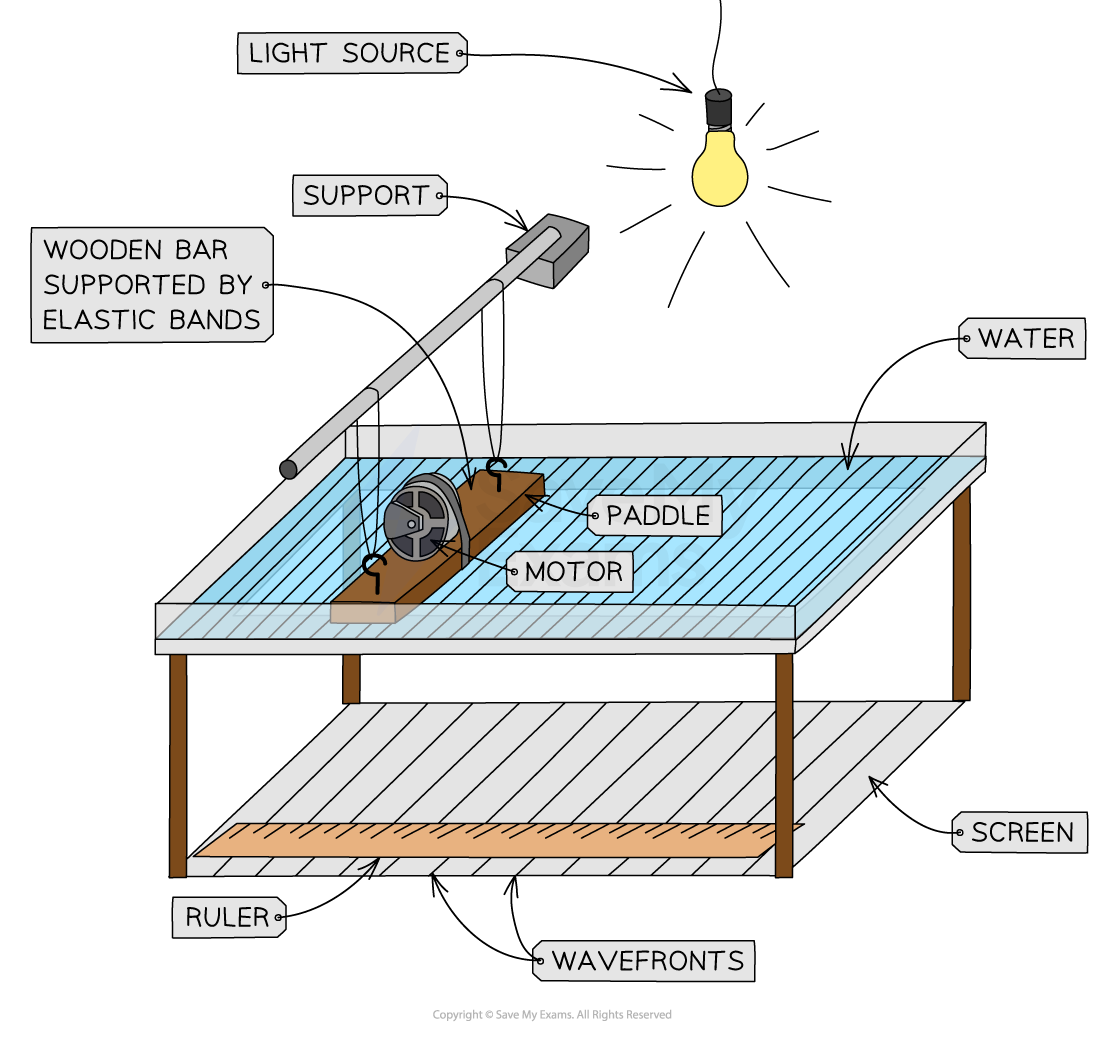
Wave effects can be demonstrated using a ripple tank - the taller regions of water block more light, allowing the wavefronts to be seen using shadows 

General wave properties

• Displacement (x) of a wave is the distance from its equilibrium position. It is a vector quantity; it can be positive or negative

• Amplitude (A) is the maximum displacement of a particle in the wave from its equilibrium position

• Wavelength (λ) is the distance between points on successive oscillations of the wave that are in phase

  • These are all measured in metres (m)

 Graph showing displacement of a wave against distance

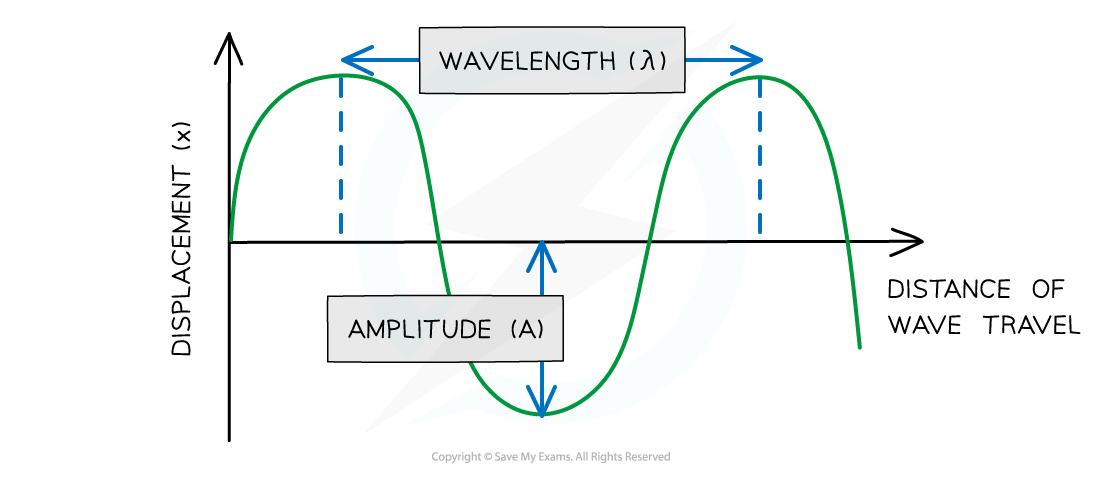
Wavelength is the distance between two identical points on the wave and amplitude is maximum displacement from equilibrium position

• Period (T) or time period, is the time taken for one complete oscillation or cycle of the wave

  • Measured in seconds (s)

Graph showing displacement of a wave against time 

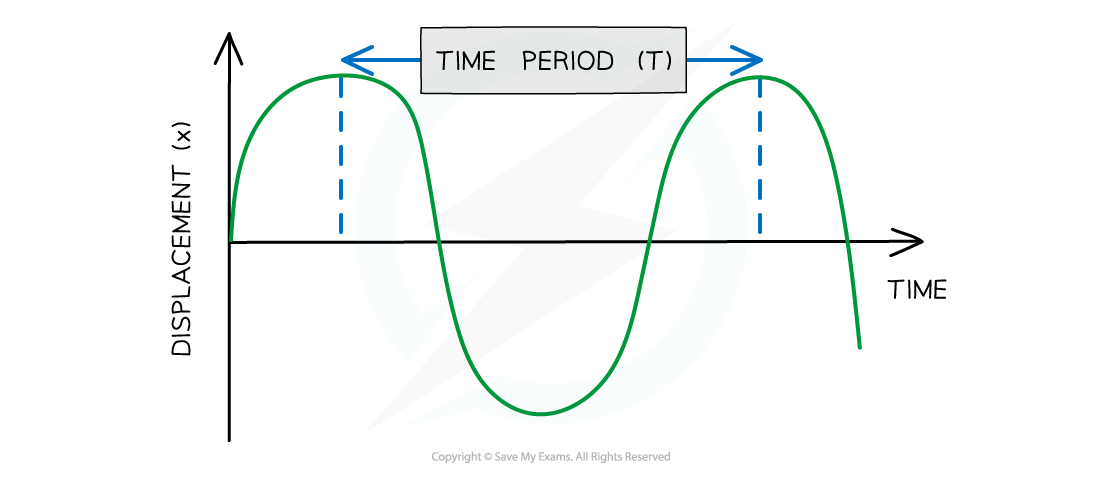
When the x axis is time, the separation between two identical points on the wave is the period of the wave, not the wavelength. Look out for the quantity on the x axis.

• Frequency f is the number of complete oscillations per unit time

  • Measured in Hertz (Hz) or s^-1

• Where T is the time period of the oscillation, measured in seconds (s)

• Speed v is the distance travelled by the wave per unit time

  • Measured in metres per second (m s^-1)

Phase difference

• The phase difference tells us how much a point or a wave is in front or behind another

  • When the crests or troughs are aligned, the waves are in phase

  • When the crest of one wave aligns with the trough of another, they are in antiphase

  • This can be found from the relative positions of the crests or troughs of two different waves of the same frequency

• The diagram below shows that

  • the green wave leads the purple wave by ¼ λ

  • the purple wave is said to lag behind the green wave by ¼ λ

Displacement time graph shows phase difference

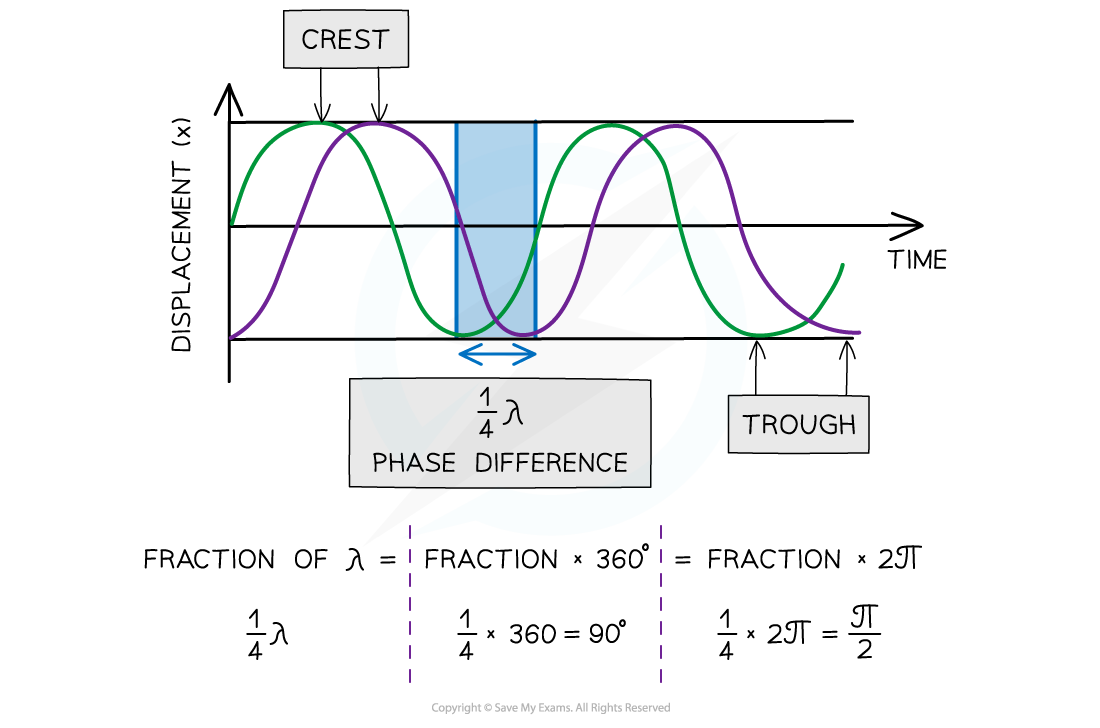
Two waves ¼ λ out of phase

• Phase difference is measured in fractions of a wavelength, degrees or radians

• The phase difference between two points is:

  • In phase is 360^o or 2π radians

  • In anti-phase is 180^o or π radians

Wave energy

• Waves that transfer energy are known as progressive waves

• Progressive waves transfer energy between points, without transferring matter

• When a progressive wave travels between two points, no matter actually travels with it

  • The points on the wave simply vibrate back and forth about fixed positions

• Waves that do not transfer energy are known as stationary waves