Elastic Strain Energy (AQA AS Physics): Revision Note
Exam code: 7407
Elastic Strain Energy
Work has to be done to stretch a material
Before a material reaches its elastic limit (whilst it obeys Hooke's Law), all the work is done is stored as elastic strain energy
The work done, or the elastic strain energy is the area under the force-extension graph

Work done is the area under the force-extension graph
This is true for whether the material obeys Hooke’s law or not
For the region where the material obeys Hooke’s law, the work done is the area of a right-angled triangle under the graph
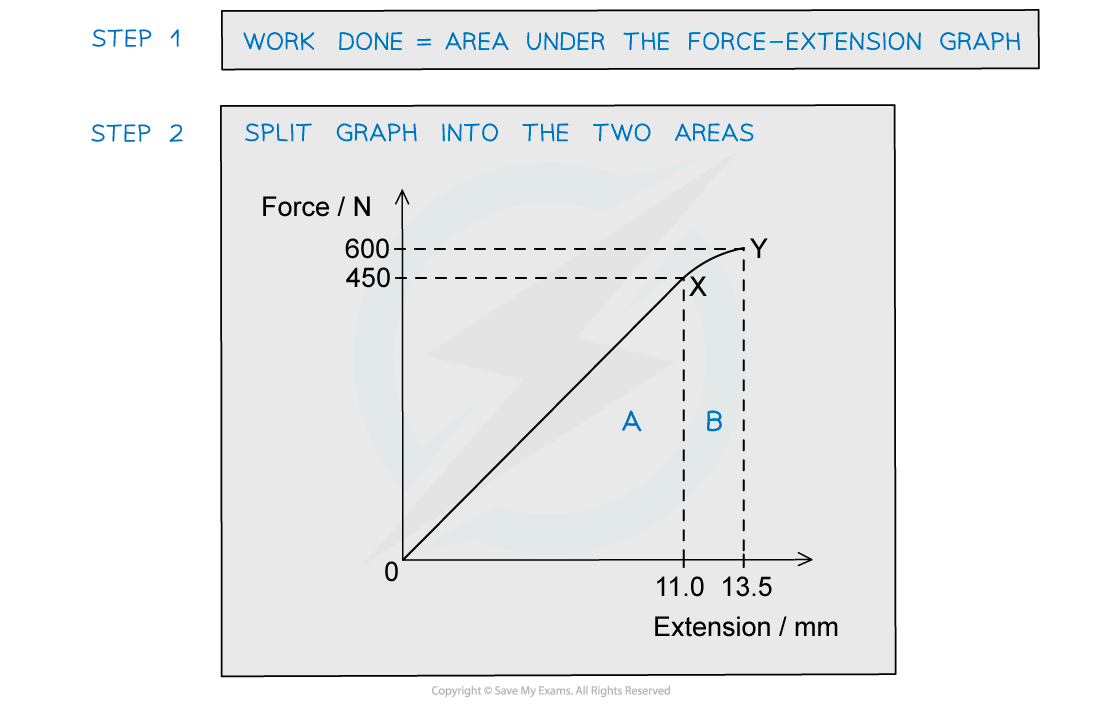
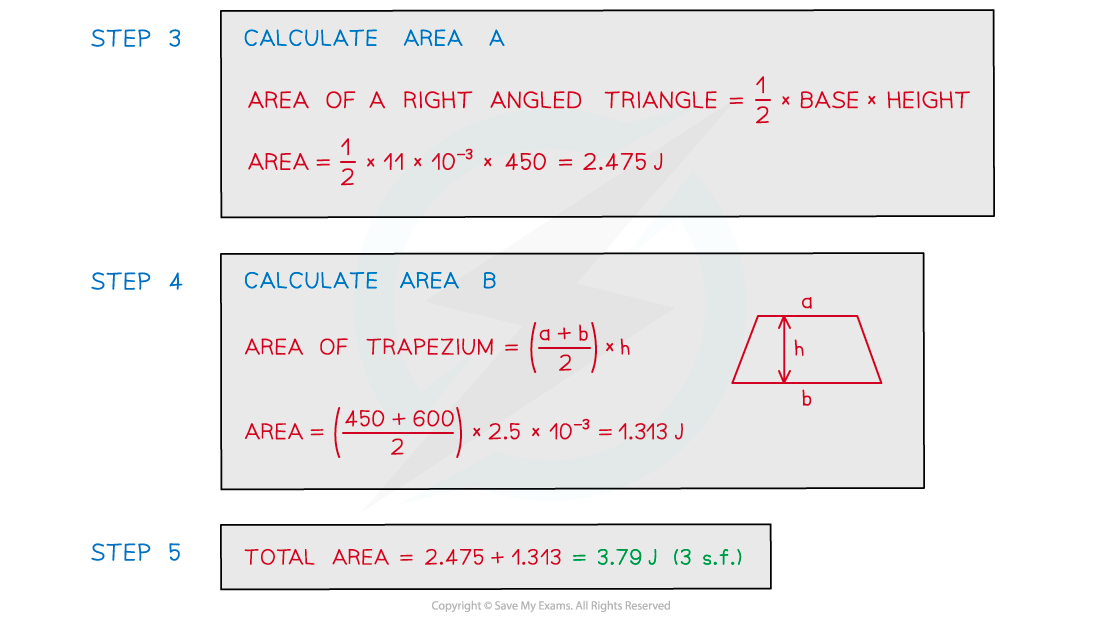
For the region where the material doesn’t obey Hooke’s law, the area is the full region under the graph. To calculate this area, split the graph into separate segments and add up the individual areas of each
When a material does obey Hooke's law, the elastic strain energy, E can be calculated using:
Where:
E = elastic strain energy (or work done) (J)
F = average force (N)
ΔL = extension (m)
This equation is equivalent to the area of a right-angled triangle under the force-extension graph
Using Hooke's law
, the elastic strain energy can also be written as:
Where:
k = spring constant (N m–1)
Breaking Stress
As greater force is applied on a material, the stress on it increases
The breaking stress is the maximum stress a material can stand before it fractures (breaks)
A material with high breaking stress is considered ductile, which means it can extend more before breaking because of plastic deformation
A common example of this is copper, as well as being a good electrical conductor, copper is ductile so it is a suitable material for making wires
The ultimate tensile stress (UTS) is sometimes also marked on a stress-strain graph
This is the maximum stress that the material can withstand
The UTS and breaking stress can depend on the condition of the material such as its temperature
This is very important for engineers when considering materials for a particular structure
The material might need to stand extreme temperatures and loads which are taken into consideration

The ultimate breaking stress is the point on the stress-strain graph of maximum stress. The breaking point is where the material fractures
Worked Example
The graph shows the behaviour of a sample of a metal when it is stretched until it starts to undergo plastic deformation.

What is the total work done in stretching the sample from zero to 13.5 mm extension?
Simplify the calculation by treating the curve XY as a straight line.
Answer:
Worked Example
A spring is extended with varying forces; the graph below shows the results.

What is the energy stored in the spring when the extension is 40 mm?
Answer:
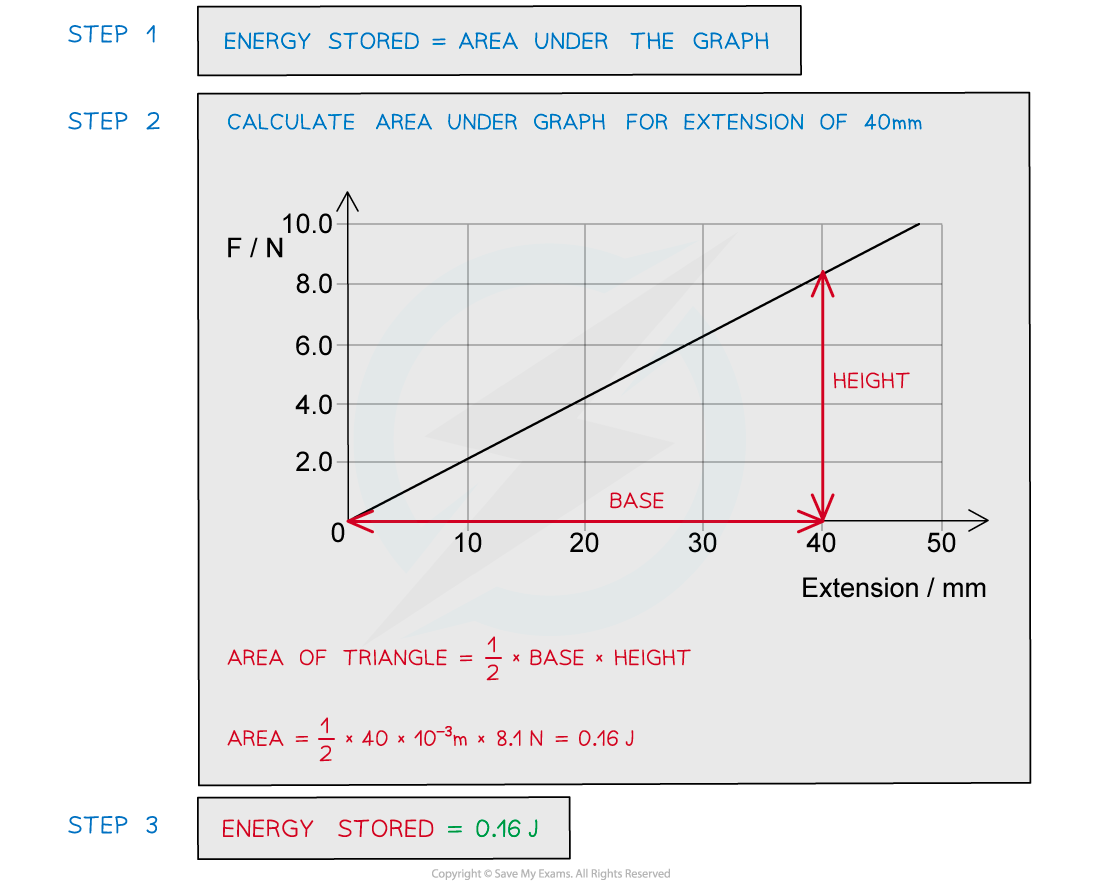
Spring Energy
When a vertical spring is extended and contracted, its energy is converted into other forms
Although the total energy of the spring will remain constant, it will have changing amounts of:
Elastic potential energy (EPE)
Kinetic energy (KE)
Gravitational potential energy (GPE)
When a vertical mass is hanging on a spring and it moves up and down, its energy will convert between the three in various amounts
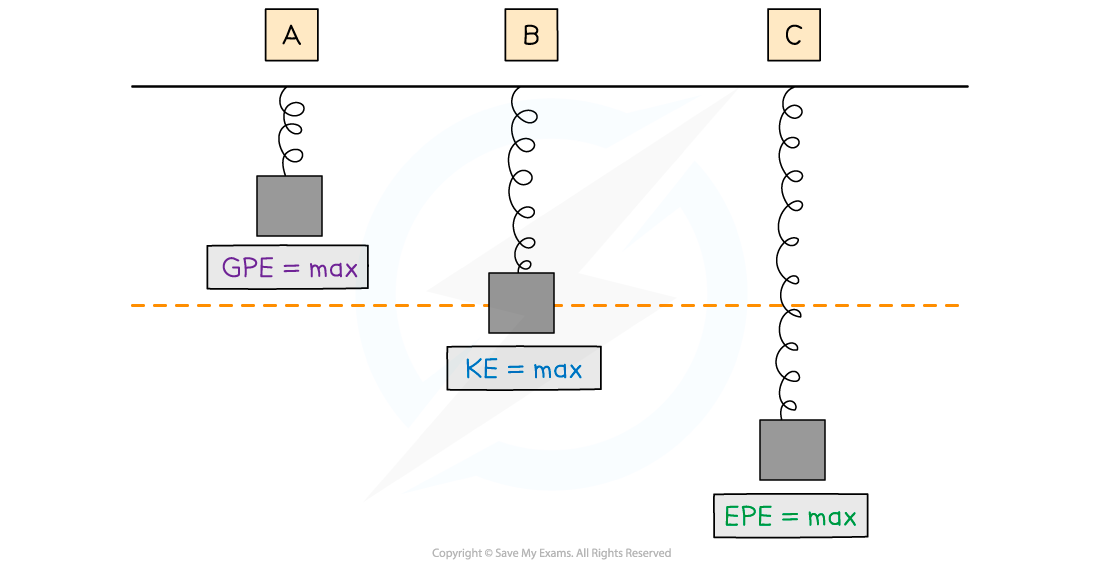
At position A:
The spring has some EPE since it is slightly compressed
Its KE is 0 since it is stationary
Its GPE is at a maximum because the mass is at its highest point
At position B:
The spring has some EPE since it is slightly stretched
Its KE is at a maximum as it passes through the equilibrium position at its maximum speed
It has some GPE since the mass is still above the ground
At position C:
The spring has its maximum EPE because it is at its maximum extension
Its KE is 0 since it is stationary
Its GPE is at a minimum because it is at its lowest point above the Earth's surface
For a horizontal mass on a spring system, there is no gravitational potential energy to consider. The spring only converts between kinetic and elastic potential energy
Examiner Tips and Tricks
It is important to remember at which position the mass has the greatest amount of which type of energy (and which type of energy is 0).

Unlock more, it's free!
Did this page help you?