Expanding Brackets (AQA AS Maths: Pure)

What do I need to know about expanding brackets?

• To expand brackets, the rule is that each term in one set of brackets must be multiplied by each term in the other set of brackets

 

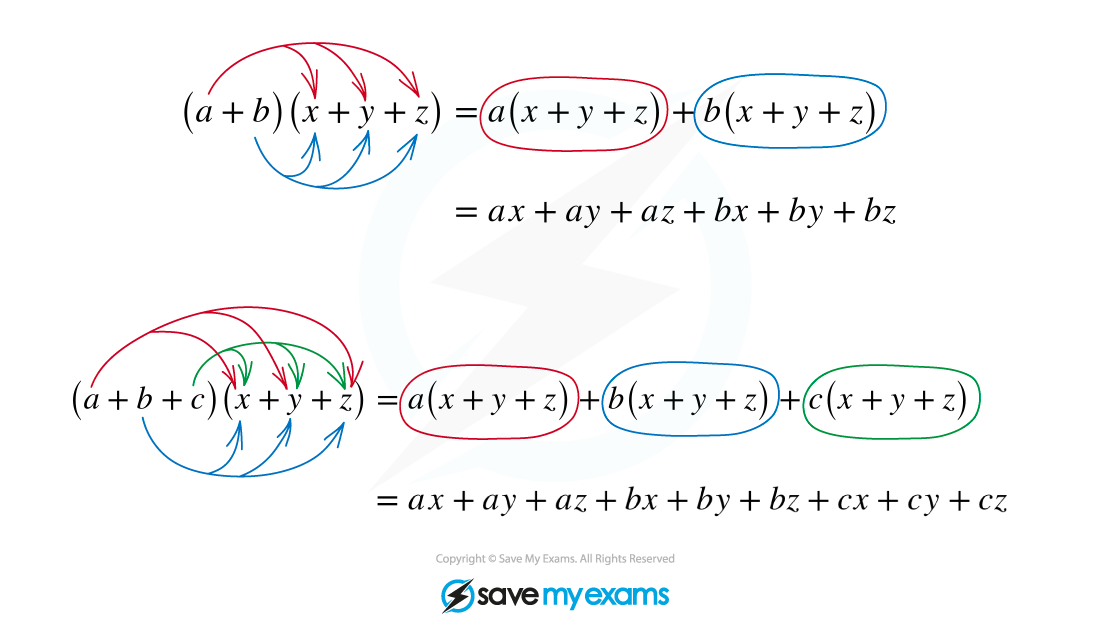
• 'FOIL' is a special case of this, when each set of brackets contains two terms you can mulitply in order
  • e.g. to multiply out or expand these brackets (2x + 3)(x - 5)
    • First (2x + 3)(x - 5) gives 2x²
    • Outside (2x + 3)(x - 5) gives -10x
    • Inside (2x + 3)(x - 5) gives +3x
    • Last (2x + 3)(x - 5) gives -15
  • (2x + 3)(x - 5) = 2x² -10x +3x -15 = 2x² -7x -15
• If you spot something like (a + b)² or (a + b)³ just write the brackets out twice or three times respectively
  • e.g. (3x + 2)² = (3x +2)(3x+2) = 9x² + 6x + 6x + 4 = 9x² + 12x + 4
• If you are trying to expand something like (a + b)ⁿ for powers of n greater than 2 or 3, use the binomial expansion
• If you have to expand more than two sets of brackets, just expand them two at a time