Quantities, Units & Modelling (OCR AS Maths: Mechanics)

State whether the quantity discussed in each of the following statements is a scalar or a vector.

A golf ball is hit with a force of .

A bath tub holds of water.

A hot air balloon rises vertically at .

The density of water is about .

Gravity on Earth is approximated to .

The average human has a body temperature of .

Convert the following into S.I. units:

per hour       

State the S.I. units which would be used to measure the following.

Car A is travelling at , Car B travels in 5 minutes.  Find the difference in their average speeds. Giving your answer in , correct to three significant figures.

Label the following diagrams with the appropriate forces.

A snowboarder sliding down a ski slope.

A parachuting penguin.

Two blocks, A and B are attached by means of a light inextensible string running over a smooth pulley, as shown in the diagram below. Block A is accelerating along a smooth horizontal surface in the direction shown, block B is moving towards the ground. Both A and B are modelled as particles.

Explain what effect each of the following assumptions have on the model described above.

Draw a simple diagram to represent each of the following models. Labelling your diagrams with the appropriate forces involved.
 

List any assumptions you may make in order to create a simple model for each of the following.

A canal barge travels in a straight line from home to two locks, A and B, as shown in the diagram below. The barge travels at a speed of on stretches of the canal that are over long and 4 km h^-1 on stretches that are under long.

Taking the positive direction as shown in the diagram, state the following in relation to the canal barge:

Convert the units in the following statements into S.I. units.

A hot air balloon descends at .

The density of oil is about .

The average spike ball player can hit the ball at a velocity of .

Roger throws a frisbee upwards, releasing it at a height of .

The motion of the frisbee is modelled by the equation

where h m is the height of the frisbee above the ground and x m is the horizontal distance travelled.

Write down the value of H needed to complete the model and explain why the model is only valid for .

Use the model to predict how far horizontally the frisbee will have travelled by the time it reaches the ground.

What is the maximum height the frisbee reaches?

By converting to S.I. units compare which of the following accelerates quickest.

A: A motorbike accelerates at .

B: A cheetah accelerates at . 

C: A race car accelerates at per square min.

Define each of the following and give an example of how they could be used in a mathematical model, include any related assumptions which can be made.

A lamina and a non-uniform rod.

A bead and wire. 

Label the following diagrams with the appropriate forces.

 
A speed boat travelling through water.

A climber abseiling down a cliff.

Fishing company, Fin-tastic Rods, are designing a new fishing pole. They set up a model as shown in the diagram below to consider the forces involved in catching a fish.

Explain what effect the following assumptions would have on the model described above and whether or not they are suitable.

Draw a simple diagram to represent each of the following models. Label your diagrams with the appropriate forces involved and detail any assumptions you make about your model.

List any assumptions you could make in order to create a simple model for each of the following.

A yo-yo moves in a straight line up and down a 1 m string. As it travels down the string it has a velocity of and a velocity of when returning towards the hand. In order to do certain tricks, the yo-yo stops at different places along the string as shown in the diagram below. The stops of one particular trick, ‘The Mechanic’, are as follows: Hand-2-1-3-1-Hand

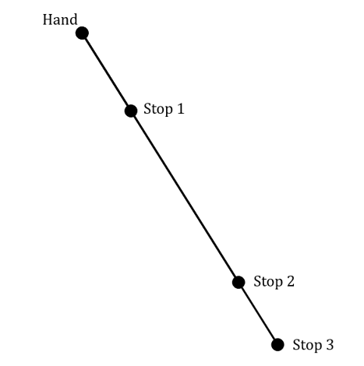
Stop 1 is  of the way along the string, Stop 2 is  of the way along the string. When the yo-yo reaches Stop 3 the string is fully extended. 

By indicating your chosen positive direction clearly on the diagram above, state the following in relation to the yo-yo:

A speedboat accelerates from rest to 90  in 5 . The distance travelled, d , in time t , can be modelled by a quadratic equation in the form . When the speedboat has travelled a distance of .

Use the model to find the distance travelled when the boat reaches .

For what range of values is  valid for this model. Give a reason for your answer.

Melody throws a netball into a net. The path of the ball from leaving Melody’s hand to passing through the net is modelled by the function

where h m is the height of the netball above the ground and x m is the horizontal distance travelled.

Find the height of the ball when it horizontally half way from being thrown to reaching its maximum height.

The above model is valid for , where k m is the horizontal distance of the net from the player. The standard height of a netball hoop is .

Find the value of k. Give your answer to three significant figures.

Explain why the model is not valid for .

A stone is thrown from the edge of a lake into the water. The height of the stone above the water level h m, at time t  after it is thrown is modelled by a quadratic equation in the form .

Explain why the value of a must be negative and what the variable c must indicate.

The diagram below shows a child holding onto a kite flying in the wind. Label the diagram with the appropriate forces, explain any assumptions you make about the diagram.

Define each of the following and give an example of how they could be used in a mathematical model, include any related assumptions which can be made.

The diagram below shows a basketball player taking a shot.

Label the forces on the ball at the three different stages of the throw.

A: As the player takes the shot, with their hands still in contact with the ball.

B: When the ball is in flight at its maximum height.

C: When the ball reaches the basket, hitting the back of the metal rim.

List any assumptions you made for each, or all, stages of the throw A, B and C in order to answer part (a).

Draw a simple diagram to represent each of the following models. Label your diagrams with the appropriate forces involved and detail any assumptions you make about each model.

A boxer hitting a punch bag suspended from the ceiling.

The forces acting on a cat batting at a piece of string held by its owner.

A particle is attached to the end of a rod. One end of the rod is fixed to a wall using a hinge, the other end is held using a piece of string before it is let go.

State all the assumptions that would need to be made to model the situation above. You may draw a diagram to support your answer.