Revision NotesExam QuestionsPast Papers
ASMathsOCRMaths APureRevision NotesAlgebra & FunctionsLaws of Indices & SurdsRationalising the Denominator with Surds

Rationalising the Denominator with Surds (OCR AS Maths A): Revision Note

Roger B

Author

Roger B

Last updated

OCR Maths A: PureAQA Maths: PureEdexcel Maths: Pure

Did this video help you?

Surds - Rationalising the Denominator

What does it mean to rationalise a denominator?

  • Rationalising a denominator changes a fraction with surds in its denominator, into an equivalent fraction where the denominator is a rational number (usually an integer) and any surds are in the numerator

 

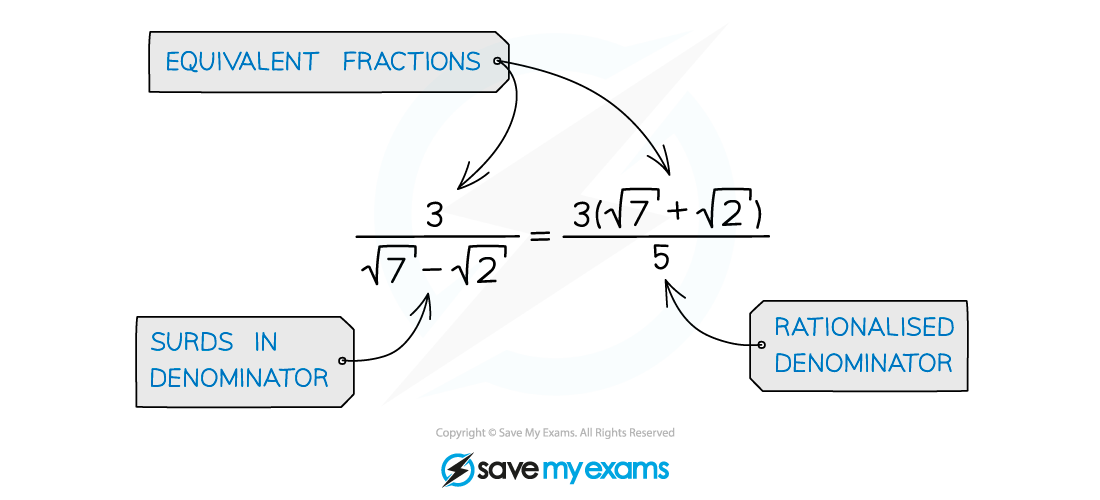
Explanation diagram, A Level & AS Level Pure Maths Revision Notes

  • There are three cases you need to know how to deal with when rationalising denominators:

2.1.3 List of rationalising denominator cases, Edexcel A Level Maths: Pure revision notes

Examiner Tips and Tricks

  • If an exam question asks you to give an answer, for example, "in the form p + q√3 , where p and q are rational numbers", this does NOT mean that p and q have to be integers, or positive!

  • Remember: both integers and fractions (both positive and negative) are rational numbers

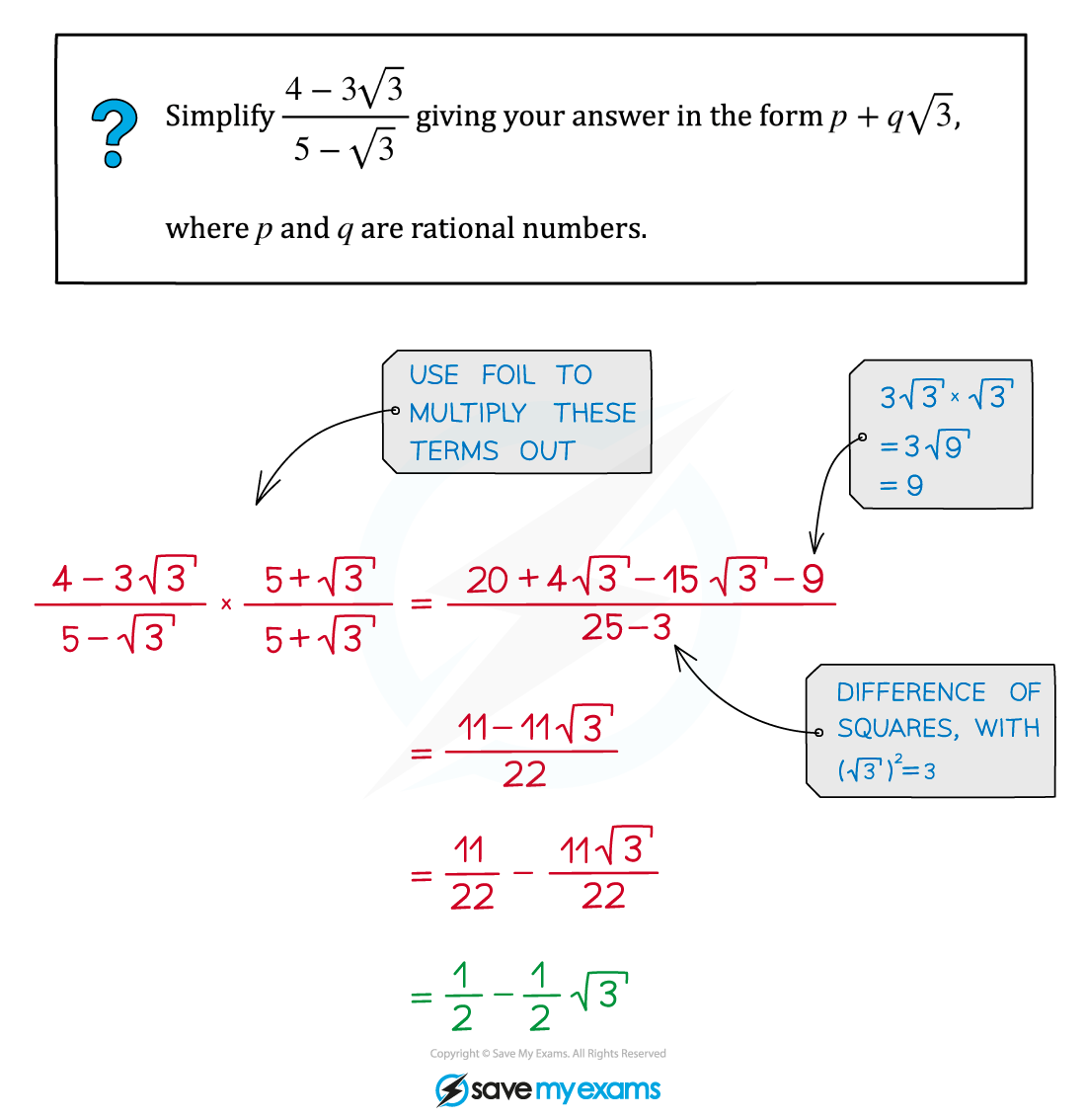
Worked Example

Rationalising the Denominator Example, A Level & AS Level Pure Maths Revision Notes

You've read 0 of your 5 free revision notes this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Manipulating SurdsNext:Quadratic Graphs
    Roger B

    Author: Roger B

    Expertise: Maths

    Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.