Reacting Masses & Volumes (CIE AS Chemistry)

• The number of moles of a substance can be found by using the following equation:

• It is important to be clear about the type of particle you are referring to when dealing with moles
  • Eg. 1 mole of CaF₂ contains one mole of CaF₂ formula units, but one mole of Ca²⁺ and two moles of F^- ions

Reacting masses

• The masses of reactants are useful to determine how much of the reactants exactly react with each other to prevent waste
• To calculate the reacting masses, the chemical equation is required
• This equation shows the ratio of moles of all the reactants and products, also called the stoichiometry, of the equation
• To find the mass of products formed in a reaction the following pieces of information is needed:
  • The mass of the reactants
  • The molar mass of the reactants
  • The balanced equation

Percentage yield

• In a lot of reactions, not all reactants react to form products which can be due to several factors:
  • Other reactions take place simultaneously
  • The reaction does not go to completion
  • Reactants or products are lost to the atmosphere

• The percentage yield shows how much of a particular product you get from the reactants compared to the maximum theoretical amount that you can get:

• Where actual yield is the number of moles or mass of product obtained experimentally
• The predicted yield is the number of moles or mass obtained by calculation

Worked example: Mass calculation using moles

Answer

• Step 1: The symbol equation is:

2Mg(s)    +     O₂(g)      →      2MgO(s)

• Step 2: The relative formula masses are:

 Magnesium : 24         Oxygen : 32         Magnesium Oxide : 40

• Step 3: Calculate the moles of magnesium used in reaction

• Step 4: Find the ratio of magnesium to magnesium oxide using the balanced chemical equation

Therefore, 0.25 mol of MgO is formed

• Step 5: Find the mass of magnesium oxide

mass = mol x M_r

mass = 10 g

Therefore, mass of magnesium oxide produced is 10 g

Worked Example: Calculate % yield using moles

Answer

• Step 1: The symbol equation is:

Zn (s)    +    CuSO₄ (aq)     →     ZnSO₄ (aq)    + Cu (s)

•  Step 2: Calculate the amount of zinc reacted in moles

• Step 3: Calculate the maximum amount of copper that could be formed from the molar ratio:

Since the ratio of Zn(s) to Cu(s) is 1:1 a maximum of 0.10 moles can be produced

• Step 4: Calculate the maximum mass of copper that could be formed (theoretical yield)

mass =   mol   x   M_r

mass =   0.10 mol x 64 g mol^-1

• Step 5: Calculate the percentage yield of copper

Excess & limiting reagents

• Sometimes, there is an excess of one or more of the reactants (excess reagent)
• The reactant which is not in excess is called the limiting reagent
• To determine which reactant is limiting:
  • The number of moles of the reactants should be calculated
  • The ratio of the reactants shown in the equation should be taken into account eg:

    C  + 2H₂      →     CH₄

There are 10 mol of Carbon reacting with 3 mol of Hydrogen

• Hydrogen is the limiting reagent and since the ratio of C : H₂ is 1:2 only 1.5 mol of C will react with 3 mol of H₂

Worked example: Excess & limiting reagent

Answer

• Step 1: Calculate the moles of each reactant

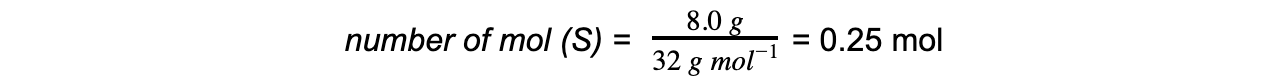
• Step 2: Write the balanced equation and determine the molar ratio

2Na + S → Na₂S

The molar ratio of Na: Na₂S is 2:1

• Step 3: Compare the moles and determine the limiting reagent

So to react completely 0.40 moles of Na require 0.20 moles of S and since there are 0.25 moles of S, then S is in excess. Na is therefore the limiting reactant.

Volumes of gases

• Avogadro suggested that ‘equal volumes of gases contain the same number of molecules’ (also called Avogadro’s hypothesis)
• At room temperature (20 degrees Celsius) and pressure (1 atm) one mole of any gas has a volume of 24.0 dm³
• This molar gas volume of 24.0 dm³ can be used to find:
  • The volume of a given mass or number of moles of gas:

volume of gas (dm³) = amount of gas (mol) x 24

• The mass or number of moles of a given volume of gas:

Worked example: Calculation volume of gas using excess & limiting reagents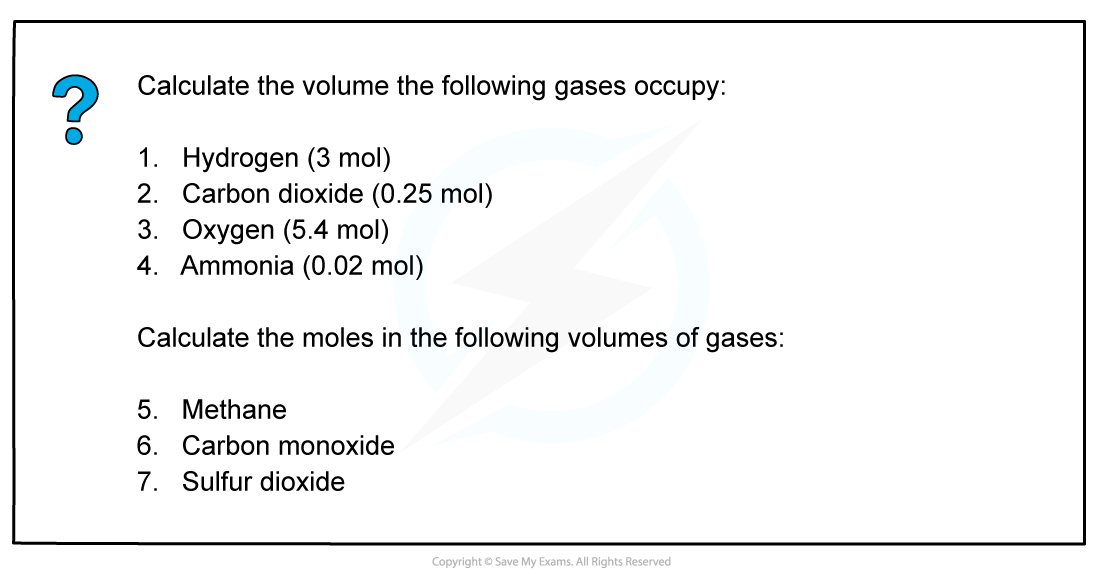

Answer

Volumes & concentrations of solutions

• The concentration of a solution is the amount of solute dissolved in a solvent to make 1 dm³ of  solution
  • The solute is the substance that dissolves in a solvent to form a solution
  • The solvent is often water

• A concentrated solution is a solution that has a high concentration of solute
• A dilute solution is a solution with a low concentration of solute
• When carrying out calculations involve concentrations in mol dm^-3 the following points need to be considered:
  • Change mass in grams to moles
  • Change cm³ to dm³ 

• To calculate the mass of a substance present in solution of known concentration and volume:
  • Rearrange the concentration equation

number of moles (mol) = concentration (mol dm^-3) x volume (dm³)

• • Multiply the moles of solute by its molar mass

mass of solute (g) = number of moles (mol) x molar mass (g mol^-1)

Worked example: Calculating volume from concentration

Answer

• Step 1: Write the balanced symbol equation

CaCO₃  +  2HCl  →  CaCl₂  +  H₂O  +  CO₂

• Step 2: Calculate the amount, in moles, of calcium carbonate that reacts

• Step 3: Calculate the moles of hydrochloric acid required using the reaction’s stoichiometry

1 mol of CaCO₃ requires 2 mol of HCl

So 0.025 mol of CaCO₃ requires 0.05 mol of HCl

• Step 4: Calculate the volume of HCl required

Volume of hydrochloric acid = 0.05 dm³

Worked example: Neutralisation calculation

Answer

• Step 1: Write the balanced symbol equation

Na₂CO₃  +  2HCl  →  Na₂Cl₂  +  H₂O  +  CO₂

• Step 2: Calculate the amount, in moles, of sodium carbonate reacted by rearranging the equation for amount of substance (mol) and dividing by the volume by 1000 to convert cm³ to dm³

amount (Na₂CO₃) = 0.025 dm³ x 0.050 mol dm^-3 = 0.00125 mol

• Step 3: Calculate the moles of hydrochloric acid required using the reaction’s stoichiometry

1 mol of Na₂CO₃ reacts with 2 mol of HCl, so the molar ratio is 1 : 2

Therefore 0.00125 moles of Na₂CO₃ react with 0.00250 moles of HCl

• Step 4: Calculate the concentration, in mol dm^-3 of hydrochloric acid

concentration (HCl) (mol dm^-3) = 0.125 mol dm^-3

Stoichiometric relationships

• The stoichiometry of a reaction can be found if the exact amounts of reactants and products formed are known
• The amounts can be found by using the following equation:

• The gas volumes can be used to deduce the stoichiometry of a reaction
  • Eg. in the combustion of 50 cm³ of propane reacting with 250 cm³ of oxygen, 150 cm³  of carbon dioxide is formed suggesting that the ratio of propane:oxygen:carbon dioxide is 1:5:3

C₃H₈ (g) + 5O₂ (g) → 3CO₂ (g) + H₂O (l)