AP®StatisticsCollege BoardStudy GuidesUnit 4: Probability, Random Variables & Probability DistributionsDiscrete Random VariablesCumulative Probability Distributions for Discrete Random Variables

Cumulative Probability Distributions for Discrete Random Variables (College Board AP® Statistics)

Study Guide

Test yourself
Dan Finlay

Written by: Dan Finlay

Reviewed by: Lucy Kirkham

Discrete cumulative probability distributions

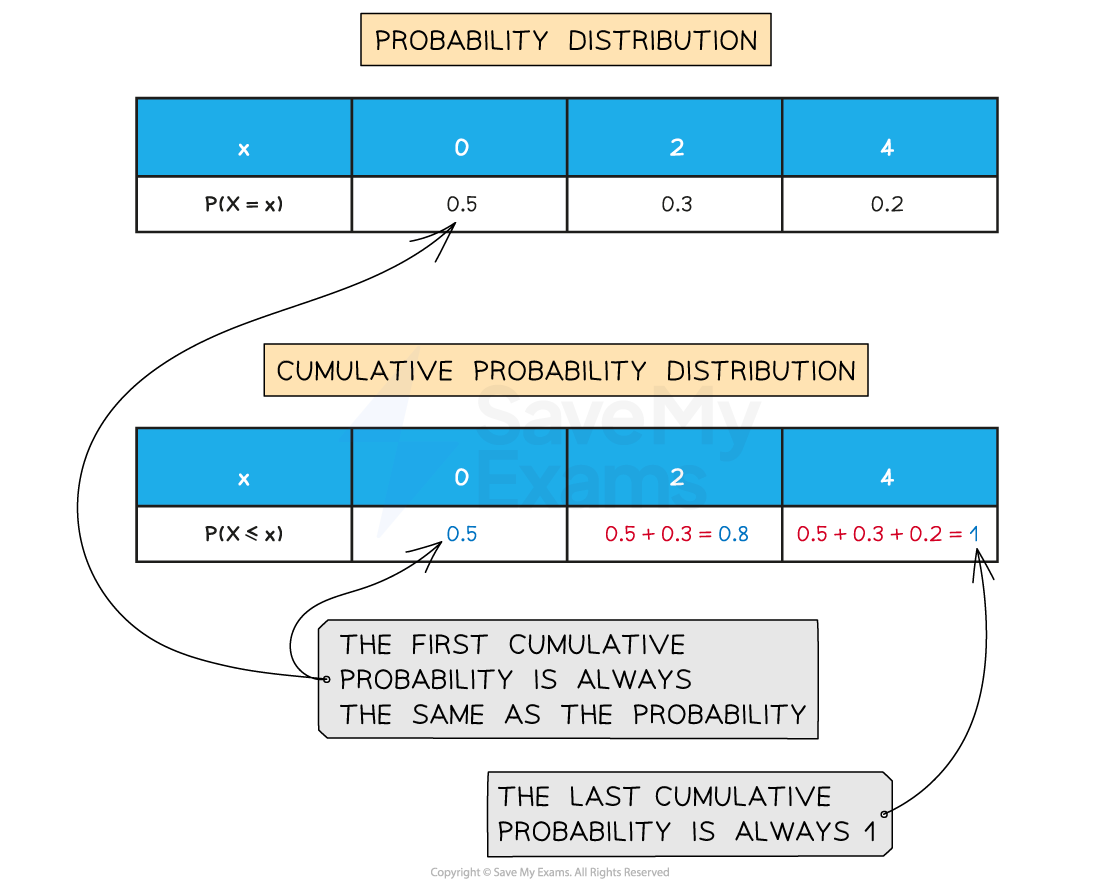
What is a discrete cumulative probability distribution?

  • A discrete cumulative probability distribution shows the probability that a discrete random variable is less than or equal to each of its possible values

  • A discrete cumulative probability distribution can be given as either a table or a function

  • To find the cumulative probability P open parentheses X less or equal than x close parentheses

    • identify the values of the random variable that are less than or equal to the x

    • add together the probabilities of these values

  • The cumulative probability of the smallest value is always equal to the probability of that value

    • e.g. if X can only take the values 0, 2 or 4, then P open parentheses X less or equal than 0 close parentheses equals P open parentheses X equals 0 close parentheses

  • The cumulative probability of the largest value is always equal to 1

    • e.g. if X can only take the values 0, 2 or 4, then P open parentheses X less or equal than 4 close parentheses equals 1

Diagram showing probability and cumulative probability distributions. First probability is 0.5, second is 0.3, third is 0.2. Cumulative sums are 0.5, 0.8, and 1.
Example of a cumulative probability distribution

How can I find probabilities using a cumulative probability distribution?

  • To find P open parentheses X less or equal than x close parentheses

    • if X can take the value x

      • read P open parentheses X less or equal than x close parentheses directly from the distribution

    • if X cannot take the value x

      • find the biggest value of X that is less than x

      • find the cumulative probability of this value

        • e.g. if X can take the values, 1, 2, 3 or 5 then P open parentheses X less or equal than 4 close parentheses equals P open parentheses X less or equal than 3 close parentheses

  • To find P open parentheses X less than x close parentheses

    • find the biggest value of X that is less than x

    • find the cumulative probability of this value

      • e.g. if X can take the values, 1, 2, 3 or 5 then P open parentheses X less than 5 close parentheses equals P open parentheses X less or equal than 3 close parentheses

  • To find P open parentheses X greater than x close parentheses use the identity

    • P open parentheses X greater than x close parentheses equals 1 minus P open parentheses X less or equal than x close parentheses

  • To find P open parentheses X greater or equal than x close parentheses use the identity

    • P open parentheses X greater or equal than x close parentheses equals 1 minus P open parentheses X less than x close parentheses

  • To find P open parentheses a less or equal than X less or equal than b close parentheses use the identity

    • P open parentheses X less or equal than b close parentheses minus P open parentheses X less than a close parentheses

      • Note that a is not included in the second inequality

  • To find P open parentheses X equals x close parentheses

    • find the biggest value of X that is less than x

    • find the cumulative probability of this value

    • subtract this probability from P open parentheses X less or equal than x close parentheses

      • e.g. if X can take the values, 1, 2, 3 or 5 then P open parentheses X equals 3 close parentheses equals P open parentheses X less or equal than 3 close parentheses minus P open parentheses X less or equal than 2 close parentheses

Worked Example

X is a discrete random variable that can take any positive integer value. The cumulative probability distribution is given by the function P open parentheses X less or equal than x close parentheses equals fraction numerator 1 over denominator x plus 1 end fraction, where x is a positive integer.

(a) Find P open parentheses X equals 1 close parentheses.

Answer:

1 is the first positive integer and so is the smallest value that X can take

Therefore P open parentheses X less or equal than 1 close parentheses equals P open parentheses X equals 1 close parentheses

Substitute x equals 1 into the function

table row cell P open parentheses X less or equal than 1 close parentheses end cell equals cell fraction numerator 1 over denominator 1 plus 1 end fraction end cell row blank equals cell 1 half end cell end table

P open parentheses X equals 1 close parentheses equals 1 half

(b) Find P open parentheses X greater or equal than 5 close parentheses.

Answer:

Use the identity P open parentheses X greater or equal than x close parentheses equals 1 minus P open parentheses X less than x close parentheses

P open parentheses X greater or equal than 5 close parentheses equals 1 minus P open parentheses X less than 4 close parentheses

3 is the largest value that X can take that is also less than 4

P open parentheses X less than 4 close parentheses equals P open parentheses X less or equal than 3 close parentheses

Substitute x equals 3 into the function

table row cell P open parentheses X less or equal than 3 close parentheses end cell equals cell fraction numerator 1 over denominator 3 plus 1 end fraction end cell row blank equals cell 1 fourth end cell end table

This is also P open parentheses X less than 4 close parentheses so substitute into P open parentheses X greater or equal than 5 close parentheses equals 1 minus P open parentheses X less than 4 close parentheses

table row cell P open parentheses X greater or equal than 5 close parentheses end cell equals cell 1 minus 1 fourth end cell row blank equals cell 3 over 4 end cell end table

P open parentheses X greater or equal than 5 close parentheses equals 3 over 4

(c) What is the value of P open parentheses X less or equal than 3.5 close parentheses?

(A) 0

(B) 1 fourth

(C) 2 over 9

(D) 1 fifth

Answer:

X cannot take the value of 3.5

3 is the largest value that X can take that is also less than 3.5

Substitute x equals 3 into the function

table row cell P open parentheses X less or equal than 3 close parentheses end cell equals cell fraction numerator 1 over denominator 3 plus 1 end fraction end cell row blank equals cell 1 fourth end cell end table

The correct answer is B

Last updated:

You've read 0 of your 10 free study guides

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Probability Distributions for Discrete Random VariablesNext:Mean & Standard Deviation of a Discrete Random Variable
    Author
    Reviewer
    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.

    Lucy Kirkham

    Author: Lucy Kirkham

    Expertise: Head of STEM

    Lucy has been a passionate Maths teacher for over 12 years, teaching maths across the UK and abroad helping to engage, interest and develop confidence in the subject at all levels.Working as a Head of Department and then Director of Maths, Lucy has advised schools and academy trusts in both Scotland and the East Midlands, where her role was to support and coach teachers to improve Maths teaching for all.