Study GuidesExam QuestionsPast Papers
AP®PhysicsCollege BoardPhysics 1: Algebra-BasedExam QuestionsUnit 5: Torque & Rotational DynamicsRotational Kinematics

Rotational Kinematics (College Board AP® Physics 1: Algebra-Based): Exam Questions

15 mins15 questions

Select a download format for Rotational Kinematics

Scroll for more
Easy
Medium
Hard
1
Sme Calculator1 mark

A record on a turntable is initially at rest before experiencing a constant angular acceleration of alpha.

Which of the following gives the angular displacement of the record after 4 seconds?

  • 2 alpha

  • 4 alpha

  • 8 alpha

  • 16 alpha

Choose your answer

Did this page help you?

2
Sme Calculator1 mark
Graph showing angular velocity in radians per second versus time in seconds. The velocity rises linearly to 20 rad/s when t = 2 s, then remains constant at 20 rad/s until t = 4 s.

The graph shows the angular velocity of a wheel as a function of time.

Which of the following is most nearly the angular displacement of the wheel at t space equals space 4 space straight s?

  • 5 space rad

  • 20 space rad

  • 60 space rad

  • 80 space rad

Choose your answer

Did this page help you?

3
Sme Calculator1 mark
A pulley system with two blocks, A and B. Block A ascends while Block B descends. Arrows direction: the clockwise angular acceleration of the pulley α and the acceleration a of the blocks.

Two blocks, A and B, are attached to a rope which runs over a frictionless pulley of radius 20 cm, as shown in the figure. When the blocks are released, block A accelerates upward, block B accelerates toward the ground and the pulley experiences a clockwise angular acceleration of 10 rad/s2.

Which of the following is most nearly the magnitude of the acceleration of the blocks?

  • 1.0 space straight m divided by straight s squared

  • 2.0 space straight m divided by straight s squared

  • 4.0 space straight m divided by straight s squared

  • 50 space straight m divided by straight s squared

Choose your answer

Did this page help you?

4
Sme Calculator1 mark
Graph showing angular velocity in rad/s versus time in seconds. Between t = 0 and t = 2 s, the angular velocity remains constant at +7.5 rad/s, then between t = 2 and t = 6, it decreases linearly to -7.5 rad/s, then remains constant at this value until t = 8 s.

The graph shows the angular velocity omega of a disk rotating about its axis as a function of time t.

Which of the following statements is true about the motion of the disk?

  • The disk is not rotating between t space equals space 0 and t space equals space 2 space straight s.

  • The disk's rotation is slowing down between t space equals space 4 space straight s and t space equals space 6 space straight s.

  • The disk is not rotating between t space equals space 6 space straight s and t space equals space 8 space straight s.

  • The disk's rotation is speeding up between t space equals space 4 space straight s and t space equals space 6 space straight s.

Choose your answer

Did this page help you?

5
Sme Calculator1 mark

A wheel of radius 0.5 space straight m, which is initially at rest on the ground, begins rolling forward with an angular acceleration of 4 space rad divided by straight s squared.

Which of the following is most nearly the distance traveled by the wheel in 3 seconds?

  • 3 space straight m

  • 6 space straight m

  • 9 space straight m

  • 18 space straight m

Choose your answer

Did this page help you?

1
Sme Calculator1 mark
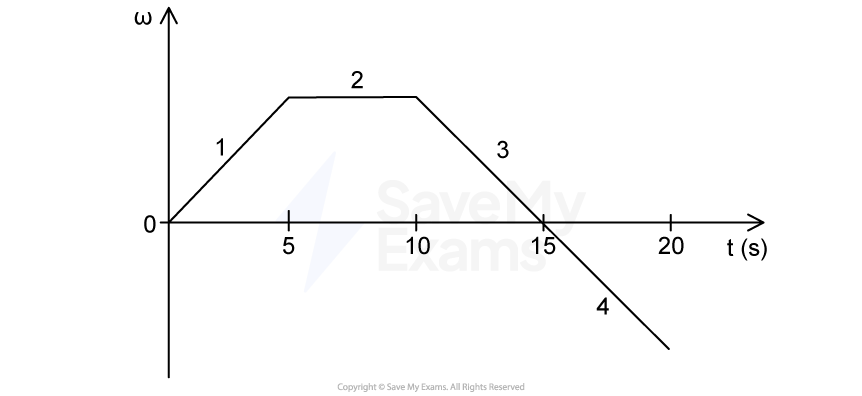
Graph of angular velocity (ω) vs time (t) in seconds, showing four linear segments: increasing, constant, decreasing, and returning to zero.

The graph shows the angular velocity omega as a function of time t for an object that rotates about an axis. The time intervals labeled 1-4 divide the graph into four sections.

Which of the following correctly compares the angular displacement increment theta of the object during each time interval?

  • increment theta subscript 2 space greater than space increment theta subscript 1 space equals space increment theta subscript 3 space equals space increment theta subscript 4

  • increment theta subscript 2 space greater than space increment theta subscript 1 space equals space increment theta subscript 3 space greater than space increment theta subscript 4

  • increment theta subscript 4 space greater than space increment theta subscript 3 space greater than space increment theta subscript 2 space greater than space increment theta subscript 1

  • increment theta subscript 1 space greater than space increment theta subscript 2 space greater than space increment theta subscript 3 space greater than space increment theta subscript 4

Choose your answer

Did this page help you?

2
Sme Calculator1 mark

Two points, A and B, are located on a disk that rotates about an axis. Point A is three times as far from the axis as point B. The linear speed of point B is v.

Which of the following expressions correctly represents the linear speed of point A?

  • 1 third v

  • v

  • 2 v

  • 3 v

Choose your answer

Did this page help you?

3
Sme Calculator1 mark

A car with 50 cm diameter tires is traveling at 9.0 m/s. When the brakes are applied, the car comes to a stop after the tires complete 12.0 revolutions.

Which of the following is most nearly the magnitude of the angular acceleration of the tires when the brakes are applied?

  • 2.1 space rad divided by straight s squared

  • 4.3 space rad divided by straight s squared

  • 8.6 space rad divided by straight s squared

  • 17 space rad divided by straight s squared

Choose your answer

Did this page help you?

4
Sme Calculator1 mark

The radius of the Earth is 6400 km.

Which of the following is most nearly the linear speed of a point on the Earth's surface at the equator?

  • 0.47 space straight m divided by straight s

  • 1.7 space straight m divided by straight s

  • 470 space straight m divided by straight s

  • 1700 space straight m divided by straight s

Choose your answer

Did this page help you?

5
Sme Calculator1 mark

A disk of radius 60 space mm is made to spin by applying a constant force to its outer rim. It takes 3.0 space seconds for the disk to rotate from rest up to a rate of 500 revolutions per minute.

Which of the following is most nearly the linear acceleration at a point on the outer rim of the disk during the application of the force?

  • straight pi over 3 space straight m divided by straight s squared

  • straight pi space straight m divided by straight s squared

  • fraction numerator 10 straight pi over denominator 3 end fraction space straight m divided by straight s squared

  • fraction numerator 50 straight pi over denominator 9 end fraction space straight m divided by straight s squared

Choose your answer

Did this page help you?

11 mark
Graph of angular displacement in radians vs. time in seconds showing a parabolic curve with marked points A, B, C, and D at various times.

The graph shows the angular displacement as a function of time for a disc rotating with constant angular acceleration.

At which positions shown on the graph are the angular velocity and angular acceleration of the disc both positive?

  • Positions A and D only

  • Position B only

  • Positions C and D only

  • Position D only

Choose your answer

Did this page help you?

2
Sme Calculator1 mark
Diagram of a vertical rod with a spinning object of mass m, at angle θ, rotating in a circle of radius R, with angular speed ω.

A ball of mass m is attached by two strings to a vertical rod, as shown in the figure. The entire system is made to rotate about the axis of the rod with angular speed omega.

If the lower string is just taut, which of the following expressions gives the minimum time required for the ball to complete one revolution?

  • 2 straight pi square root of fraction numerator R over denominator g space tan space theta end fraction end root

  • fraction numerator 1 over denominator 2 straight pi end fraction square root of fraction numerator g space tan space theta over denominator R end fraction end root

  • fraction numerator 2 straight pi R over denominator g space tan space theta end fraction

  • fraction numerator g space tan space theta over denominator 2 straight pi R end fraction

Choose your answer

Did this page help you?

3
Sme Calculator1 mark
An overhead view of a horizontal track for a rollercoaster. The track begins at point O and forms a circular arc of radius r to point P which subtends an angle of θP.

The first section of a horizontal rollercoaster track is designed to include a circular arc of radius r. The rollercoaster starts from rest at point O with a constant tangential acceleration of g and reaches a net acceleration of 4 g at point P. The angle subtended by the circular arc is theta subscript P, as shown in the figure.

Which of the following gives the angle theta subscript P required for the rollercoaster to achieve a net acceleration of 4 g at point P?

  • 3 over 2 space rad

  • fraction numerator square root of 15 over denominator 2 end fraction space rad

  • 2 space rad

  • square root of 15 space rad

Choose your answer

Did this page help you?

4
Sme Calculator1 mark

A flywheel has an initial angular velocity of omega subscript 0 space equals space plus 5.0 space rad divided by straight s and a constant angular acceleration of alpha space equals space minus 0.25 space rad divided by straight s squared.

Which of the following pairs of graphs best represent the flywheel's angular displacement and angular velocity as functions of time?

  • Two graphs: (1) Angular displacement as a function of time showing a parabola opening downwards starting at a non-zero initial angular position. (2) Angular velocity as a function of time showing a straight line with a positive slope starting at zero.
  • Two graphs: (1) Angular displacement as a function of time showing a parabola opening downwards starting at zero. (2) Angular velocity as a function of time showing a straight line with a negative slope starting at a non-zero initial angular velocity.
  • Two graphs: (1) Angular displacement as a function of time showing a straight line with a negative slope starting at a non-zero initial angular position. (2) Angular velocity as a function of time showing a parabola opening downwards starting at a non-zero initial angular velocity.
  • Two graphs: (1) Angular displacement as a function of time showing a parabola opening upwards starting at zero. (2) Angular velocity as a function of time showing a straight line with a negative slope starting at a non-zero initial angular velocity.

Choose your answer

Did this page help you?

5
Sme Calculator1 mark
Diagram of a light experiment with a light source passing through a rotating slotted wheel towards a perpendicular mirror a distance L away which reflects it back to the wheel.

In an experiment to measure the speed of light, a student sets up a light source, a rotating slotted wheel, and a mirror, as shown in the figure. The beam of light passes through the centre of a slot at the edge of the wheel, reflects from the distant mirror, and returns to the wheel just in time to pass through the centre of the next slot.

The wheel has a radius of 5.0 space cm and 500 slots around its edge. The distance between the mirror and the wheel is L space equals space 600 space straight m and the student obtains a value of 3.0 cross times 10 to the power of 5 space km divided by straight s for the speed of light.

Which of the following is most nearly the linear speed of a point on the edge of the wheel?

  • 5 straight pi space straight m divided by straight s

  • 50 straight pi space straight m divided by straight s

  • 100 straight pi space straight m divided by straight s

  • 1000 straight pi space straight m divided by straight s

Choose your answer

Did this page help you?