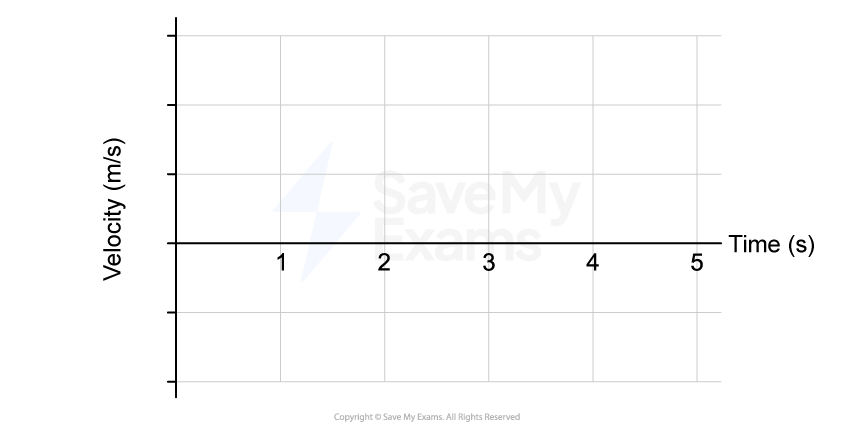
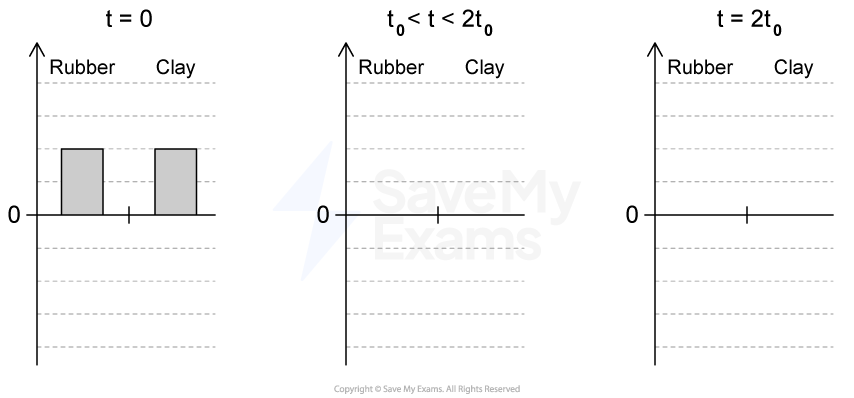
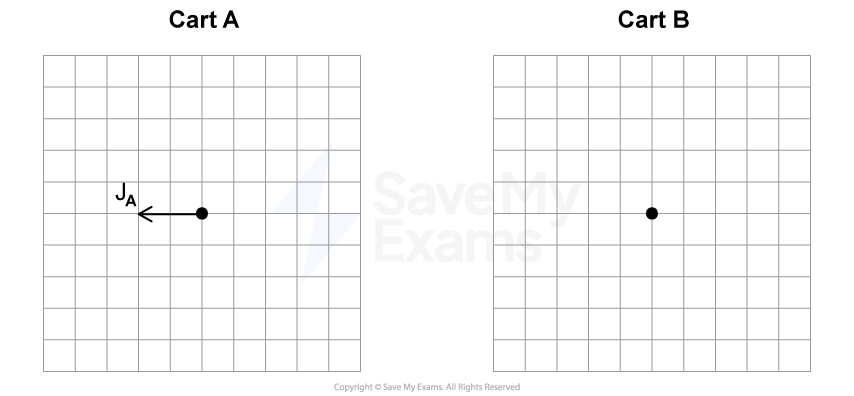
Define the term linear momentum.
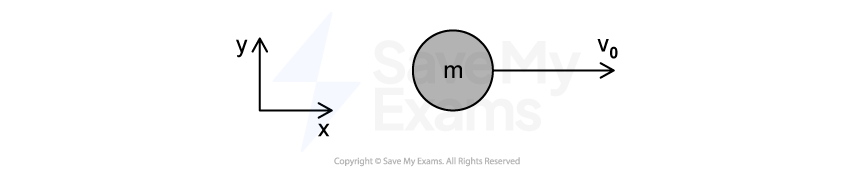
Figure 1
An object of mass moves with speed
, as shown in Figure 1.
i) Determine an expression for the magnitude of the object's momentum.
ii) Indicate the direction of the object's momentum. Justify your answer.
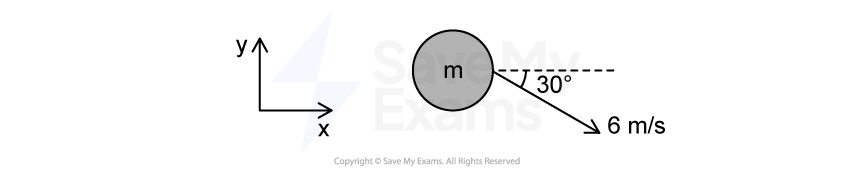
Figure 2
The mass of the object is . A force is exerted on the object and changes its direction, as shown in Figure 2.
i) Calculate the x-component of the object's momentum.
ii) Calculate the y-component of the object's momentum.
Before the force is applied, the speed of the object is .
i) Determine the change in the -component of the object's momentum.
ii) Determine the change in the -component of the object's momentum.
Did this page help you?