Study GuidesExam QuestionsPast Papers
AP®PhysicsCollege BoardPhysics 1: Algebra-BasedStudy GuidesUnit 5: Torque & Rotational DynamicsNewton’s First & Second Law in Rotational FormNewton’s First Law in Rotational Form

Newton’s First Law in Rotational Form (College Board AP® Physics 1: Algebra-Based)

Study Guide

Katie M

Written by: Katie M

Reviewed by: Caroline Carroll

Newton’s first law in rotational form

  • The rotational analog of Newton’s first law states:

If the net torque exerted on a system is zero, the angular velocity of that system will remain constant

  • A constant angular velocity could also be an angular velocity of zero, i.e. when the system is not rotating

  • If the net torque acting on a system is zero, it is said to be in rotational equilibrium

Unbalanced torque

  • According to Newton’s second law, if the torques exerted on a rigid system are not balanced, the system’s angular velocity must be changing

  • Therefore, a net torque produces an angular acceleration

  • The direction of this angular acceleration depends on the direction of the net torque

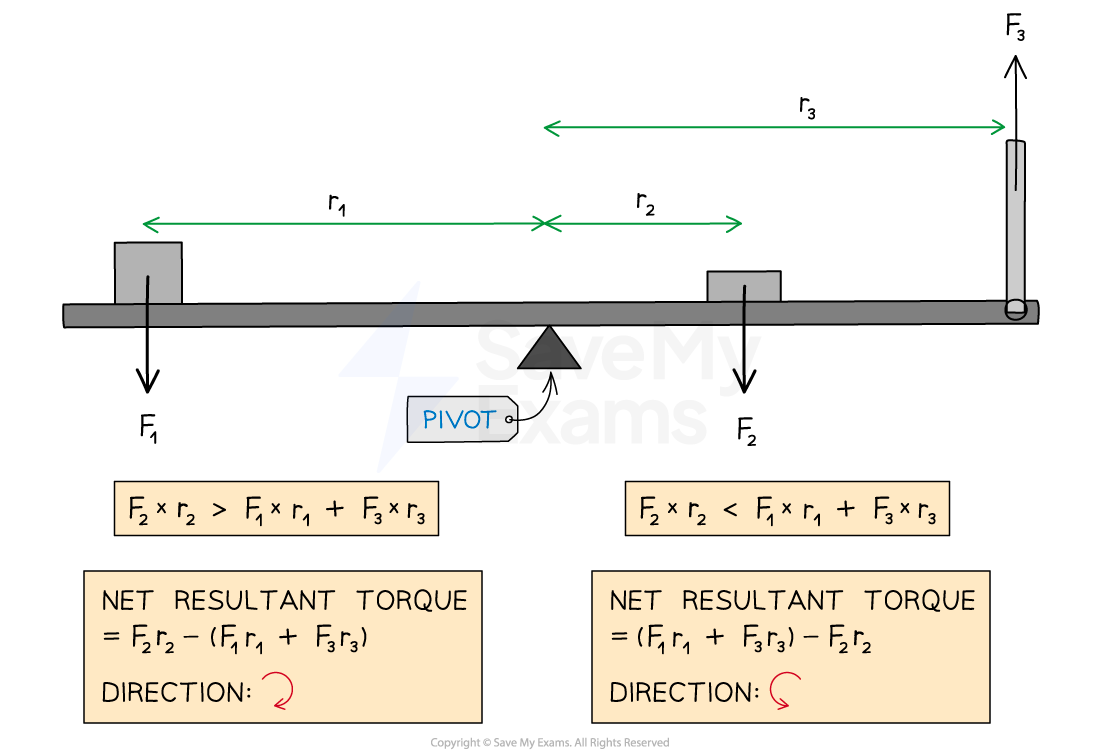
Beam with an unbalanced torque

A diagram showing a lever with forces applied at three different points, labeled F1, F2, and F3, with their respective distances from the pivot indicated as r1, r2, and r3. Text boxes below show torque equations.
The diagram shows two different scenarios: net torque in the clockwise and counterclockwise directions. If there is a net torque in the clockwise or counterclockwise direction, the beam will also have an angular acceleration in that direction.

Worked Example

Three forces act on a wheel which is free to rotate about its center O, as shown in the diagram.

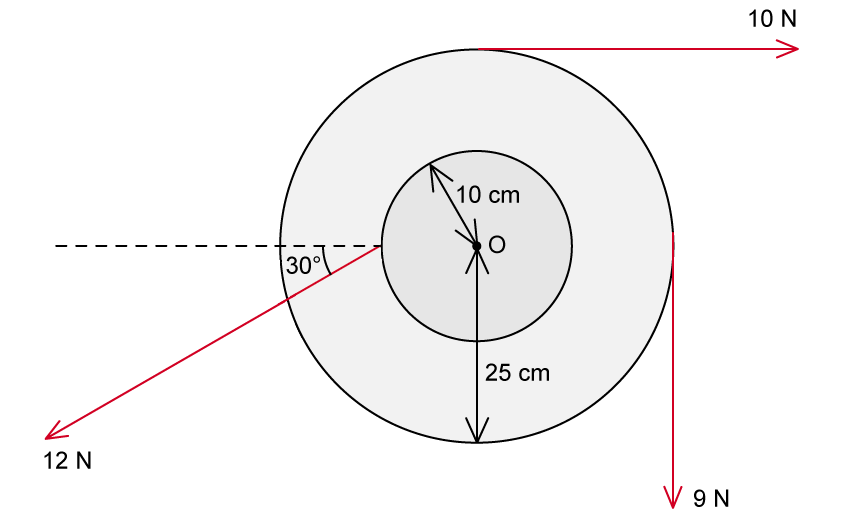
Diagram showing a circular disk with a central point O. Various force vectors (10 N, 12 N, 9 N) are applied at different points and angles. Dimensions are 10 cm and 25 cm.

Calculate the net torque about O and state whether the angular acceleration that is produced is clockwise or counterclockwise.

Answer:

Step 1: Analyze the scenario

  • The magnitude of the torque about the axis of rotation O is given by

tau space equals space F r space sin space theta

  • The 9 N force and the 10N force are both perpendicular to the radius and produce a torque in the clockwise direction

  • The perpendicular component of the 12 N force produces a torque in the counterclockwise direction

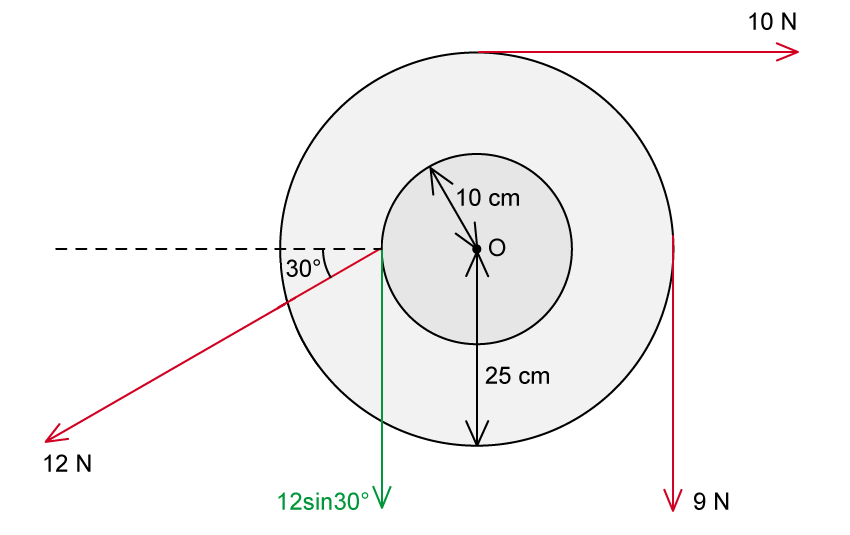
Diagram shows a circle with forces: 12 N at 30 degrees to the left, 10 N to the right, 9 N downward, and components 12sin30° downward. Radii are 10 cm and 25 cm.

Step 2: Calculate the total clockwise torque

  • The torque due to the 10 N force is

tau space equals space 10 cross times 0.25 cross times sin space 90 space equals space 2.5 space straight N times straight m

  • The torque due to the 9 N force is

tau space equals space 9 cross times 0.25 cross times sin space 90 space equals space 2.25 space straight N times straight m

  • Therefore, the total clockwise torque = 2.5 + 2.25 = 4.75 N m

Step 3: Calculate the total counterclockwise torque

  • The torque due to the 12 N force is:

tau space equals space 12 cross times 0.1 cross times sin space 30 space equals 0.6 space straight N times straight m

  • Therefore, the total counterclockwise torque = 0.6 N m

Step 4: Determine the net torque and direction of angular acceleration

Net torque = total clockwise torque − total counterclockwise torque

Net torque = sum tau space equals space 4.75 space minus space 0.6 space equals space 4.15 space straight N times straight m, clockwise

  • The net torque and angular acceleration both act in the clockwise direction

Examiner Tips and Tricks

In the AP Physics 1 exam, you will not be expected to analyze rotation in multiple planes. When analyzing the torques exerted on a system, you only need to be able to consider whether they produce clockwise or counterclockwise motion

Diagram of a lever with a pivot point. It shows the effects of forces labeled clockwise and anti-clockwise on either side of the pivot.

Last updated:

You've read 0 of your 5 free study guides this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Rotational EquilibriumNext:Newton’s Second Law in Rotational Form
    Author
    Reviewer
    Katie M

    Author: Katie M

    Expertise: Physics

    Katie has always been passionate about the sciences, and completed a degree in Astrophysics at Sheffield University. She decided that she wanted to inspire other young people, so moved to Bristol to complete a PGCE in Secondary Science. She particularly loves creating fun and absorbing materials to help students achieve their exam potential.

    Caroline Carroll

    Author: Caroline Carroll

    Expertise: Physics Subject Lead

    Caroline graduated from the University of Nottingham with a degree in Chemistry and Molecular Physics. She spent several years working as an Industrial Chemist in the automotive industry before retraining to teach. Caroline has over 12 years of experience teaching GCSE and A-level chemistry and physics. She is passionate about creating high-quality resources to help students achieve their full potential.