Fluid Flow Rates (College Board AP® Physics 1: Algebra-Based)

Fluid flow rates

Pressure difference

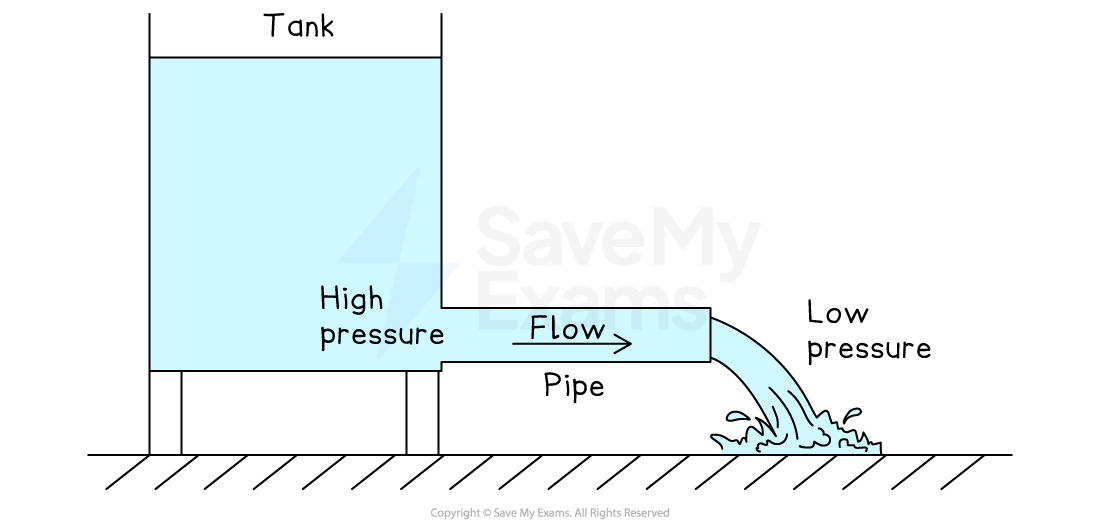
• A pressure difference is required for a fluid to flow

• Consider a large tank of water with an outlet pipe at the bottom, the water flows out of it because:

  • the end of the pipe attached to the tank experiences a higher gauge pressure due to the large height of water

  • the end of the pipe open to the air experiences atmospheric pressure only

• If pressure was equal on each end, no net force would be exerted on the water, and it would not flow

• One example of this pressure difference is a pipe releasing water from a large tank

  • The end of the pipe at the bottom of the tank experiences a large gauge pressure

  • The end of the pipe open to the air only experiences atmospheric pressure

  • The pressure difference causes fluid to flow

Pressure difference causing fluid flow

Conservation of mass

• The fluid in an open-ended tube is incompressible and flows steadily

  • This means the total mass of fluid in the pipe at any one time is constant

• Mass is conserved, so the rate at which mass passes any point is constant

  • If 15 kg of fluid enters the pipe in 2 s, 15 kg must exit the pipe in the same 2 s

• Density is constant, so this means the rate at which volume passes a given point is also constant

Conservation of mass flow rate

Derived equation

• The rate at which matter flows past a point is proportional to the cross-sectional area of the flow and the speed at which the fluid flows

• This is known as the volume flow rate equation:

• Where:

  • = volume of fluid passing a point, measured in

  • = time taken for the volume to pass the point, measured in

  • = cross-sectional area of the flow at that point, measured in

  • = speed of the flow at that point, measured in

Fluid passing a point in a pipe

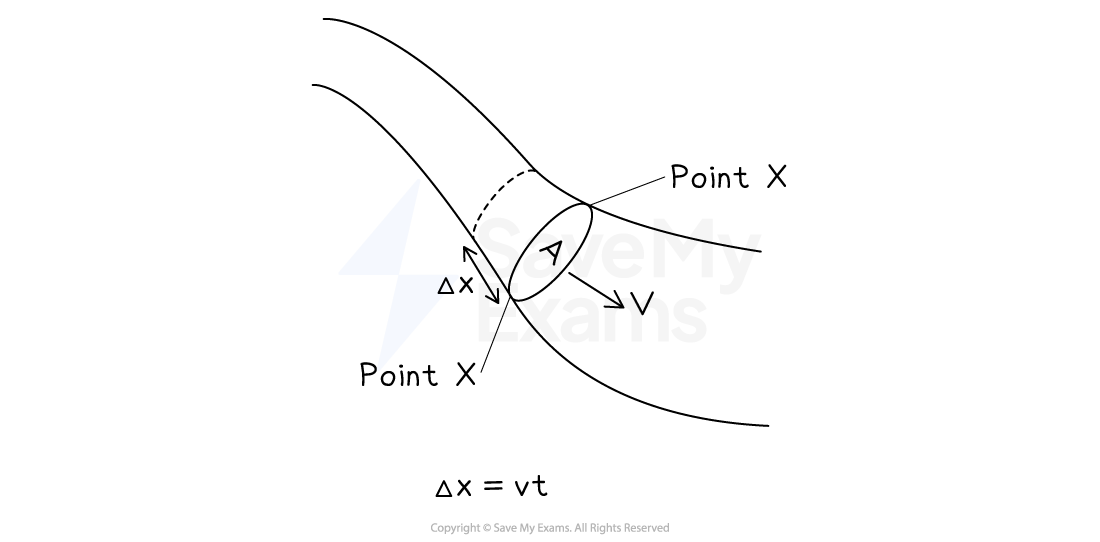
Derivation

Step 1: Identify the fundamental principles

• The volume of fluid passing a given point X per unit time is constant

  • This is due to the conservation of mass

• Fluid in a steady flow is always traveling at the same speed when passing a given point

Step 2: Apply the specific conditions

• Using the above diagram, the fluid has a cross-sectional area at point X

• A column of fluid of volume passes point X

  • This column has a length

  • It takes time for the column to pass point X

• The length of the column can be written in terms of its speed and time:

• The volume of the column of fluid can be written in terms of its cross-sectional area and length:

Step 3: Combine the specific conditions

• Substituting the expression for the column's length gives:

• Rearranging this gives the expression for the rate of flow of volume past a point: