A slope field for the differential equation is shown below.
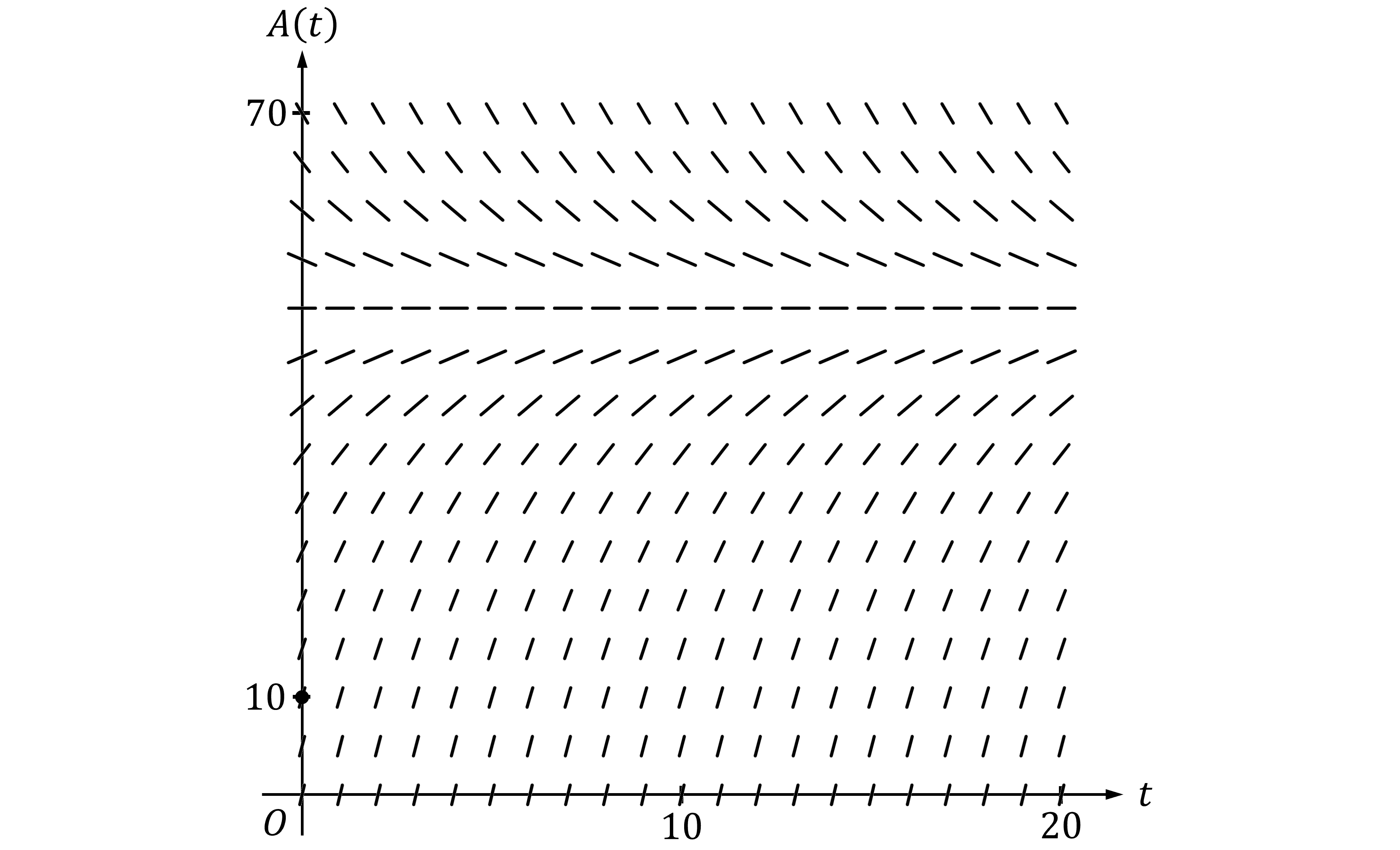
Sketch the solution curve through the point .
Did this page help you?
Select a download format for First-Order Differential Equations
Select an answer set to view for
First-Order Differential Equations
A slope field for the differential equation is shown below.

Sketch the solution curve through the point .
How did you do?
Did this page help you?
A portion of the slope field for the differential equation is given below.

Sketch the solution curve through the point .
How did you do?
Did this page help you?
Consider the differential equation .
On the axes provided, sketch a slope field for the given differential equation at the six points indicated.

How did you do?
Did this page help you?
Consider the differential equation .
On the axes provided, sketch a slope field for the given differential equation at the nine points indicated.

How did you do?
Did this page help you?
Consider the differential equation .
Describe all the points in the -plane,
, for which
.
How did you do?
Did this page help you?
Consider the differential equation . Let
be the particular solution to that differential equation with the initial condition
. Use Euler's method with a step size of
, starting at
, to approximate
. Show the work that leads to your answer.
How did you do?
Did this page help you?
A population is modeled by a function that satisfies the logistic differential equation
.
If , what is
?
If , what is
?
How did you do?
Did this page help you?
A cylindrical tank contains water and is being used to fill up a garden pond. The tank is standing upright on its circular base. The rate of change of the height of the water in the tank with respect to time
is modeled by
, where
is measured in feet and
is measured in seconds.
At time seconds, the height of water in the tank is 4 feet. Use separation of variables to find an expression for
in terms of
.
How did you do?
Did this page help you?
Consider the differential equation .
There is a horizontal line with equation that satisfies this differential equation. Find the value of
.
How did you do?
Did this page help you?
Consider the differential equation .
Find the particular solution to the differential equation with the initial condition
.
How did you do?
Did this page help you?
Consider the differential equation .
Describe all the points in the -plane for which the slopes of tangent lines drawn on a slope field for that differential equation will be negative.
How did you do?
Did this page help you?
Consider the differential equation .
Find the particular solution to the differential equation with the initial condition
.
How did you do?
Did this page help you?
3 | 5 | 7 | 2 | 1 |
is a twice-differentiable function for all values of
, with
. The table above gives values of the derivative of
,
, for selected values of
.
Use Euler's method, with two steps of equal size starting at , to approximate
. Show the computations that lead to your answer.
How did you do?
Did this page help you?
Consider the logistic differential equation for a population
. Let
be the particular solution to the differential equation for a given initial condition.
If , what is the range of
for
?
How did you do?
If, instead, , for what value of
is the population growing the fastest?
How did you do?
Did this page help you?
Consider the differential equation .
Find the particular solution to the differential equation such that the line
is tangent to the graph of
.
How did you do?
Did this page help you?
Scientists are studying a population of leopards in a nature reserve. The rate of change of the population is proportional to the difference between the current population and the maximum population that the reserve can support. If is the population at time
months after the start of the study, then
Use separation of variables to find the particular solution to the differential equation with initial condition . Give your answer as an expression for
in terms of
.
How did you do?
Did this page help you?
Use separation of variables to find , the particular solution to the differential equation
with the initial condition
.
How did you do?
Did this page help you?
Consider the differential equation .
Find the values of the constants and
, for which
is a solution to the differential equation.
How did you do?
Did this page help you?
Consider the differential equation .
Find the particular solution to the differential equation with the initial condition
How did you do?
Did this page help you?
Let be the particular solution to the differential equation
with initial condition
. Use Euler's method, with two equal steps starting at
, to approximate
. Determine whether this approximation is an over- or underestimate, being sure to justify your answer.
How did you do?
Did this page help you?
A population is modeled by a function that satisfies the logistic differential equation
.
Use separation of variables to find if
.
How did you do?
Determine the value of the time, , at which the population,
, is changing the fastest.
How did you do?
Did this page help you?