Evaluating Limits Numerically & Graphically (College Board AP® Calculus BC): Study Guide
Limits from tables
How can I estimate a limit using values in a table?
Values of a function in a table can show the behavior of a function near a point
This can allow you to estimate the limit at that point
For example, let
be the function defined by
The table below shows values of the function near
Note that the function is not defined at
, because
x | f(x) |
|---|---|
-0.1 | 0.49958347 |
-0.01 | 0.49999583 |
-0.001 | 0.49999996 |
0 | not defined |
0.001 | 0.49999996 |
0.01 | 0.49999583 |
0.1 | 0.49958347 |
From the table we can see that
gets nearer and nearer to 0.5 as
gets nearer and nearer to 0
Therefore we can estimate that
is equal to 0.5
Analytical methods would need to be used to confirm that this is indeed the limit
Limits from graphs
How can I estimate a limit using a graph?
A graph can show the behavior of a function near a point
This can allow you to estimate the limit at that point
For example, let
be the function defined by
The graph below shows the behavior the function near
Note that the function is not defined at
, because
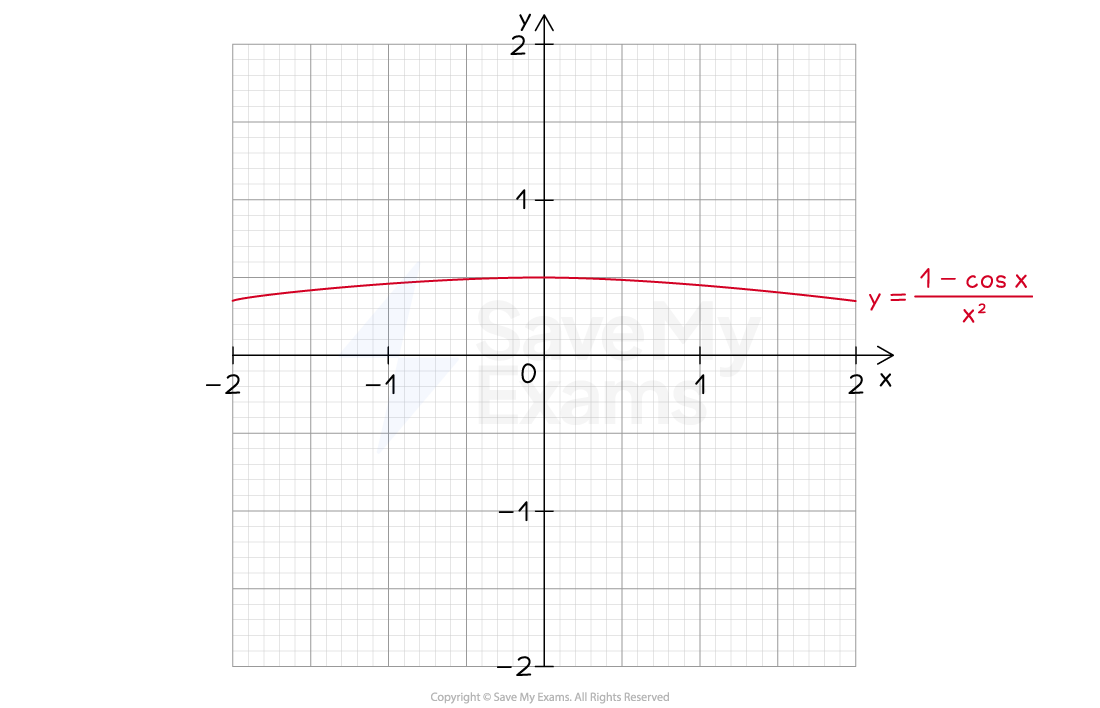
From the graph we can see that
gets nearer and nearer to 0.5 as
gets nearer and nearer to 0
Therefore we can estimate that
is equal to 0.5
Analytical methods would need to be used to confirm that this is indeed the limit
Examiner Tips and Tricks
You can graph functions on your graphing calculator to check your answers when determining limits analytically.
Horizontal asymptote
What is a horizontal asymptote?
A horizontal asymptote is a horizontal line
that the graph of a function gets closer and closer to (but never touches or intersects)
as x becomes unbounded in the positive or negative direction
On the following diagram, the horizontal asymptote is indicated by a dashed line
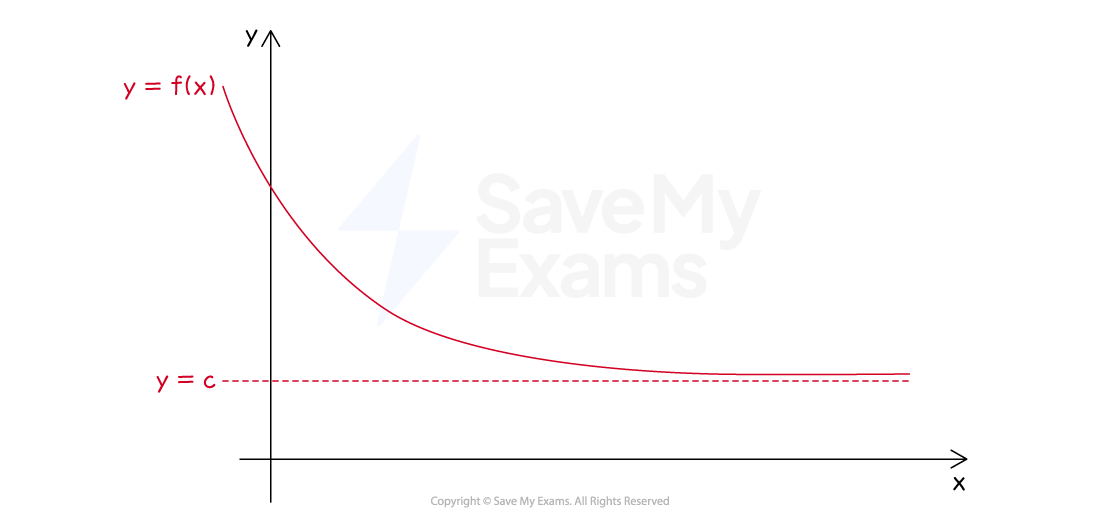
How can I identify horizontal asymptotes using limits?
A function will have a horizontal asymptote if it has a finite limit at infinity
I.e. the line
will be a horizontal asymptote for the graph of a function
if
, or
Horizontal asymptotes (if any) may therefore be determined by evaluating the limits at infinity
Examiner Tips and Tricks
By graphing a function on your graphing calculator you can:
spot any asymptotic behavior by a function at plus or minus infinity
check limits that you have determined analytically
Vertical asymptote
What is a vertical asymptote?
A vertical asymptote is a vertical line
that the graph of a function gets closer and closer to (but never touches or intersects)
as x gets closer and closer to the x-value of the vertical line
On the following diagram, the vertical asymptote is indicated by a dashed line
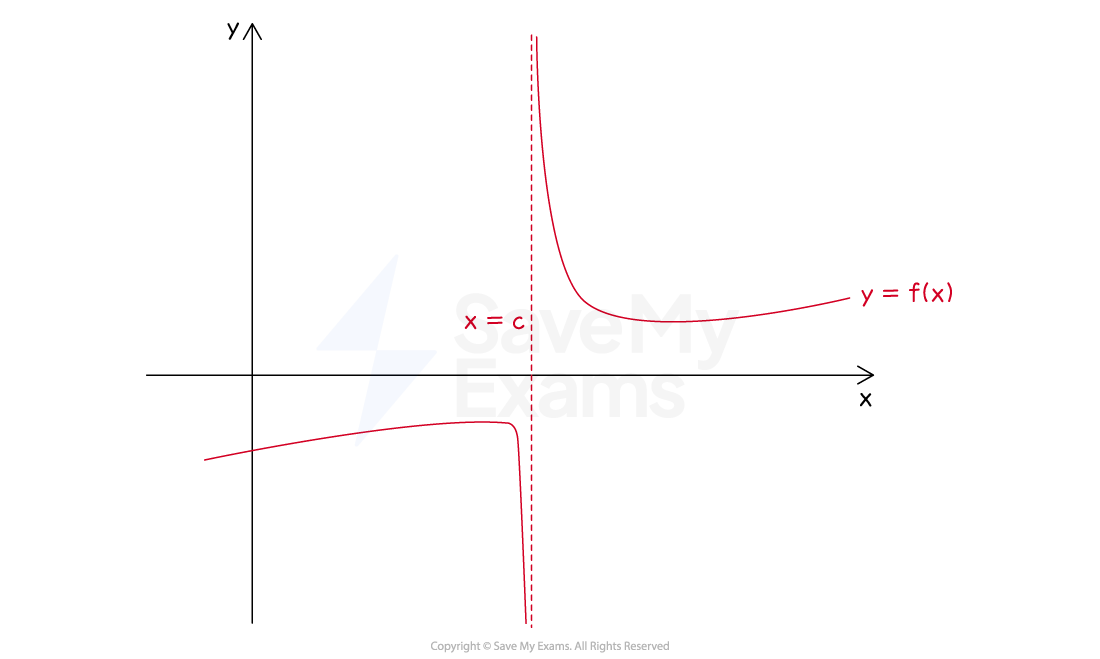
How can I identify vertical asymptotes using limits?
A function will have a vertical asymptote at any x-value where the function becomes unbounded
I.e. the line
will be a vertical asymptote for the graph of a function
if
, or
Vertical asymptotes (if any) may therefore be determined by identifying points where the function becomes unbounded
Usually this will involve a function in the form of a quotient
at points where the denominator becomes zero
Examiner Tips and Tricks
By graphing a function on your graphing calculator you can:
spot any asymptotic (i.e. unbounded) behavior by a function at certain values of x
check that vertical asymptotes you determine analytically are correct
Worked Example
Let be the function defined by
.
Using limits, identify the vertical and horizontal asymptotes (if any) on the graph of .
Answer:
The denominator becomes 0 when , so start by considering the limits there
At 2 the numerator is equal to -5, so zero only occurs in the denominator
Just 'to the left' of 2, and
so
Just 'to the right' of 2, and
so
This confirms that the graph of has a vertical asymptote at
To identify horizontal asymptotes, start by rearranging to make the behavior of the function more obvious
becomes closer and closer to zero as x increases in the positive or negative directions, so
This means that the graph of has a horizontal asymptote at
The graph of has a vertical asymptote at
, and a horizontal asymptote at

Unlock more, it's free!
Did this page help you?