Improper Integrals (College Board AP® Calculus BC)

Study Guide

Written by: Dan Finlay

Reviewed by: Mark Curtis

Updated on 22 January 2025

Evaluating improper integrals

What is an improper integral?

An improper integral is of the form $\int_{a}^{b} f (x) d x$ where
- at least one of the limits of integration is infinite
- or $f$ is unbounded at a point in the interval $[a, b]$
An improper integral can either converge to a limit or diverge

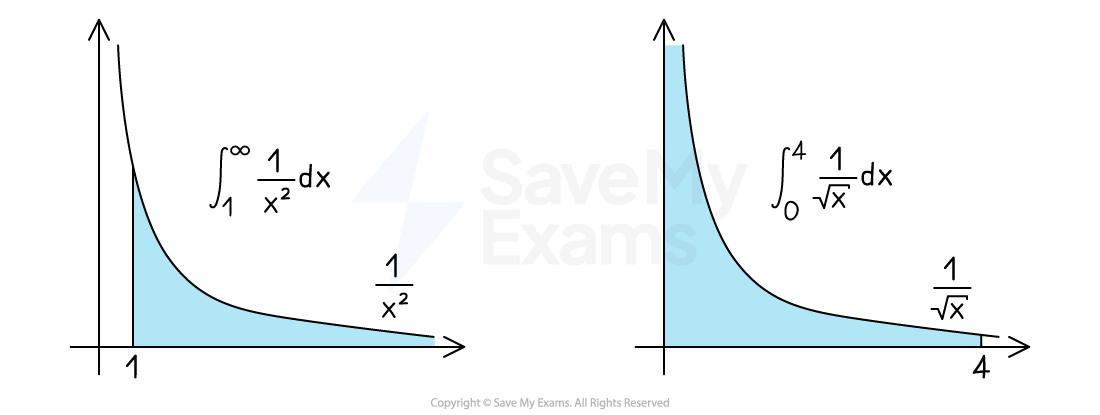
Two graphs showing integrals: left, integral of 1/x² from 1 to ∞; right, integral of 1/√x from 0 to 4. Both areas under curves are shaded blue. — **Examples of improper integrals and their corresponding areas**

How do I evaluate an improper integral with infinite limits?

Case 1: One of the limits is infinite

$\int_{a}^{\infty} f (x) d x$ or $\int_{- \infty}^{b} f (x) d x$

STEP 1
Write the improper integral as a limit
- Replace the infinite limit of integration with a variable
- Take the limit as that variable approaches the infinite limit
  - e.g. $\int_{1}^{\infty} \frac{1}{x^{2}} d x = \lim_{k \to \infty} \int_{1}^{k} \frac{1}{x^{2}} d x$
STEP 2
Evaluate the definite integral
- It will be an expression in terms of the newly introduced variable
  - e.g. $\lim_{k \to \infty} \int_{1}^{k} \frac{1}{x^{2}} d x = \lim_{k \to \infty} {[- \frac{1}{x}]}_{1}^{k} = \lim_{k \to \infty} (- \frac{1}{k} + 1)$
STEP 3
Find the value of the limit if it exists
- e.g. $\lim_{k \to \infty} (- \frac{1}{k} + 1) = 1$
- If the limit does not exist, the improper integral is divergent

Case 2: Both of the limits are infinite

$\int_{- \infty}^{\infty} \frac{1}{x^{2} + 1} d x$

STEP 1
Write the integral as a sum of two integrals
- Choose any value as the limit of integration where the two integrals 'meet'
  - e.g. $\int_{- \infty}^{\infty} \frac{1}{x^{2} + 1} d x = \int_{- \infty}^{0} \frac{1}{x^{2} + 1} d x + \int_{0}^{\infty} \frac{1}{x^{2} + 1} d x$
STEP 2
Write each integral as a limit
- e.g. $\lim_{h \to - \infty} \int_{h}^{0} \frac{1}{x^{2} + 1} d x + \lim_{k \to \infty} \int_{0}^{k} \frac{1}{x^{2} + 1} d x$
  - Use a different limit variable for each integral
STEP 3
Evaluate each definite integral
- e.g. $\lim_{h \to - \infty} {[\arctan x]}_{h}^{0} + \lim_{k \to \infty} {[\arctan x]}_{0}^{k} = \lim_{h \to - \infty} (- \arctan (h)) + \lim_{k \to \infty} (\arctan (k))$
STEP 4
Find the value of each limit and add them together
- e.g. $\lim_{h \to - \infty} (- \arctan (h)) + \lim_{k \to \infty} (\arctan (k)) = - (- \frac{π}{2}) + \frac{π}{2} = π$
- If either of the limits does not exist, the improper integral is divergent

Worked Example

Show that the improper integral $\int_{- \infty}^{\infty} e^{x} d x$ is divergent.

Answer:

STEP 1
Write the integral as a sum of two integrals

$\int_{- \infty}^{\infty} e^{x} d x = \int_{- \infty}^{0} e^{x} d x + \int_{0}^{\infty} e^{x} d x$

STEP 2
Write each integral as a limit

$\int_{- \infty}^{\infty} e^{x} d x = \lim_{h \to - \infty} \int_{h}^{0} e^{x} d x + \lim_{k \to \infty} \int_{0}^{k} e^{x} d x$

STEP 3
Evaluate each definite integral

$\begin{array}{rcl} \int_{- \infty}^{\infty} e^{x} d x & = & \lim_{h \to - \infty} {[e^{x}]}_{h}^{0} + \lim_{k \to \infty} {[e^{x}]}_{0}^{k} \\ = & \lim_{h \to - \infty} (1 - e^{h}) + \lim_{k \to \infty} (e^{k} - 1) \end{array}$

STEP 4
Find the value of each limit and add them together

$\begin{array}{rcl} \lim_{h \to - \infty} (1 - e^{h}) & = & 1 - 0 = 1 \\ \lim_{k \to \infty} (e^{k} - 1) & = & \infty \end{array}$

$\int_{- \infty}^{\infty} e^{x} d x$ is divergent because $\begin{array}{rcl} \lim_{k \to \infty} (e^{k} - 1) \end{array}$ is not finite

How do I evaluate an improper integral with unbounded integrands?

$\int_{a}^{b} f (x) d x$

Case 1: The function is unbounded at one of the endpoints

STEP 1
Write the improper integral as a limit by replacing the relevant limit of integration with a variable
- If you are replacing the lower limit of integration, then take the limit as that variable approaches the original value from above
  - e.g. $\int_{0}^{4} \frac{1}{\sqrt{x}} d x = \lim_{h \to 0^{+}} \int_{h}^{4} \frac{1}{\sqrt{x}} d x$
- If you are replacing the upper limit of integration, then take the limit as that variable approaches the original value from below
  - e.g. $\int_{0}^{1} \frac{1}{x - 1} d x = \lim_{k \to 1^{-}} \int_{0}^{k} \frac{1}{x - 1} d x$
STEP 2
Evaluate the definite integral
- It will be an expression in terms of the newly introduced variable
  - e.g. $\lim_{h \to 0^{+}} \int_{h}^{4} \frac{1}{\sqrt{x}} d x = \lim_{h \to 0^{+}} {[2 \sqrt{x}]}_{h}^{4} = \lim_{h \to 0^{+}} (4 - 2 \sqrt{h})$
  - e.g. $\lim_{k \to 1^{-}} \int_{0}^{k} \frac{1}{x - 1} d x = \lim_{k \to 1^{-}} {[\ln |x - 1|]}_{0}^{k} = \lim_{k \to 1^{-}} (\ln |k - 1|)$
STEP 3
Find the value of the limit if it exists
- e.g. $\lim_{h \to 0^{+}} (4 - 2 \sqrt{h}) = 4$
- If the limit does not exist, the improper integral is divergent
  - e.g. $\lim_{k \to 1^{-}} (\ln |k - 1|) = - \infty$ so $\int_{0}^{1} \frac{1}{x - 1} d x$ is divergent

Case 2: The function is unbounded at a point in the interval (a, b)

STEP 1
Write the integral as a sum of two integrals
- Use the point of the essential discontinuity as the limit of integration where the two integrals 'meet'
  - e.g. $\frac{1}{x^{2}}$ is unbounded at $x = 0$
  - so $\int_{- 1}^{1} \frac{1}{x^{2}} d x = \int_{- 1}^{0} \frac{1}{x^{2}} d x + \int_{0}^{1} \frac{1}{x^{2}} d x$
STEP 2
Write each integral as a limit
- e.g. $\lim_{k \to 0^{-}} \int_{- 1}^{k} \frac{1}{x^{2}} d x + \lim_{h \to 0^{+}} \int_{h}^{1} \frac{1}{x^{2}} d x$
STEP 3
Evaluate each definite integral
- e.g. $\lim_{k \to 0^{-}} {[- \frac{1}{x}]}_{- 1}^{k} + \lim_{h \to 0 +} {[- \frac{1}{x}]}_{h}^{1} = \lim_{k \to 0^{-}} (- \frac{1}{k} + 1) + \lim_{h \to 0 +} (- 1 + \frac{1}{h})$
STEP 4
Find the value of each limit
- If both limits exist, add them together to find the limit of the improper integral
- If either of the limits does not exist, the improper integral is divergent
  - e.g. $\lim_{k \to 0^{-}} (- \frac{1}{k} + 1) = + \infty$ so $\int_{- 1}^{1} \frac{1}{x^{2}} d x$ diverges
    - In this case $\lim_{h \to 0 +} (- 1 + \frac{1}{h}) = + \infty$ as well
    - But one unbounded limit is enough to show that the improper integral diverges

Examiner Tips and Tricks

Always check whether the function to be integrated is unbounded at any point in the interval. If you forget to check, you might end up with an incorrect answer that seems to work mathematically.

For example, you might incorrectly write

$\int_{- 1}^{1} \frac{1}{x^{2}} d x = {[- \frac{1}{x}]}_{- 1}^{1} = - \frac{1}{1} - (- \frac{1}{- 1}) = - 1 - 1 = - 2$ .

This is untrue due to the essential discontinuity at $x = 0$ .

Examiner Tips and Tricks

Your first step should always be to write the improper integral as a limit of a definite integral. If you need to use a u-substitution when calculating the definite integral, then remember to change the limits of integration.

Worked Example

Evaluate $\int_{0}^{1} \frac{x}{\sqrt{1 - x^{2}}} d x$ or show that the integral diverges.

Answer:

The integrand is undefined when $x = 1$

STEP 1
Write the improper integral as a limit by replacing the relevant limit of integration with a variable

$\int_{0}^{1} \frac{x}{\sqrt{1 - x^{2}}} d x = \lim_{k \to 1^{-}} \int_{0}^{k} \frac{x}{\sqrt{1 - x^{2}}} d x$

STEP 2
Evaluate the definite integral

$\int_{0}^{1} \frac{x}{\sqrt{1 - x^{2}}} d x = \lim_{k \to 1^{-}} \int_{0}^{k} x {(1 - x^{2})}^{- \frac{1}{2}} d x$

Use the substitution $u = 1 - x^{2}$ and change the limits of integration

$u = 1 - x^{2} \frac{d u}{d x} = - 2 x \Rightarrow - \frac{1}{2} d u = x d x x = 0 \Rightarrow u = 1 x = k \Rightarrow u = 1 - k^{2}$

$\begin{array}{rcl} \int_{0}^{1} \frac{x}{\sqrt{1 - x^{2}}} d x & = & \lim_{k \to 1^{-}} \int_{1}^{1 - k^{2}} - \frac{1}{2} u^{- \frac{1}{2}} d u \\ = & \lim_{k \to 1^{-}} {[- \frac{1}{2} \cdot 2 u^{\frac{1}{2}}]}_{1}^{1 - k^{2}} \\ = & \lim_{k \to 1^{-}} {[- \sqrt{u}]}_{1}^{1 - k^{2}} \end{array}$

STEP 3
Find the value of the limit if it exists

$\begin{array}{rcl} \int_{0}^{1} \frac{x}{\sqrt{1 - x^{2}}} d x & = & \lim_{k \to 1^{-}} (- \sqrt{1 - k^{2}} + 1) \\ = & 1 \end{array}$

$\begin{array}{rcl} \int_{0}^{1} \frac{x}{\sqrt{1 - x^{2}}} d x & = & 1 \end{array}$

Summary of improper integrals

The general approach is to write an improper integral as the limit of a definite integral
The table below shows the relevant limit to use

Reason for the improper integral	Limit of a definite integral
The upper limit of integration is infinite	$\int_{a}^{\infty} f (x) d x = \lim_{k \to \infty} \int_{a}^{k} f (x) d x$
The lower limit of integration is infinite	$\int_{- \infty}^{b} f (x) d x = \lim_{h \to - \infty} \int_{h}^{b} f (x) d x$
Both limits of integration are infinite	$\begin{array}{rcl} \int_{- \infty}^{\infty} f (x) d x & = & \int_{- \infty}^{c} f (x) d x + \int_{c}^{\infty} f (x) d x \\ = & \lim_{h \to - \infty} \int_{h}^{c} f (x) d x + \lim_{k \to \infty} \int_{c}^{k} f (x) d x \end{array}$ For any value $c$
The integrand is unbounded at the upper limit of integration	$\int_{a}^{b} f (x) d x = \lim_{k \to b -} \int_{a}^{k} f (x) d x$
The integrand is unbounded at the lower limit of integration	$\int_{a}^{b} f (x) d x = \lim_{h \to a +} \int_{h}^{b} f (x) d x$
The integrand is unbounded at a point $c$ in the interval $(a, b)$	$\begin{array}{rcl} \int_{a}^{b} f (x) d x & = & \int_{a}^{c} f (x) d x + \int_{c}^{b} f (x) d x \\ = & \lim_{k \to c^{-}} \int_{a}^{k} f (x) d x + \lim_{h \to c^{+}} \int_{h}^{b} f (x) d x \end{array}$

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Did this page help you?

Previous:Integration Using Partial FractionsNext:Selecting Techniques for Integration

Improper Integrals (College Board AP® Calculus BC)

Study Guide

Evaluating improper integrals

What is an improper integral?

How do I evaluate an improper integral with infinite limits?

Case 1: One of the limits is infinite

Case 2: Both of the limits are infinite

How do I evaluate an improper integral with unbounded integrands?

Case 1: The function is unbounded at one of the endpoints

Case 2: The function is unbounded at a point in the interval (a, b)

Summary of improper integrals

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

Unit 1: Limits & Continuity

Limits

Definition of a Limit

Infinite Limits & Limits at Infinity

Properties of Limits

Evaluating Limits Analytically

Evaluating Limits Numerically & Graphically

Squeeze Theorem & Trigonometric Limits

Summary of Limits

Selecting Procedures for Determining Limits

Continuity

Continuity

Removable Discontinuities

Non-removable Discontinuities

Intermediate Value Theorem

Unit 2: Differentiation Definition & Fundamental Properties

Definition of Differentiation

Average Rate of Change

Instantaneous Rate of Change

Derivatives & Tangents

Estimating the Derivative at a Point

Differentiability & Continuity

Fundamental Properties of Differentiation

Derivative Rules

Derivatives of Exponentials and Logarithms

Derivatives of Sine and Cosine Functions

The Product Rule

The Quotient Rule

Derivatives of Tangent and Reciprocal Trig Functions

Unit 3: Differentiation of Composite, Implicit & Inverse Functions

Differentiation of Composite & Inverse Functions

The Chain Rule

The Inverse Function Theorem

Derivatives of Inverse Trigonometric Functions

Higher-Order Derivatives

Summary of Differentiation

Selecting Procedures for Calculating Derivatives

Unit 4: Contextual Applications of Differentiation

Rates of Change & Related Rates

Meaning of a Derivative in Context

Motion in a Straight Line

Related Rates

Linearization

Approximating Values of a Function

L'Hospital's Rule

L'Hospital's Rule

Unit 5: Analytical Applications of Differentiation

Graphs of Functions & Their Derivatives

Mean Value Theorem

Extreme Value Theorem

Critical Points

Increasing & Decreasing Functions

First Derivative Test for Local Extrema

Candidates Test for Global Extrema

Concavity of Functions

Second Derivative Test for Local Extrema

Graphs of f, f' & f''

Optimization Problems

Behaviors of Implicit Relations

Second Derivatives of Implicit Functions

Critical Points of Implicit Relations

Unit 6: Integration & Accumulation of Change

Integration & Antiderivatives

Indefinite Integrals

Derivatives & Antiderivatives

Indefinite Integral Rules

Constant of Integration