Instantaneous Rate of Change (College Board AP® Calculus BC): Revision Note

Instantaneous rate of change

What is the instantaneous rate of change?

• The instantaneous rate of change is the slope of a graph at a specific point, rather than between two points

How do I find the instantaneous rate of change?

• Consider the graph of a function $f$ with two points on the graph, $A$ and $B$

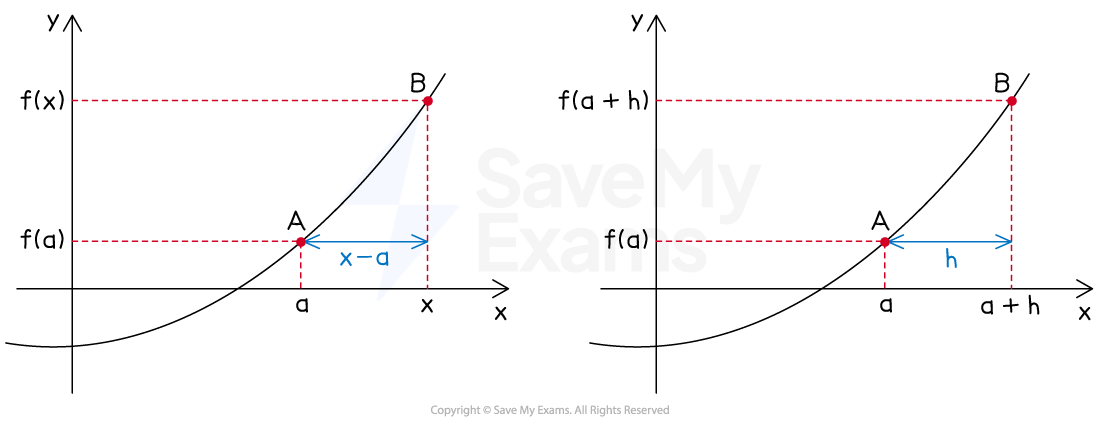
• Using the labeling on the left,

  • the average rate of change from $A$ to $B$ can be written as $\frac{f\left(x\right)-f\left(a\right)}{x-a}$

• Using the labeling on the right,

  • the average rate of change from $A$ to $B$ can be written as $\frac{f\left(a+h\right)-f\left(a\right)}{h}$

• To find the instantaneous rate of change, consider finding the slope of the secant line between $A$ and $B$ as point $B$ moves closer to point $A$

  • As the distance between the two points becomes smaller, the slope will become a more accurate estimate for the instantaneous rate of change at $x=a$

  • Therefore the instantaneous rate of change will be the limit as this distance tends to zero

• The instantaneous rate of change at $x=a$ can be written as

  • $\underset{x\to a}{\lim }\frac{f\left(x\right)-f\left(a\right)}{x-a}$ or

  • $\underset{h\to 0}{\lim }\frac{f\left(a+h\right)-f\left(a\right)}{h}$

• These only give a valid answer if the relevant limit exists

• They are both equivalent forms of the definition of the derivative of the function at $x=a$, denoted by $f^{\text{'}}\left(a\right)$