Average Rate of Change (College Board AP® Calculus BC): Study Guide

Average rate of change

What is the average rate of change?

• The average rate of change between two points on a graph, is the slope of the line segment joining the two points

• The slope is equal to the change in -values, divided by the change in -values

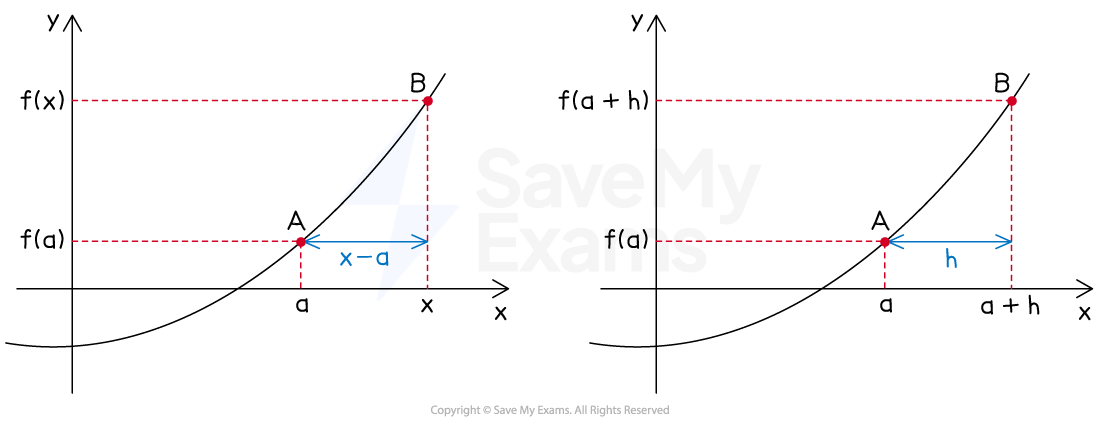
• The average rate of change between and is therefore

  • 

• This means that the average rate of change is undefined at a point where the change in is zero for a given change in

How can I write the average rate of change using function notation?

• For a function ,

  • a point with -coordinate will have -coordinate

  • a point with -coordinate will have -coordinate

• The average rate of change can therefore be written as

  • 

• If an -coordinate of is used for the first point, and the second point lies units to the right,

  • the second point will have an -coordinate of

• The average rate of change can therefore also be written as

  •