Study GuidesExam QuestionsPast Papers
AP®MathsCollege BoardCalculus BCStudy GuidesUnit 8: Applications of IntegrationVolumes with Cross SectionsTriangles as Cross Sections

Triangles as Cross Sections (College Board AP® Calculus BC): Study Guide

Roger B

Written by: Roger B

Reviewed by: Dan Finlay

Updated on

College Board Calculus BCCollege Board Calculus AB

Volumes with cross sections as triangles

How can I find the volume of a solid with a triangular cross section?

  • Use the basic concept

    • If the area of the cross section of a solid is given by A open parentheses x close parentheses

      • and A open parentheses x close parentheses is continuous on open square brackets a comma space b close square brackets

    • Then the volume of the corresponding solid from x equals a to x equals b is

      • Volume equals integral subscript a superscript b A open parentheses x close parentheses space d x

  • You may need to create the cross sectional area function A open parentheses x close parentheses based on information provided

    • For example A open parentheses x close parentheses may depend on the values of another function (or functions) given to you in the question

  • Remember that the area of a triangle is

    • Area equals 1 half cross times base cross times perpendicular space height

Worked Example

Let R be the region enclosed by the graph of f open parentheses x close parentheses equals square root of 4 minus x end root and the x- and y-axes, as shown in the figure below.

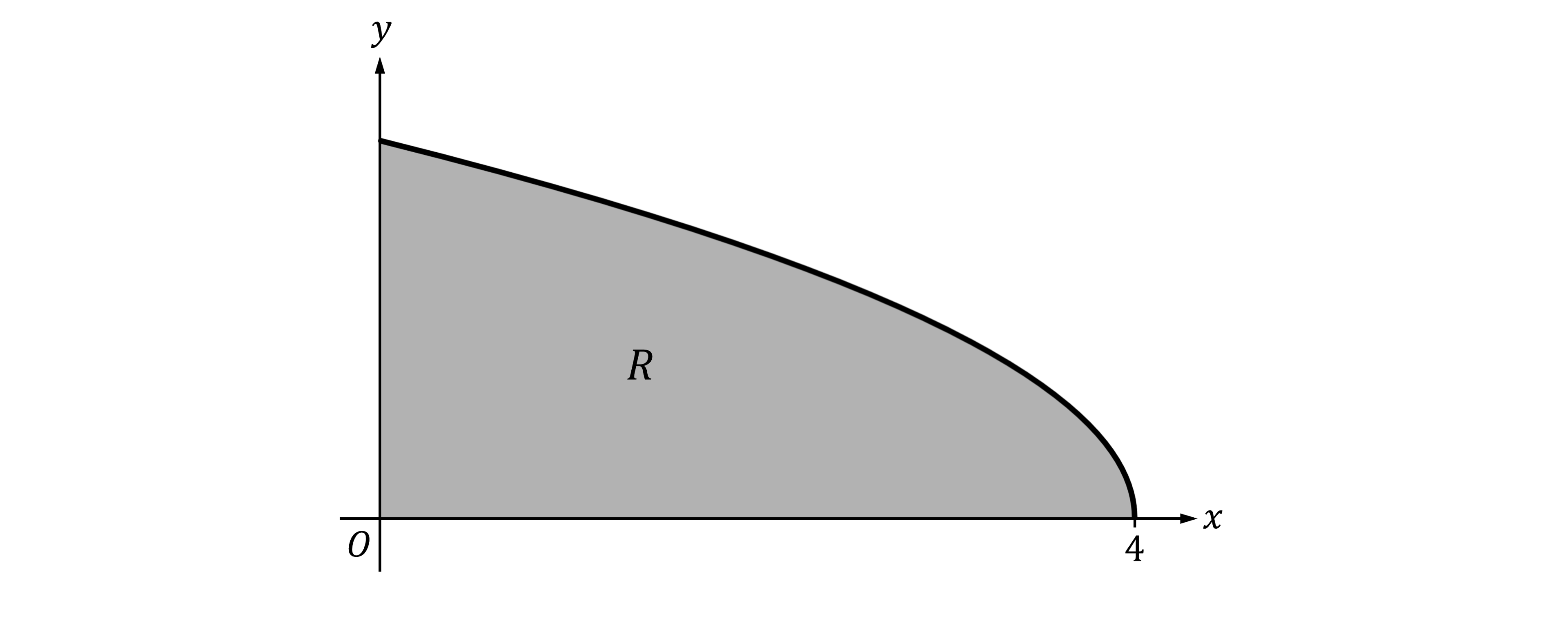
A shaded region labeled "R" is enclosed by the x-axis, the y-axis and the curve y=sqrt(4-x) from x=0 to x=4

Region R is the base of a solid. For the solid, at each x the cross section perpendicular to the x-axis is an equilateral triangle. Find the volume of the solid.

Answer:

Use Volume equals integral subscript a superscript b A open parentheses x close parentheses space d x

Use Pythagoras' theorem to work out the relevant lengths in the cross section

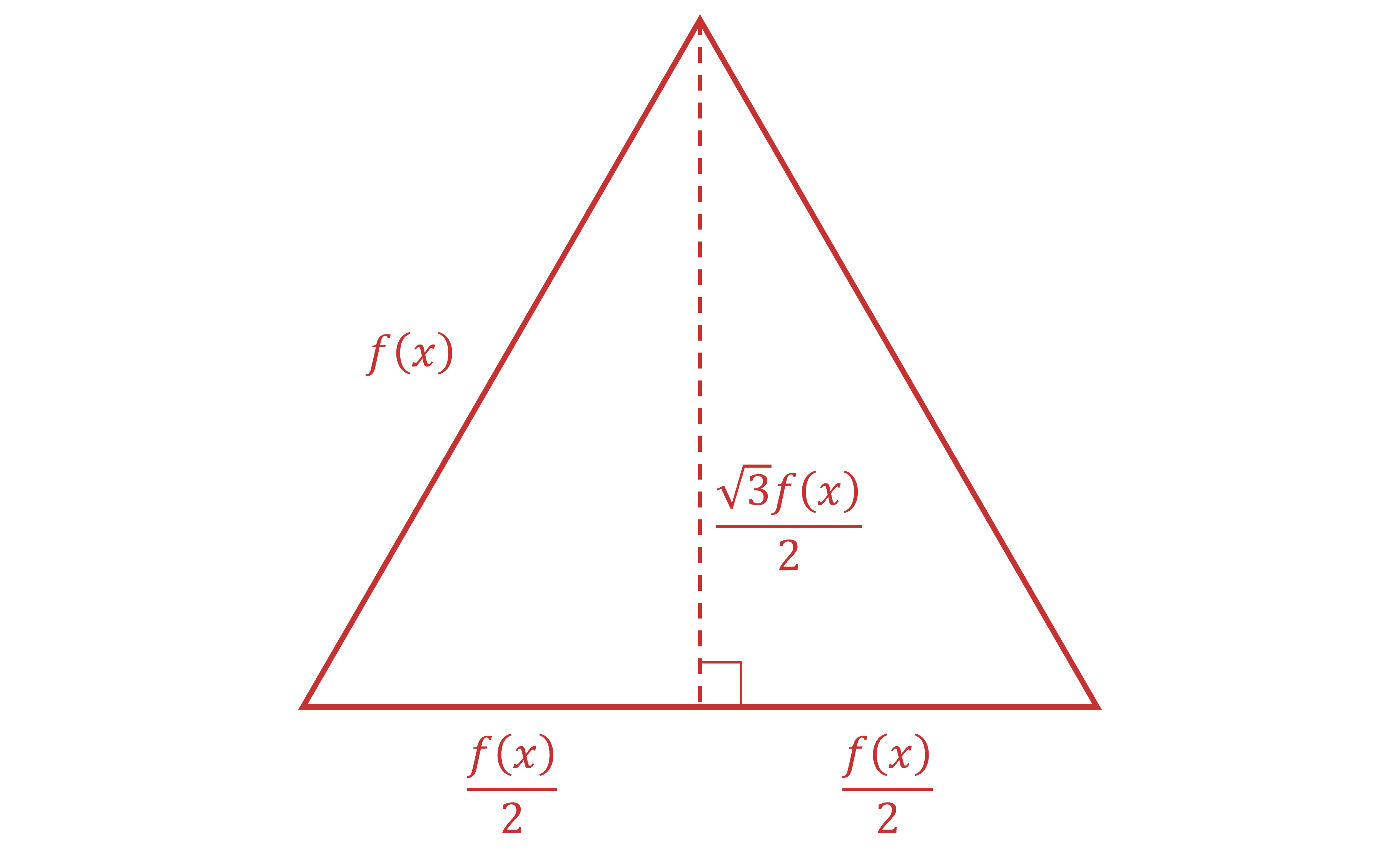
Equilateral triangle with side lengths of f(x), showing that the perpendicular height of the triangle is (sqrt(3)/2) * f(x)

height equals square root of open parentheses f open parentheses x close parentheses close parentheses squared minus open parentheses fraction numerator f open parentheses x close parentheses over denominator 2 end fraction close parentheses squared end root equals fraction numerator square root of 3 over denominator 2 end fraction f open parentheses x close parentheses

At each x the cross-sectional area is 1 half cross times base cross times height

A open parentheses x close parentheses equals 1 half times f open parentheses x close parentheses times fraction numerator square root of 3 over denominator 2 end fraction f open parentheses x close parentheses equals fraction numerator square root of 3 over denominator 4 end fraction open parentheses f open parentheses x close parentheses close parentheses squared

Now the volume integral can be used

table row Volume equals cell integral subscript 0 superscript 4 fraction numerator square root of 3 over denominator 4 end fraction open parentheses square root of 4 minus x end root space close parentheses squared space d x end cell row blank equals cell fraction numerator square root of 3 over denominator 4 end fraction integral subscript 0 superscript 4 open parentheses 4 minus x close parentheses space d x end cell row blank equals cell fraction numerator square root of 3 over denominator 4 end fraction open square brackets 4 x minus 1 half x squared close square brackets subscript 0 superscript 4 end cell row blank equals cell fraction numerator square root of 3 over denominator 4 end fraction open parentheses open parentheses 4 open parentheses 4 close parentheses minus 1 half open parentheses 4 close parentheses squared close parentheses minus open parentheses 0 close parentheses close parentheses end cell row blank equals cell 2 square root of 3 end cell row blank equals cell 3.464101... end cell end table

The question doesn't specify units, so the units of volume will be units cubed

3.464 units3 (to 3 decimal places)

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Did this page help you?

    Previous:Rectangles as Cross SectionsNext:Semicircles as Cross Sections
    Author
    Reviewer
    Roger B

    Author: Roger B

    Expertise: Maths

    Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.

    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.