Study GuidesExam QuestionsPast Papers
AP®MathsCollege BoardCalculus BCStudy GuidesUnit 8: Applications of IntegrationVolumes of RevolutionWasher Method Around Other Axes

Washer Method Around Other Axes (College Board AP® Calculus BC): Study Guide

Roger B

Written by: Roger B

Reviewed by: Dan Finlay

Updated on

College Board Calculus BCCollege Board Calculus AB

Volume with washer method revolving around other axes

How can I use the washer method to calculate a volume of revolution around a line parallel to the x-axis?

  • Let f and g be continuous functions such that open vertical bar f open parentheses x close parentheses minus k close vertical bar less than open vertical bar g open parentheses x close parentheses minus k close vertical bar on the interval open square brackets a comma space b close square brackets

    • I.e. f open parentheses x close parentheses is closer to the horizontal line y equals k than g open parentheses x close parentheses is on that interval

  • If the region bounded by

    • the curves y subscript 1 equals f open parentheses x close parentheses and y subscript 2 equals g open parentheses x close parentheses

    • between x equals a and x equals b

  • is rotated 2 pi radians left parenthesis 360 degree right parenthesis around the line y equals k, then the volume of revolution is

    •  V equals pi integral subscript a superscript b open parentheses open parentheses y subscript 2 minus k close parentheses squared minus open parentheses y subscript 1 minus k close parentheses squared close parentheses space italic d x

      • Note that y subscript 1 and y subscript 2 are both functions of x

  • Make sure that y subscript 2 is the curve further away from the line y equals k

    • and y subscript 1 is the curve closer to the line y equals k

      • If the curves 'swap places' over the interval

        • then split the calculation into separate integrals

  • If x equals a and x equals b are not stated in a question, these boundaries could involve

    • the y-axis (x equals 0)

    • and/or point(s) of intersection of the two curves

Worked Example

Let R be the region enclosed by the graphs of f open parentheses x close parentheses equals 1 fourth x squared and g open parentheses x close parentheses equals x, as shown in the figure below.

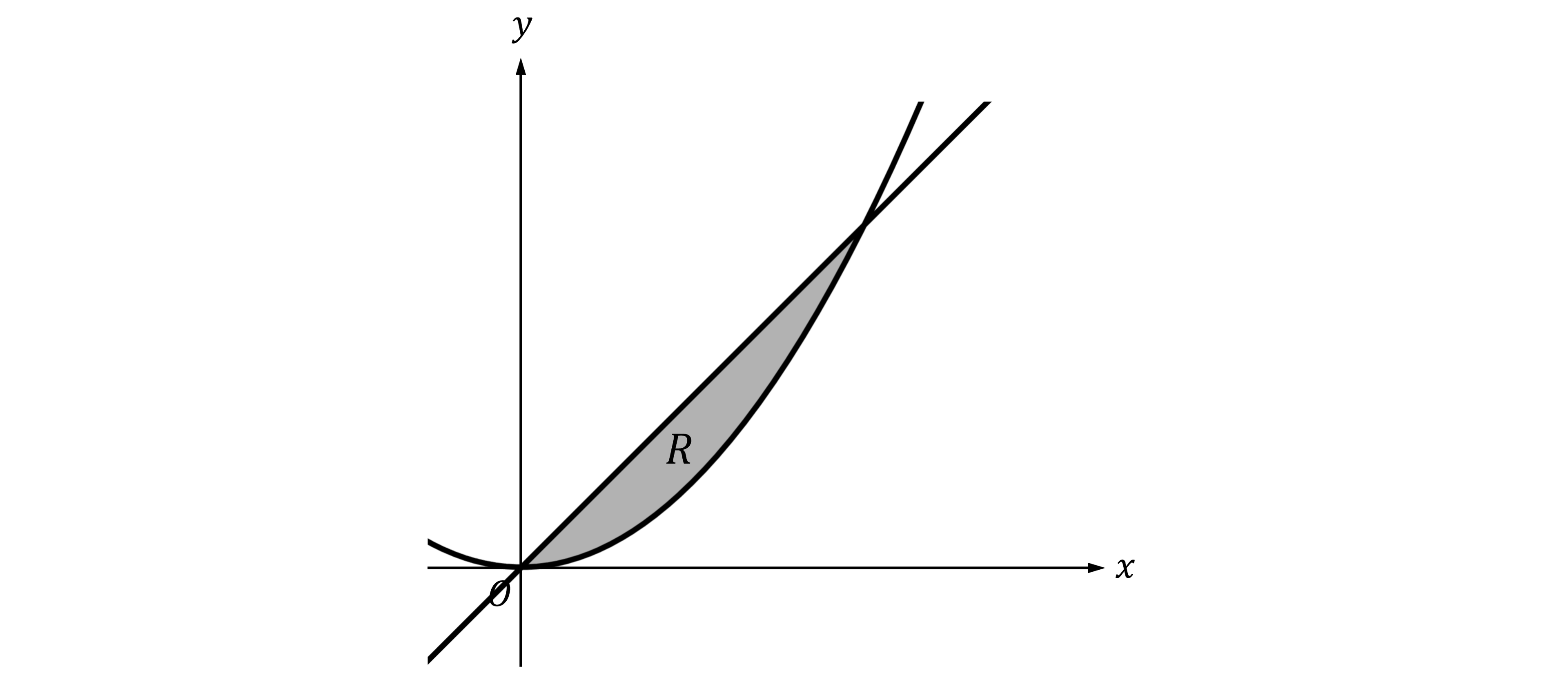
Graph showing a shaded region R enclosed by the curves y=x and y=x^2/4

Find the volume of the solid generated when R is rotated about the horizontal line y equals 5.

Answer:

Use V equals pi integral subscript a superscript b open parentheses open parentheses y subscript 2 minus k close parentheses squared minus open parentheses y subscript 1 minus k close parentheses squared close parentheses space italic d x

To find a and b, solve f open parentheses x close parentheses equals g open parentheses x close parentheses to find the x-coordinates of the points of intersection of the two curves

table row cell 1 fourth x squared end cell equals x row cell x squared minus 4 x end cell equals 0 row cell x open parentheses x minus 4 close parentheses end cell equals 0 end table

table row x equals cell 0 space space or space space x equals 4 end cell end table

So a equals 0 and b equals 4

At x equals 4, f open parentheses 4 close parentheses equals g open parentheses 4 close parentheses equals 4, so y equals 5 is above region R in the diagram

Therefore g open parentheses x close parentheses equals x is the function closest to the line y equals 5, so use y subscript 1 equals x and y subscript 2 equals 1 fourth x squared

Set up and solve the integral

table row V equals cell pi integral subscript 0 superscript 4 open parentheses open parentheses 1 fourth x squared minus 5 close parentheses squared minus open parentheses x minus 5 close parentheses squared close parentheses space italic d x end cell row blank equals cell pi integral subscript 0 superscript 4 open parentheses 1 over 16 x to the power of 4 minus 5 over 2 x squared plus 25 minus open parentheses x squared minus 10 x plus 25 close parentheses close parentheses space italic d x end cell row blank equals cell pi integral subscript 0 superscript 4 open parentheses 1 over 16 x to the power of 4 minus 7 over 2 x squared plus 10 x close parentheses space italic d x end cell row blank equals cell pi open square brackets 1 over 80 x to the power of 5 minus 7 over 6 x cubed plus 5 x squared close square brackets subscript 0 superscript 4 end cell row blank equals cell pi open parentheses open parentheses 1 over 80 open parentheses 4 close parentheses to the power of 5 minus 7 over 6 open parentheses 4 close parentheses cubed plus 5 open parentheses 4 close parentheses squared close parentheses minus 0 close parentheses end cell row blank equals cell fraction numerator 272 pi over denominator 15 end fraction end cell row blank equals cell 56.967546... end cell end table

The question doesn't specify units, so the units of volume will be units cubed

56.968 units3 (to 3 decimal places)

How can I use the washer method to calculate a volume of revolution around a line parallel to the y-axis?

  • Let f and g be continuous functions of y such that open vertical bar f open parentheses y close parentheses minus k close vertical bar less than open vertical bar g open parentheses y close parentheses minus k close vertical bar on the interval open square brackets a comma space b close square brackets

    • I.e. f open parentheses y close parentheses is closer to the vertical line x equals k than g open parentheses y close parentheses is on that interval

  • If the region bounded by

    • the curves x subscript 1 equals f open parentheses y close parentheses and x subscript 2 equals g open parentheses y close parentheses

    • between y equals a and y equals b

  • is rotated 2 pi radians left parenthesis 360 degree right parenthesis around the line x equals k, then the volume of revolution is

    •  V equals pi integral subscript a superscript b open parentheses open parentheses x subscript 2 minus k close parentheses squared minus open parentheses x subscript 1 minus k close parentheses squared close parentheses space italic d y

      • Note that x subscript 1 and x subscript 2 are both functions of y

      • If the functions are given as functions of x

        • e.g y equals p open parentheses x close parentheses and y equals q open parentheses x close parentheses

      • then you will need to rewrite them as functions of y

        • See the Worked Example

      • Also note that the integration is done with respect to y

  • Make sure that x subscript 2 is the curve further away from the line x equals k

    • and x subscript 1 is the curve closer to the line x equals k

      • If the curves 'swap places' over the interval

        • then split the calculation into separate integrals

  • If y equals a and y equals b are not stated in a question, these boundaries could involve

    • the x-axis (y equals 0)

    • and/or point(s) of intersection of the two curves

Worked Example

Let R be the region enclosed by the graphs of f open parentheses x close parentheses equals 1 fourth x squared and g open parentheses x close parentheses equals x, as shown in the figure below.

Graph showing a shaded region R enclosed by the curves y=x and y=x^2/4

Find the volume of the solid generated when R is rotated about the vertical line x equals negative 2.

Answer:

Use V equals pi integral subscript a superscript b open parentheses open parentheses x subscript 2 minus k close parentheses squared minus open parentheses x subscript 1 minus k close parentheses squared close parentheses space italic d y

First rewrite the functions as functions of y

table row y equals cell f open parentheses x close parentheses end cell row y equals cell 1 fourth x squared end cell row cell x squared end cell equals cell 4 y end cell row x equals cell 2 square root of y end cell end table

Note that x equals 2 square root of y is used instead of x equals negative 2 square root of y because it can be seen from the graph that the x values of the relevant part of the curve are positive

table row y equals cell g open parentheses x close parentheses end cell row y equals x row x equals y end table

The line x equals negative 2 is to the left of region R in the diagram

Therefore g open parentheses x close parentheses equals x is the function closest to the line x equals negative 2, so use x subscript 1 equals y and x subscript 2 equals 2 square root of y

To find a and b, solve x subscript 1 open parentheses y close parentheses equals x subscript 2 open parentheses y close parentheses to find the y-coordinates of the points of intersection of the two curves

table row y equals cell 2 square root of y end cell row cell y squared end cell equals cell 4 y end cell row cell y squared minus 4 y end cell equals 0 row cell y open parentheses y minus 4 close parentheses end cell equals 0 end table

table row y equals cell 0 space space or space space y equals 4 end cell end table

So a equals 0 and b equals 4

Set up and solve the integral

table row V equals cell pi integral subscript 0 superscript 4 open parentheses open parentheses 2 square root of y minus open parentheses negative 2 close parentheses close parentheses squared minus open parentheses y minus open parentheses negative 2 close parentheses close parentheses squared close parentheses space italic d y end cell row blank equals cell pi integral subscript 0 superscript 4 open parentheses open parentheses 2 square root of y plus 2 close parentheses squared minus open parentheses y plus 2 close parentheses squared close parentheses space italic d y end cell row blank equals cell pi integral subscript 0 superscript 4 open parentheses 4 y plus 8 square root of y plus 4 minus open parentheses y squared plus 4 y plus 4 close parentheses close parentheses space italic d y end cell row blank equals cell pi integral subscript 0 superscript 4 open parentheses 8 square root of y minus y squared close parentheses space italic d y end cell row blank equals cell pi integral subscript 0 superscript 4 open parentheses 8 y to the power of 1 half end exponent minus y squared close parentheses space italic d y end cell row blank equals cell pi open square brackets 16 over 3 y to the power of 3 over 2 end exponent minus 1 third y cubed close square brackets subscript 0 superscript 4 end cell row blank equals cell pi open parentheses open parentheses 16 over 3 open parentheses 4 close parentheses to the power of 3 over 2 end exponent minus 1 third open parentheses 4 close parentheses cubed close parentheses minus 0 close parentheses end cell row blank equals cell fraction numerator 64 pi over denominator 3 end fraction end cell row blank equals cell 67.020643... end cell end table

The question doesn't specify units, so the units of volume will be units cubed

67.021 units3 (to 3 decimal places)

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Did this page help you?

    Previous:Washer Method Around the y-AxisNext:Defining Parametric Equations
    Author
    Reviewer
    Roger B

    Author: Roger B

    Expertise: Maths

    Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.

    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.