Distance & Speed (College Board AP® Calculus BC) : Study Guide
Distance & speed as integrals
How are distance and speed different from displacement and velocity?
Distance is the magnitude of displacement,
Travelling 3 meters forwards and 3 meters backwards is a distance of 6 meters traveled
But the displacement after this motion would be zero
Speed is the magnitude of velocity,
Travelling at 10 meters per second forward is a velocity of +10 meters per second
Travelling at 10 meters per second backwards is a velocity of -10 meters per second
In both these cases however, the speed is 10 meters per second
How do I find a distance using integration?
The total distance traveled between times
and
is given by
This is because even when the object is moving backwards (negative velocity), the distance traveled is increasing (whilst the displacement is decreasing)
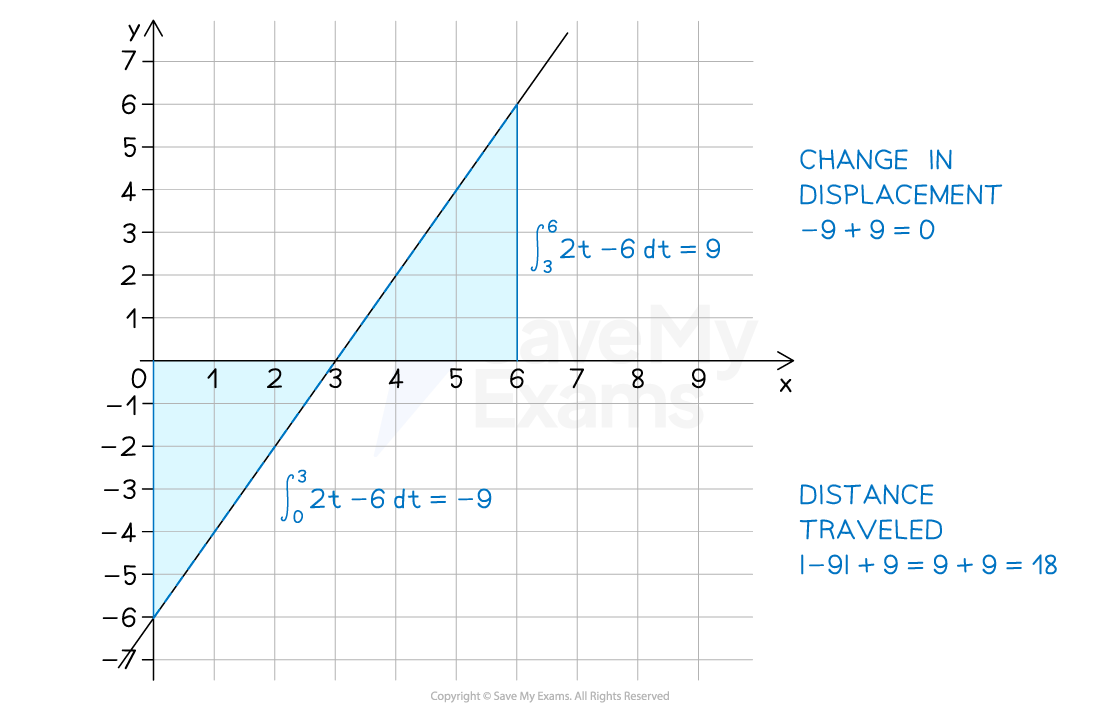
Consider a simple example, where
To find the change in displacement between
and
we would calculate
, which has a value of zero
This is because
and
To find the distance traveled between
and
we would calculate
, which has a value of 18
You could find this on your calculator directly, using the "absolute" (i.e. absolute value or modulus) function
Alternatively you can sketch a velocity-time graph to find where the area under the graph would be negative and positive, and split the calculation into two integrals
Then make any negative integrals positive
How does using speed rather than velocity affect calculations?
Pay close attention to whether a question refers to speed or velocity
This is important when a calculation involving velocity "goes through zero"
E.g. If a particle with velocity 5 meters per second decreases its velocity by 20 meters per second
Its new velocity is -15 meters per second
However its new speed is 15 meters per second
If a particle with velocity -8 meters per second, increases its velocity by 10 meters per second
Its new velocity is 2 meters per second
Its new speed is also 2 meters per second
But, it has changed from a speed of 8 meters per second, to a speed of 2 meters per second
So it is true to say its speed has decreased by 6 meters per second
To find a change in velocity, leading to a calculation similar to above, you may need to integrate an expression for acceleration
Worked Example
The acceleration of a particle over the interval is described by the function
where
is measured in feet per second and
is measured in seconds.
At , the particle is at rest.
(a) Calculate the change in speed between and
. State if this is an increase or decrease.
Answer
Start by finding an expression for the velocity by integrating the expression for the acceleration
To find the constant of integration, substitute in a known velocity at a point in time
We were told in the question the initial velocity is zero, so when
Find the velocities at and
Consider these results in terms of speeds rather than velocities
Speed at is
Speed at is 3
Therefore the speed has increased
Increase in speed =
Round to 3 decimal places
The speed has increased by 0.879 feet per second
(b) Find the total distance traveled by the particle between and
.
Answer:
Distance traveled will be
On a calculator question you could use your calculator to evaluate this
If doing it by hand, you have to be careful with negative areas (i.e. intervals where )
The graph of will intersect the horizontal axis at
, so the area between
and
will have a positive and a negative portion
Split the integral into multiple parts, with the horizontal axis intercepts as the boundaries
Find the total distance travelled by considering the absolute values of the changes in displacement
I.e.
6 + 12 = 18
Distance of 18 feet traveled between and

You've read 0 of your 5 free study guides this week
Sign up now. It’s free!
Did this page help you?

