Study GuidesExam QuestionsPast Papers
AP®MathsCollege BoardCalculus ABStudy GuidesUnit 1: Limits & ContinuityLimitsInfinite Limits & Limits at Infinity

Infinite Limits & Limits at Infinity (College Board AP® Calculus AB)

Study Guide

Roger B

Written by: Roger B

Reviewed by: Dan Finlay

Infinite limits

What do we mean by an infinite limit?

  • Sometimes the values of a function become unbounded (in the positive or negative direction) as x approaches a certain value

    • In such cases we talk about the function having an infinite limit at that value of x

    • For functions defined as fractions or quotients, this happens when the denominator becomes 0 for some value(s) of x

  • If the value of a function f increases without bound as x approaches some value c, then we write limit as x rightwards arrow c of f open parentheses x close parentheses equals infinity.

    • For example, limit as x rightwards arrow 0 of 1 over x squared equals infinity

  • If the value of a function f decreases without bound as x approaches some value c, then we write limit as x rightwards arrow c of f open parentheses x close parentheses equals negative infinity.

    • For example, limit as x rightwards arrow 0 of open parentheses negative 1 over x squared close parentheses equals negative infinity

  • It is possible for the one-sided limits to be different

    • For example, limit as x rightwards arrow 0 to the power of plus of 1 over x equals infinity

    • But limit as x rightwards arrow 0 to the power of minus of 1 over x equals negative infinity

What is the connection between infinite limits and vertical asymptotes?

  • When a function has an infinite limit at a point, its graph has a vertical asymptote at that value of x

    • This is a vertical line that the graph gets closer and closer to (but never touches or intersects) as x approaches that value

  • Vertical asymptotes on the graph of a function are an indication that it has an infinite limit at that x value

    • Conversely, identifying infinite limits for a function lets you identify where the graph of the function has vertical asymptotes

Worked Example

The figure below shows the graph of the function f defined by f open parentheses x close parentheses equals 1 over open parentheses x minus 2 close parentheses squared. The dashed line is a vertical asymptote of the graph.

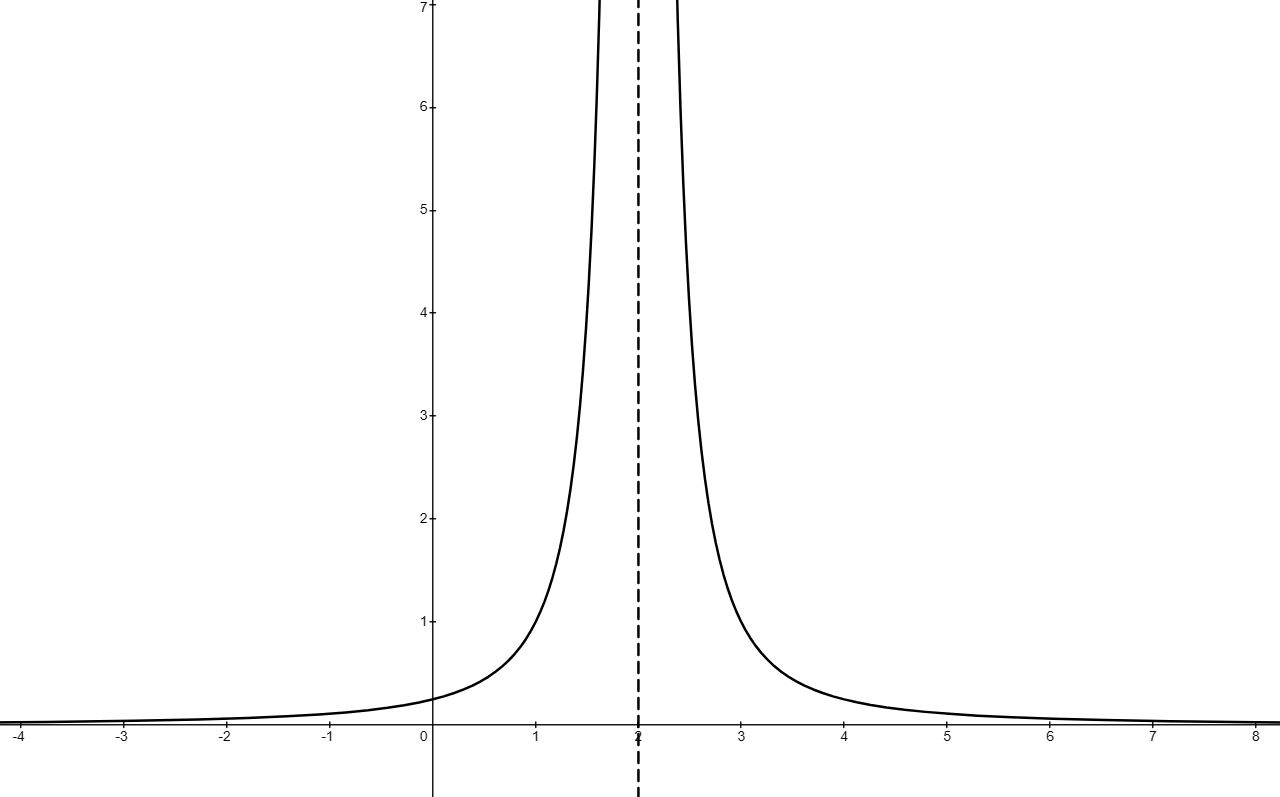
Graph of y=1/(x-2)^2, including vertical asymptote at x=2

What is limit as x rightwards arrow 2 of f open parentheses x close parentheses?

Answer:

f(x) is positive for all values of x except 2

As x approaches 2, the denominator gets closer and closer to zero, and the value of the function increases without bound (i.e. gets bigger and bigger in the positive direction)

limit as x rightwards arrow 2 of f open parentheses x close parentheses equals infinity

Limits at infinity

What do we mean by a limit at infinity?

  • Sometimes we are interested in the value of a function as x increases or decreases without bound

    • In such cases we talk about the limit at (positive or negative) infinity of the function

  • When considering the behavior of a function f as x increases without bound (i.e. becomes infinitely big in the positive direction) we write limit as x rightwards arrow infinity of f open parentheses x close parentheses

  • When considering the behavior of a function f as x decreases without bound (i.e. becomes infinitely big in the negative direction) we write limit as x rightwards arrow negative infinity of f open parentheses x close parentheses

  • For some functions, their values as x approaches positive or negative infinity also become unbounded

    • For example, limit as x rightwards arrow infinity of open parentheses x plus 1 close parentheses equals infinity and limit as x rightwards arrow negative infinity of open parentheses x plus 1 close parentheses equals negative infinity

  • But for other functions, their values settle down towards (but never quite reach) a fixed value

    • For example, limit as x rightwards arrow infinity of open parentheses 1 over x plus 1 close parentheses equals 1 and limit as x rightwards arrow negative infinity of open parentheses 1 over x plus 1 close parentheses equals 1

      • Because 1 over x gets closer and closer to zero as x becomes in large in either the positive or negative directions

What is the connection between limits at infinity and horizontal asymptotes?

  • When a function has a finite limit at infinity, its graph has a horizontal asymptote at that value of y

    • This is a horizontal line that the graph gets closer and closer to (but in general never touches or intersects) as x becomes unbounded in the indicated direction

      • For example if limit as x rightwards arrow infinity of f open parentheses x close parentheses equals 3, then the graph of f will have a horizontal asymptote at y=3

    • The graph becomes 'more and more like' the asymptote as x becomes unbounded

  • Horizontal asymptotes on the graph of a function are an indication that the function has a finite limit at infinity

    • Conversely, identifying finite limits at infinity for a function lets you identify where the graph of the function has horizontal asymptotes

Examiner Tips and Tricks

If an exam question is using a function to model a real-world scenario, be sure to interpret any limits at infinity in the context of the question.

Worked Example

The figure below shows the graph of the function P defined by P open parentheses t close parentheses equals 4 minus fraction numerator 3 over denominator t plus 1 end fraction comma space space t greater or equal than 0.

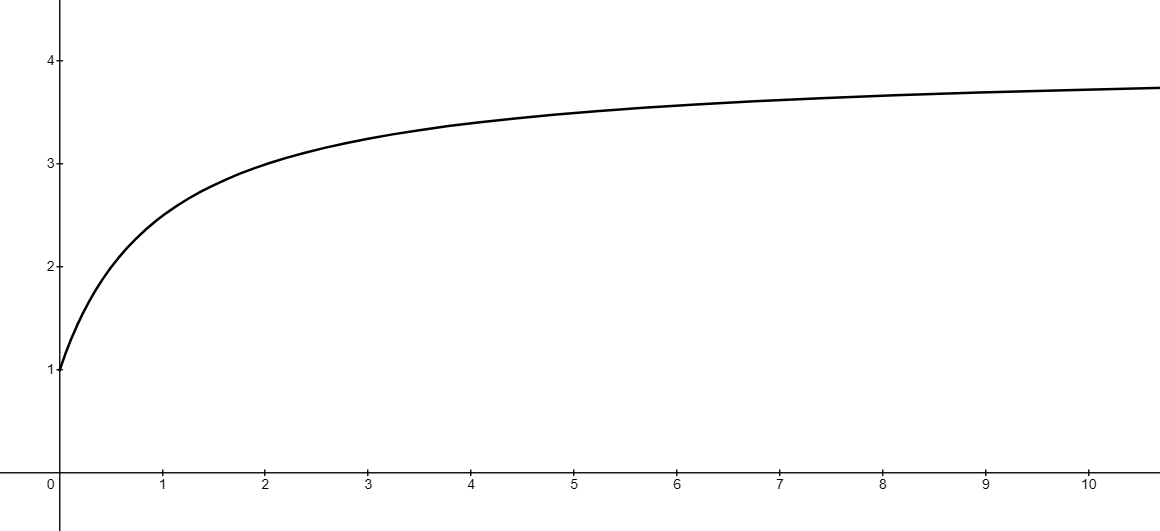
Graph of P(t)=4-3/(t+1) for t>=0

P is being used to model the population (in hundreds) of squirrels in a particular area of woodland at time t years after the beginning of a study.

(a) Find limit as t rightwards arrow infinity of P open parentheses t close parentheses.

Answer:

As t gets bigger and bigger in the positive direction, the fraction fraction numerator 3 over denominator t plus 1 end fraction will become closer and closer to zero

Therefore the function will get closer and closer to 4 (without ever quite reaching 4)

limit as t rightwards arrow infinity of P open parentheses t close parentheses equals 4

(b) Interpret your answer for part (a) in the context of this problem.

Answer:

Connect the limit in part (a) to the context given in the question

Over time the population of squirrels in the woodland will approach 400.

Last updated:

You've read 0 of your 5 free study guides this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Definition of a LimitNext:Properties of Limits
    Author
    Reviewer
    Roger B

    Author: Roger B

    Expertise: Maths

    Roger's teaching experience stretches all the way back to 1992, and in that time he has taught students at all levels between Year 7 and university undergraduate. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you.

    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.