Estimating the Derivative at a Point (College Board AP® Calculus AB)

The coordinates of the points that lie on the graph of a function can be used to estimate the derivative at a point

Recall that the derivative of at the point where , denoted as ,

• is equal to the slope of the tangent to the graph of at

To approximate the slope of the tangent to the graph of at :

• Find the slope of line segments joining nearby points that lie on the graph

The function must be continuous and differentiable within the relevant interval for this method to be valid

Consider the graph of below, where points are labeled with their coordinates

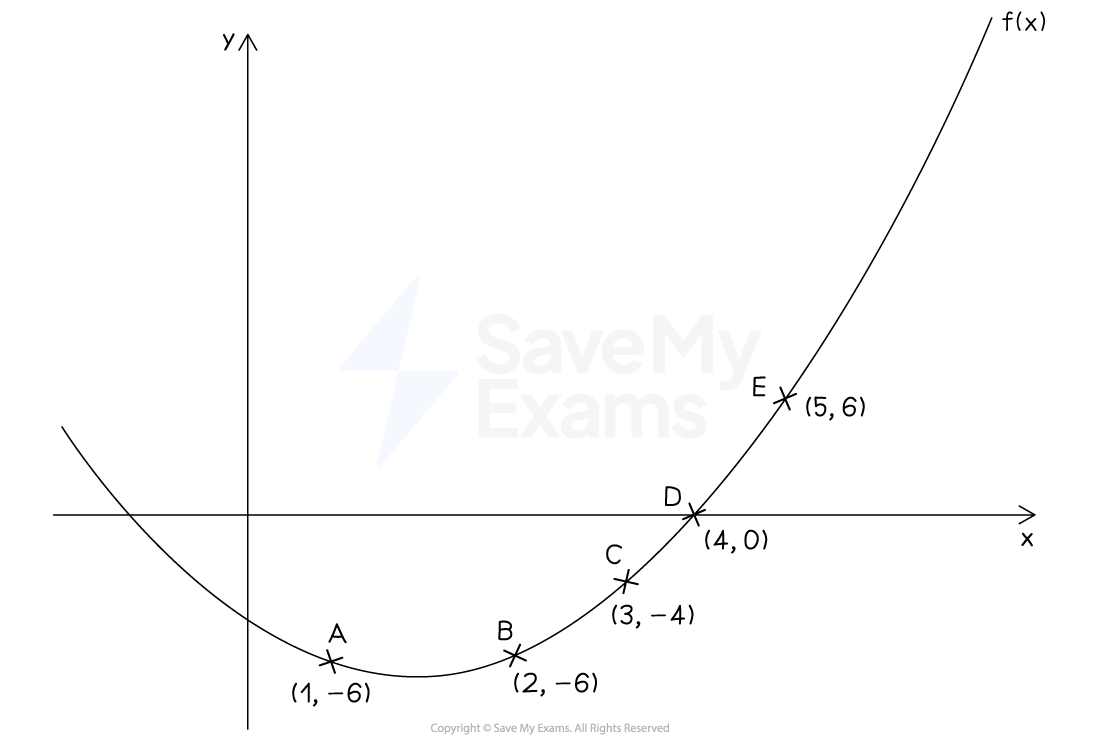
To estimate the derivative at point we can find the slope of nearby line segments

Finding the slope between and

Finding the slope between and

Finding the slope between and

Finding the slope between and

Depending on which line segment is used, an approximation for the derivative of at is 2, 3, or 4

A similar method can be used to estimate the derivative at a point from a graph, but with a table of values instead

Recall that the derivative of at the point where , denoted as ,

• is equal to the slope of the tangent to the graph of at

To approximate the slope of the tangent to the graph of at :

• Find the slope of line segments joining nearby coordinates that lie on the graph

Consider the table of values below for the function

• is a continuous and differentiable function within this interval

• This must be true to use this method

To find an estimate for the derivative of at , i.e. to find , find the slope of line segments close to the point (7, -16)

Between (3, -24) and (7, -16)

Between (9, 0) and (7, -16)

Between (5, -24) and (9, 0)

Depending on which line segment is used, an approximation for the derivative of at is 2, 8, or 6