Disc Method Around the y-Axis (College Board AP® Calculus AB)

Revision Note

Author

Roger B

Expertise

Maths

Volume with disc method revolving around the y-axis

What is a volume of revolution around the y-axis?

This is very similar to a volume of revolution around the $x$ -axis
A solid of revolution is formed when an area bounded by a function $y = f (x)$
(and other boundary equations) is rotated $2 π$ radians $(360 °)$ around the $y$ -axis
The volume of revolution is the volume of this solid

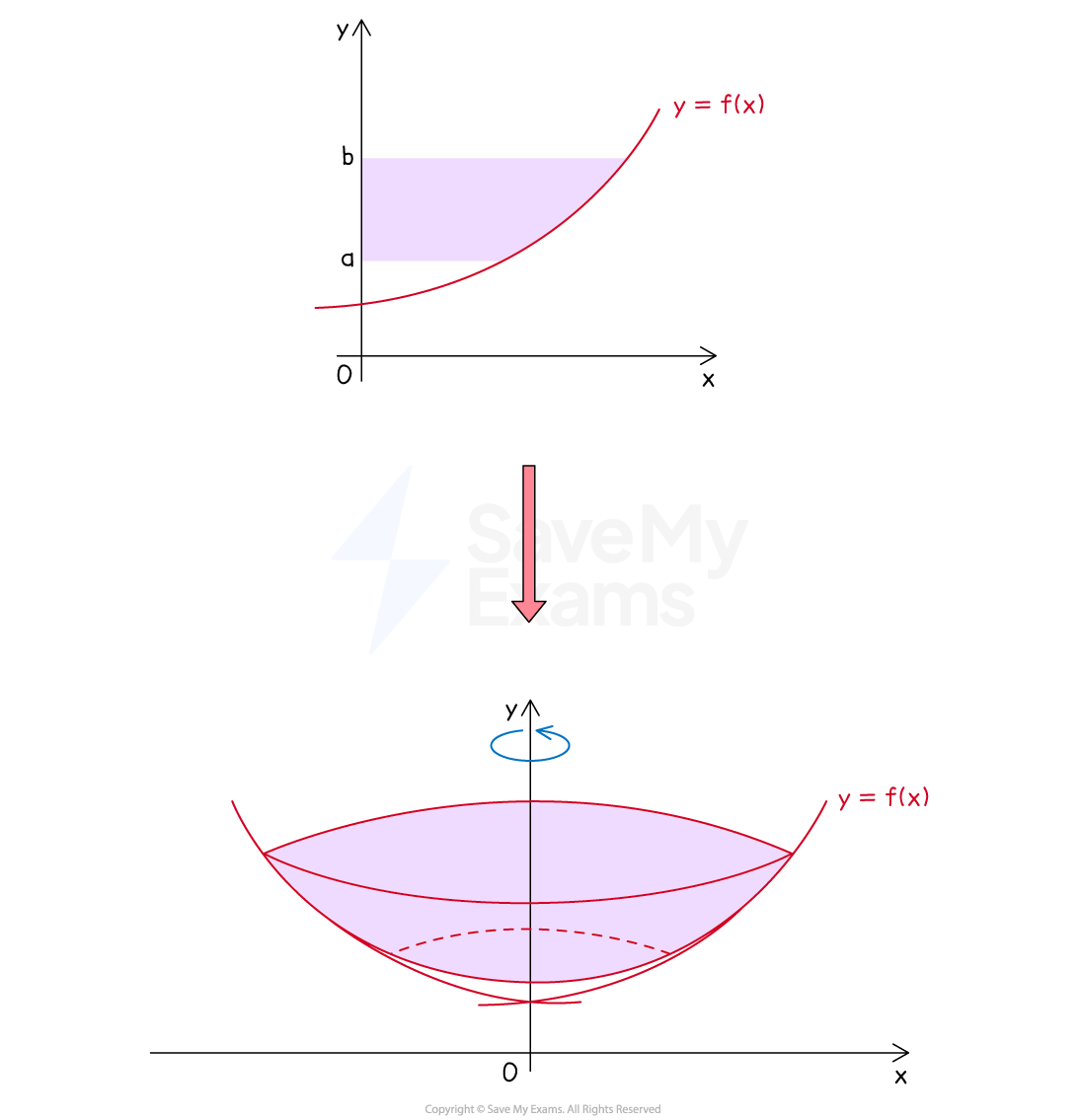
Example of a solid of revolution that is formed by rotating the area bounded by the function y=f(x), the lines y=a and y=b, and the y-axis about the y-axis

How can I use the disc method to calculate a volume of revolution around the y-axis?

For a continuous function $f$ , if the region bounded by
- the curve $y = f (x)$ and the $y$ -axis
- between $y = a$ and $y = b$
is rotated $2 π$ radians $(360 °)$ around the $y$ -axis, then the volume of revolution is
- $V = \int_{a}^{b} π x^{2} d y = π \int_{a}^{b} x^{2} d y$
  - Note that $x$ here is a function of $y$
    - This will mean rewriting $y = f (x)$ in the form $x = g (y)$
  - Also note that the integration is done with respect to $y$
If $y = a$ and $y = b$ are not stated in a question, these boundaries could involve
- the $x$ -axis ( $y = 0$ )
- and/or a $y$ -intercept of $y = f (x)$

Exam Tip

If the given function involves a square root, the problem may seem daunting

But the square root will be 'squared away' when using the Volume of Revolution formula

If a diagram is not provided, sketching the curve, limits, etc. can really help

A graphing calculator can help with this

Worked Example

Let $R$ be the region enclosed by the graph of $f (x) = \arcsin (2 x + 1)$ , the negative $x$ -axis, and the positive $y$ -axis, as shown in the figure below.

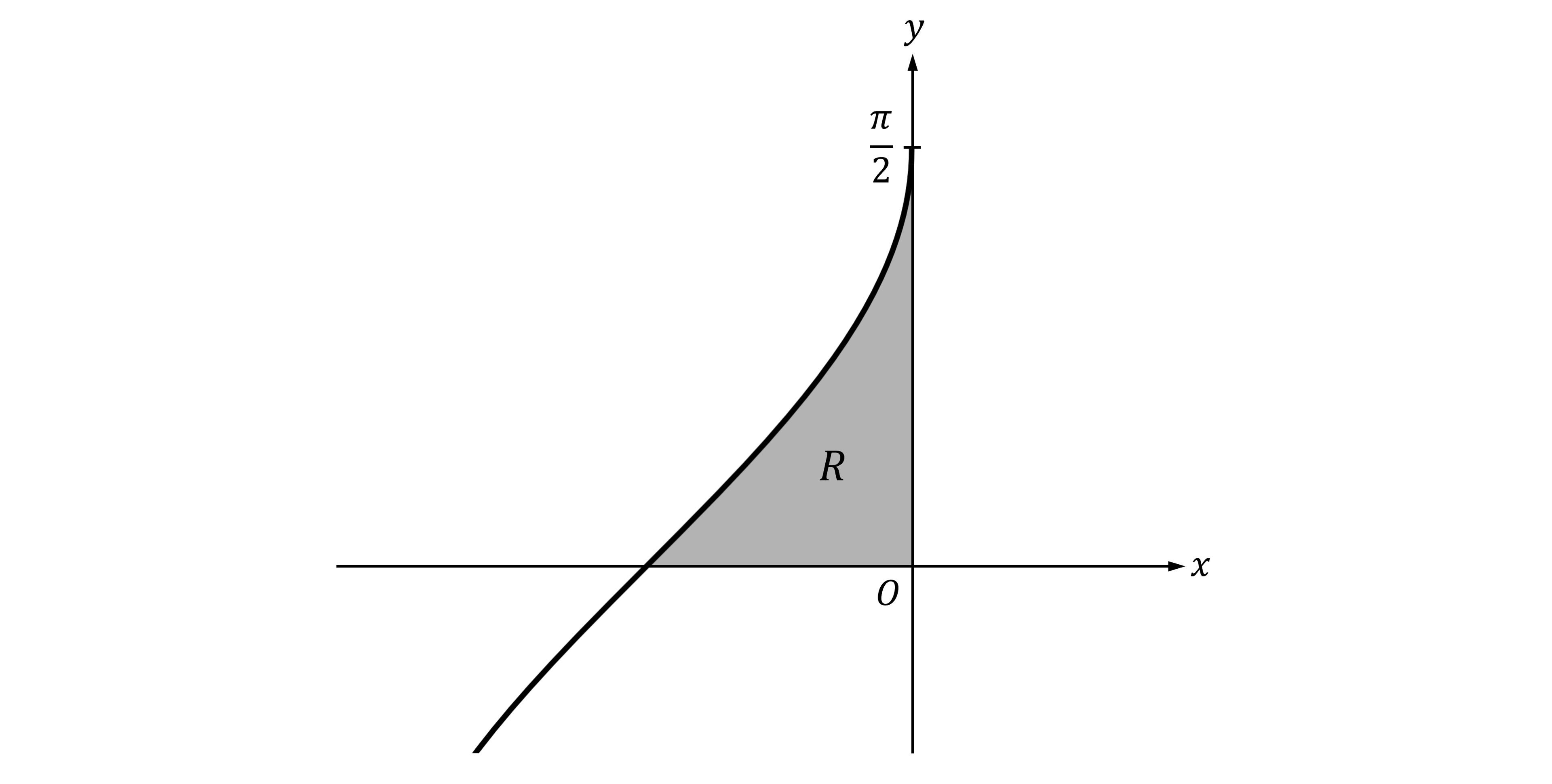
Graph showing the function y = arcsin(2x+1), shading the area (R) enclosed by the curve, the negative x-axis, and the positive y-axis

Find the volume of the solid generated when $R$ is rotated about the $y$ -axis. Give your answer correct to 3 decimal places.

Answer:

Use $V = π \int_{a}^{b} x^{2} d y$

First rewrite the function as a function of $y$

$\begin{array}{rcl} y & = & \arcsin (2 x + 1) \\ \sin y & = & 2 x + 1 \\ x & = & \frac{\sin y - 1}{2} \end{array}$

Now that can be put into the integral

Note that the integration will be along the $y$ -axis, from $y = 0$ to $y = \frac{π}{2}$

The integral can be evaluated using your calculator

$\begin{array}{rcl} V & = & π \int_{0}^{\frac{π}{2}} {(\frac{\sin y - 1}{2})}^{2} d y \\ = & 0.279754 . . . \end{array}$

The question doesn't specify units, so the units of volume will be ${units}^{3}$

0.280 units³ (to 3 decimal places)

You've read 0 of your 10 free revision notes

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Next topic

Did this page help you?