AP®MathsCollege BoardCalculus ABStudy GuidesUnit 5: Analytical Applications of DifferentiationGraphs of Functions & Their DerivativesIncreasing & Decreasing Functions

Increasing & Decreasing Functions (College Board AP® Calculus AB)

Study Guide

Test yourself
Jamie Wood

Written by: Jamie Wood

Reviewed by: Dan Finlay

Increasing & decreasing functions

How do I find where a function is increasing and decreasing?

  • The first derivative of a function, f apostrophe open parentheses x close parentheses, describes the rate of change of f open parentheses x close parentheses

    • If the rate of change is positive, the function is increasing

    • If the rate of change is negative, the function is decreasing

  • This means you can determine if a function is increasing or decreasing at a point

    • If f apostrophe open parentheses a close parentheses greater or equal than 0 then f is increasing at x equals a

    • If f apostrophe open parentheses a close parentheses less or equal than 0 then f is decreasing at x equals a

    • If f apostrophe open parentheses a close parentheses equals 0 then there is a critical point at x equals a

  • You can also find an interval where a function is increasing or decreasing

    • To find where the function is increasing,

      • Solve the inequality f apostrophe open parentheses x close parentheses greater or equal than 0

    • To find where the function is decreasing,

      • Solve the inequality f apostrophe open parentheses x close parentheses less or equal than 0

Examiner Tips and Tricks

The definitions for where a function is increasing or decreasing include the endpoints, however the scoring guidelines for exam questions often allow the point to still be awarded if the endpoints are not included.

I.e. " f open parentheses x close parentheses is increasing for 1 less or equal than x less or equal than 5 " would receive the same marks as " f open parentheses x close parentheses is increasing for 1 less than x less than 5 "

  • Sketching a graph of both f open parentheses x close parentheses and f apostrophe open parentheses x close parentheses can help to identify where a function will be increasing or decreasing

    • On the graph of f open parentheses x close parentheses,

      • An upward slope from left to right is where the function is increasing

      • A downward slope from left to right is where the function is decreasing

    • On the graph of f apostrophe open parentheses x close parentheses,

      • The portion of the graph above the x-axis is where the function is increasing

      • The portion of the graph below the x-axis is where the function is decreasing

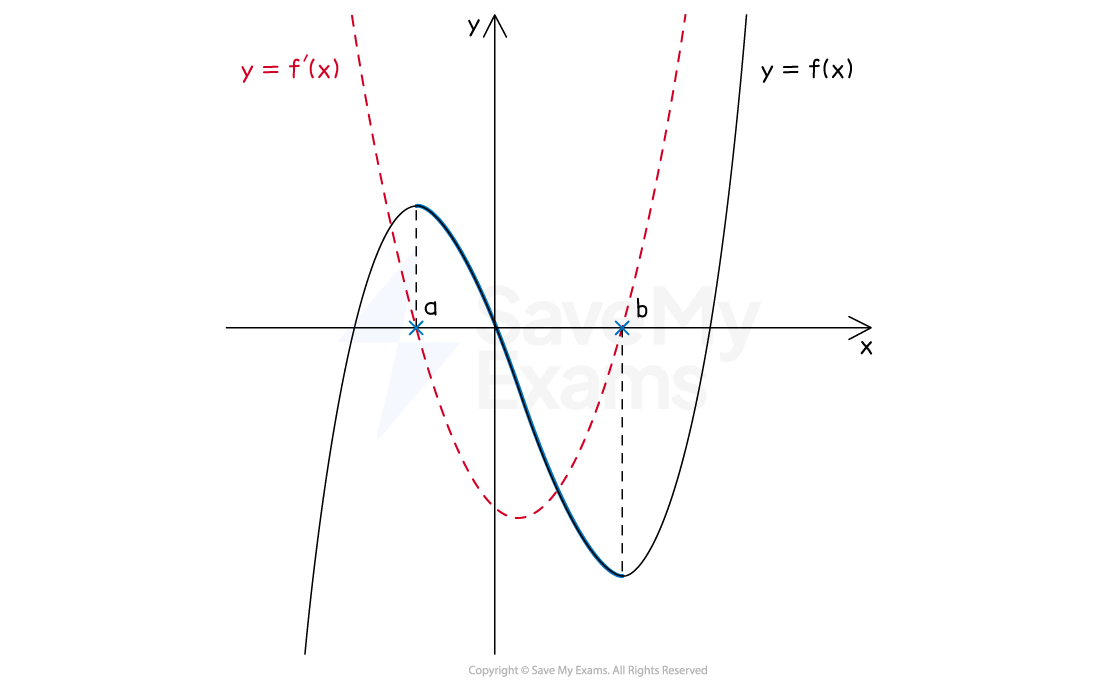
  • The diagram below shows a cubic and its derivative, a quadratic, plotted on the same graph

    • Between the critical points at a and b, the cubic is decreasing

    • Therefore the graph of the derivative is below the x-axis between a and b

Graph showing a black curve y=f(x), a red dashed curve y=f'(x). Points a and b are marked on the x-axis where f(x) changes from increasing to decreasing and vice versa
Graph of a cubic, and its derivative; a quadratic.

Worked Example

Find the interval(s) on which the graph of f open parentheses x close parentheses equals 1 fourth x to the power of 4 plus 1 third x cubed minus 3 x squared plus 4 is decreasing.

Answer:

The function is decreasing where f apostrophe open parentheses x close parentheses less or equal than 0

Find f apostrophe open parentheses x close parentheses

f apostrophe open parentheses x close parentheses equals x cubed plus x squared minus 6 x

Solve the inequality f apostrophe open parentheses x close parentheses less or equal than 0

table row cell x cubed plus x squared minus 6 x end cell less or equal than 0 row cell x open parentheses x squared plus x minus 6 close parentheses end cell less or equal than 0 row cell x open parentheses x plus 3 close parentheses open parentheses x minus 2 close parentheses end cell less or equal than 0 end table

The easiest way to solve a cubic inequality is to graph it, you could use your calculator to help you

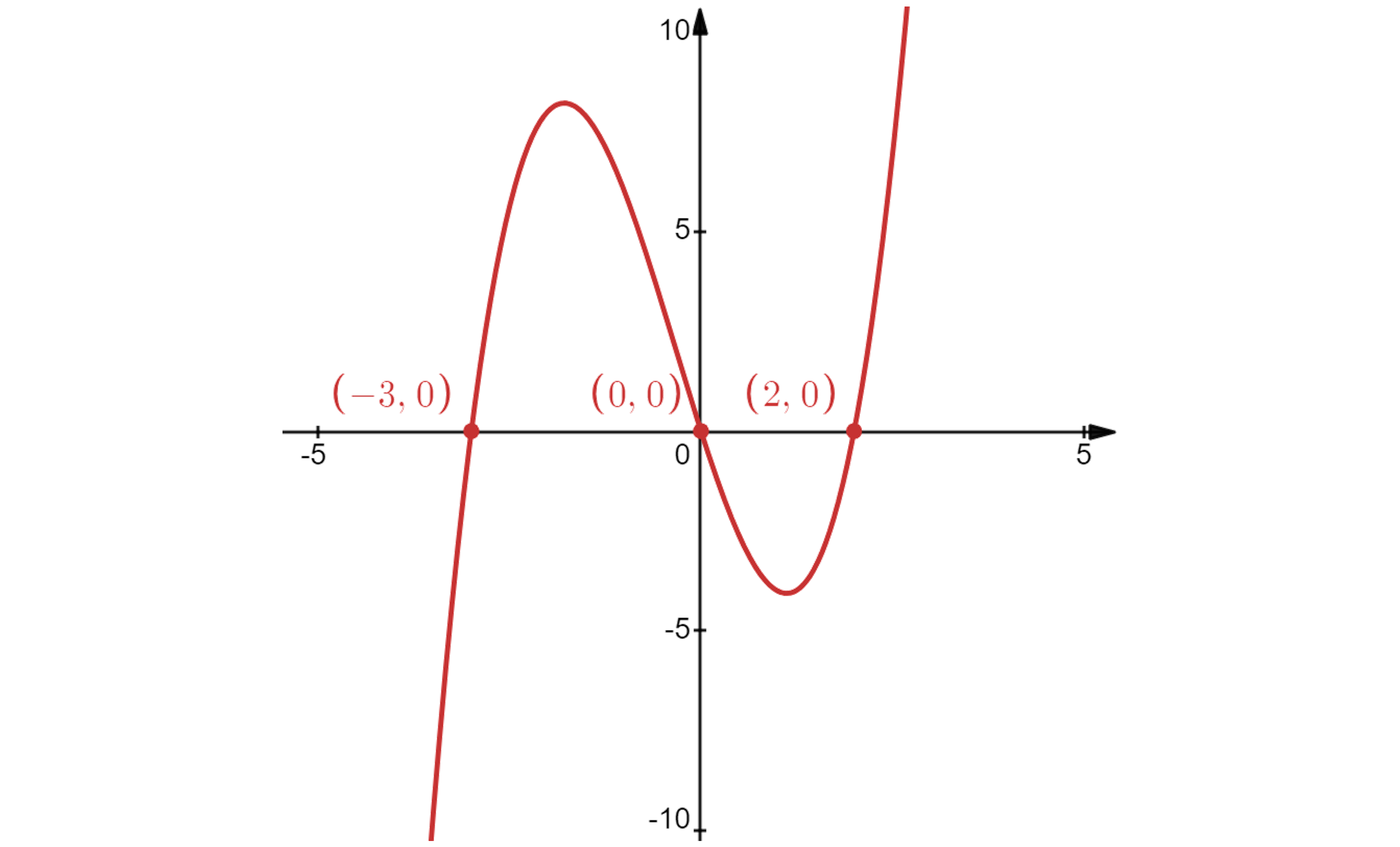
Graph of a cubic function crossing the x-axis at (-3, 0), (0, 0), and (2, 0), and the y-axis at (0, 0), with labeled coordinates.

Use the graph to identify where table row cell x open parentheses x plus 3 close parentheses open parentheses x minus 2 close parentheses end cell less or equal than 0 end table (the parts underneath the x-axis)

x less or equal than negative 3 and 0 less or equal than x less or equal than 2

So these are the regions where f apostrophe open parentheses x close parentheses less or equal than 0, therefore these are the regions where the graph of f open parentheses x close parentheses is decreasing

The question asks for intervals, rather than values of x

Decreasing on the intervals (∞, -3] and [0, 2]

Last updated:

You've read 0 of your 5 free study guides this week

Sign up now. It’s free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

    Test yourself

    Did this page help you?

    Previous:Critical PointsNext:First Derivative Test for Local Extrema
    Author
    Reviewer
    Jamie Wood

    Author: Jamie Wood

    Expertise: Maths

    Jamie graduated in 2014 from the University of Bristol with a degree in Electronic and Communications Engineering. He has worked as a teacher for 8 years, in secondary schools and in further education; teaching GCSE and A Level. He is passionate about helping students fulfil their potential through easy-to-use resources and high-quality questions and solutions.

    Dan Finlay

    Author: Dan Finlay

    Expertise: Maths Lead

    Dan graduated from the University of Oxford with a First class degree in mathematics. As well as teaching maths for over 8 years, Dan has marked a range of exams for Edexcel, tutored students and taught A Level Accounting. Dan has a keen interest in statistics and probability and their real-life applications.