Representations of Equilibrium (College Board AP® Chemistry)

Study Guide

Test yourself

Written by: Fallon

Reviewed by: Stewart Hird

Representations of Equilibrium

Particulate diagrams can be used to represent a reversible reaction over time
When no observable changes occur to the number of particles in a system involving a reversible reaction, the reaction has reached a state of equilibrium
Consider the following reaction over a period of 80 seconds

N₂O₄ (g) ⇌ 2NO₂ (g)

Particulate representation for a reversible reaction

Particulate representation for the reversible reaction N₂O₄(g) ⇌ 2NO₂(g) showing that equilibrium is established when the number of particles of each species remains constant over time

A particulate diagram of a reversible reaction at a certain point in time can be used to calculate K
For the generic equation:

aA + bB ⇌ cC + dD

$K_{c} = \frac{{[C]}^{c} {[D]}^{d}}{{[A]}^{a} {[B]}^{b}}$ $K_{p} = \frac{{(P_{C})}^{c} {(P_{D})}^{d}}{{(P_{A})}^{a} {(P_{B})}^{b}}$

A particulate representation can also be used to calculate Q and determine if the reversible reaction has reached equilibrium
- The equations for Q_c and Q_P are identical to those for K, but Q can be determined using concentration or partial pressure values from any point during the reaction
- At equilibrium, Q = K

Worked Example

A₂(g) + 2B (g) ⇌ A₂B₂(g)

Gases A₂ and B are placed in an empty 1.00 L rigid vessel at a constant temperature of 25°C. The particle diagram below represents the contents of the vessel after equilibrium is established. Assume that each particle in the diagram represents 1 mole. Based on this information, what is the equilibrium constant, K_c, for the reaction above at 25°C?

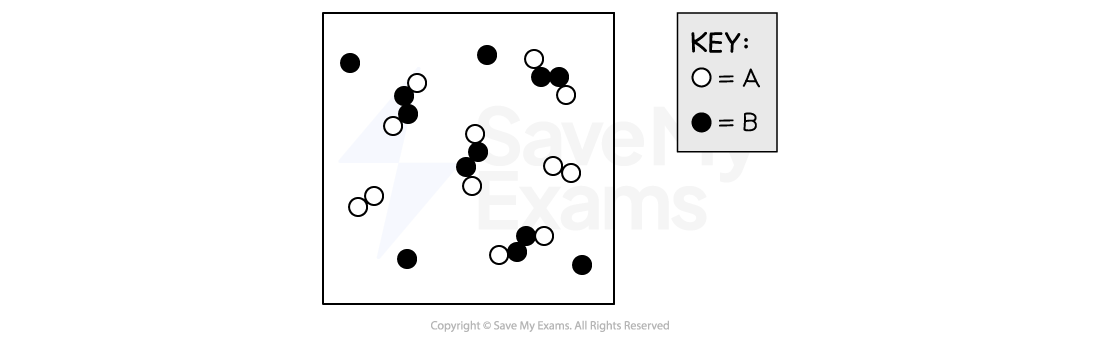
Answer:

For the generic equation

aA + bB ⇌ cC + dD

The equilibrium expression for K_c is

$K_{c} = \frac{{[C]}^{c} {[D]}^{d}}{{[A]}^{a} {[B]}^{b}}$

So the equilibrium expression for K_c for the given reaction is

$K_{c} = \frac{[A_{2} B_{2}]}{[A_{2}] {[B]}^{2}}$

Each particle in the diagram represents 1.00 mole and the total volume of the container is 1.00 L
Therefore, the number of particles of a species in the diagram also represents the concentration of that species at equilibrium in mol/L
The equilibrium concentrations are
- [A₂B₂]_eq = 4.00 mol/L
- [A₂]_eq = 2.00 mol/L
- [B]_eq = 4.00 mol/L
Substituting the concentrations into the equilibrium expression

$K_{c} = \frac{4.00}{2.00 \times {(4.00)}^{2}}$

$K_{c} = \frac{4.00}{2.00 \times 16.00}$

$K_{C} = \frac{4.00}{32.00}$

$K_{C} = 0.125$

Worked Example

2X (g) ⇌ Y (g)

At 25°C, the equilibrium constant, K_c, for the reaction shown above is 15. Which of the following particle diagrams best represents the system at equilibrium? Assume that each particle in the diagrams represents a concentration of 0.1 M.

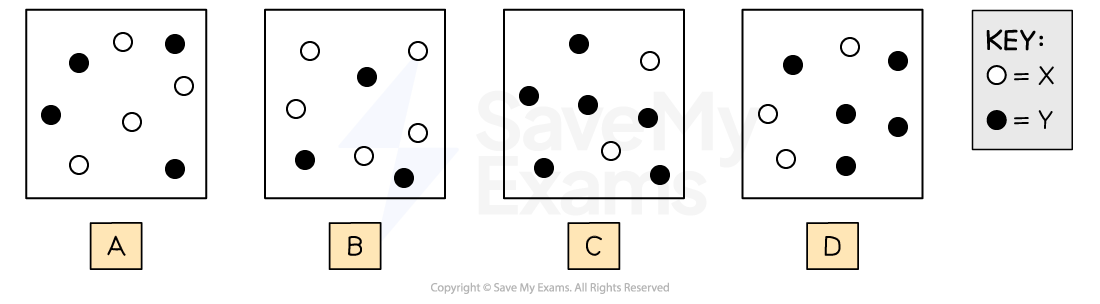
Answer:

Step 1: Determine the expression for Q

For the generic equation

aA + bB ⇌ cC + dD

The equilibrium expression for Q_c is

$Q_{C} = \frac{{[C]}^{c} {[D]}^{d}}{{[A]}^{a} {[B]}^{b}}$

So the expression for Q_c for the given reaction is

$Q_{C} = \frac{[Y]}{{[X]}^{2}}$

Step 2: Solve for Q

Diagram A
- [Y] = 0.4 M
- [X] = 0.4 M

$Q_{C} = \frac{0.4}{0 . 4^{2}}$

$Q_{C} = \frac{0.4}{0.16}$

$Q_{C} = 2.5$

Diagram B
- [Y] = 0.3 M
- [X] = 0.5 M

$Q_{C} = \frac{0.3}{0 . 5^{2}}$

$Q_{C} = \frac{0.3}{0.25}$

$Q_{C} = 1.2$

Diagram C
- [Y] = 0.6 M
- [X] = 0.2 M

$Q_{C} = \frac{0.6}{0 . 2^{2}}$

$Q_{C} = \frac{0.6}{0.04}$

$Q_{C} = 15$

Diagram D
- [Y] = 0.5 M
- [X] = 0.3 M

$Q_{C} = \frac{0.5}{0 . 3^{2}}$

$Q_{C} = \frac{0.5}{0.09}$

$Q_{C} = 5.6$

A system is in equilibrium when Q = K
So diagram C best represents the system at equilibrium

Examiner Tips and Tricks

Be sure to read a particulate diagram question carefully. An individual particle may represent one or more moles of the substance or a specific concentration. In addition, the size of the vessel, while often 1 L, may vary.

Last updated: 25 August 2024

You've read 0 of your 10 free study guides

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Test yourself

Did this page help you?

Previous:Calculating Equilibrium ConcentrationsNext:Le Châtelier’s Principle

Representations of Equilibrium (College Board AP® Chemistry)

Study Guide

Representations of Equilibrium

Particulate representation for a reversible reaction

Worked Example

Worked Example

Examiner Tips and Tricks

You've read 0 of your 10 free study guides

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Fallon

Author: Stewart Hird