Calculating the Equilibrium Constant (College Board AP® Chemistry)

Study Guide

Test yourself

Written by: Fallon

Reviewed by: Stewart Hird

Calculating the Equilibrium Constant

For the generic equation:

aA + bB ⇌ cC + dD

Where a, b, c, and d represent stoichiometric coefficients and A, B, C, and D represent chemical species

The equilibrium expression for K_c is:

$K_{c} = \frac{{[C]}^{c} {[D]}^{d}}{{[A]}^{a} {[B]}^{b}}$

[A] indicates the concentration of species A in mol/L

The equilibrium expression K_P is:

$K_{p} = \frac{{(P_{C})}^{c} {(P_{D})}^{d}}{{(P_{A})}^{a} {(P_{B})}^{b}}$

P_A indicates the partial pressure of species A in atm

Worked Example

H₂(g) + CO₂(g) ⇌ H₂O (g) + CO (g)

A mixture of H₂, CO₂, H₂O, and CO gases is combined in a 1.0 L previously evacuated, rigid sealed container. The gases are allowed to react, according to the equation above, at a constant temperature of 700°C. A graph of the concentration of each gas as a function of time is shown below.

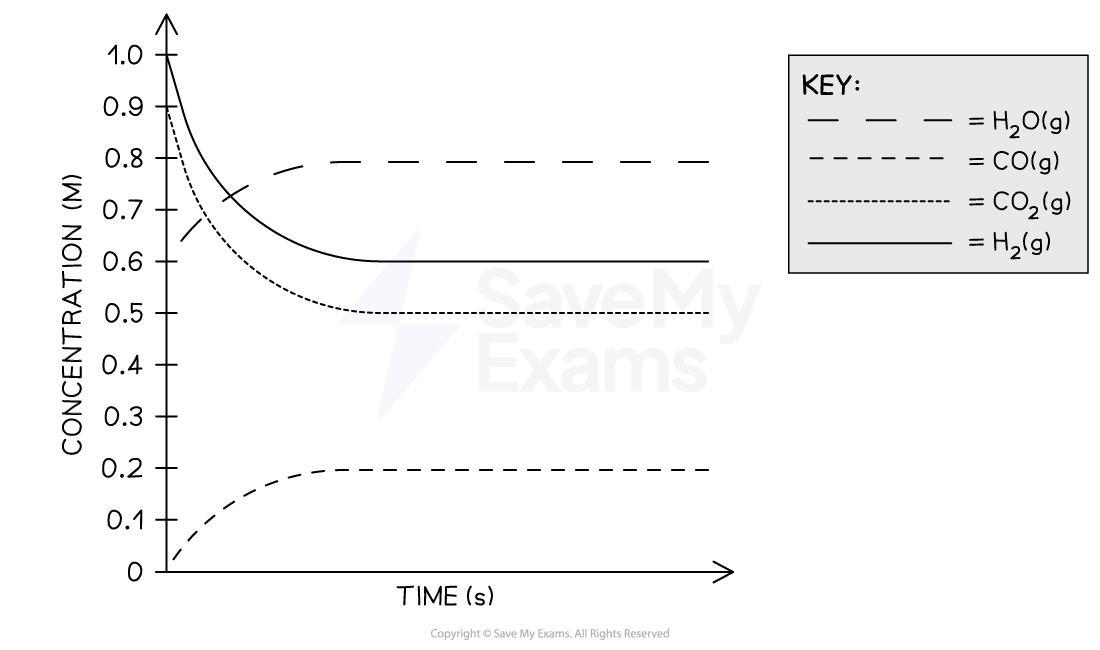
What is the value of K_c for this reaction at 700°C?

Answer:

For the generic equation

aA + bB ⇌ cC + dD

The equilibrium expression for K_c is

$K_{c} = \frac{{[C]}^{c} {[D]}^{d}}{{[A]}^{a} {[B]}^{b}}$

So, for the given reaction the equilibrium expression is

$K_{c} = \frac{[H_{2} O] [CO]}{[H_{2}] [{CO}_{2}]}$

To solve for K_c we substitute the concentration of each species at equilibrium
Equilibrium is established when the concentrations of each species remain constant
From the graph, the equilibrium concentrations are

[H₂O] = 0.8 M

[CO] = 0.2 M

[H₂] = 0.6 M

[CO₂] = 0.5 M

Substituting the concentrations into the equation for K_c

$K_{c} = \frac{0.8 \times 0.2}{0.6 \times 0.5}$

$K_{c} = \frac{0.16}{0.3}$

K_c = 0.5

Note that K_c is a unitless value

Worked Example

2NO₂ (g) ⇌ 2NO (g) + O₂(g)

A sample of pure NO₂ gas in a sealed rigid container decomposes at a constant temperature of 1000 K and a constant pressure of 6.00 atm according to the equation above. At equilibrium, the vessel is found to contain 0.200 mol of NO₂, 1.700 mol of NO, and 1.100 mol of O₂. Calculate the equilibrium constant for partial pressure, K_P, for this reaction.

Answer:

For the generic equation

aA + bB ⇌ cC + dD

The equilibrium expression for K_P is

$K_{p} = \frac{{(P_{C})}^{c} {(P_{D})}^{d}}{{(P_{A})}^{a} {(P_{B})}^{b}}$

For the given reaction the equilibrium expression is

$K_{p} = \frac{{(P_{N O})}^{2} (P_{O_{2}})}{{(P_{N O_{2}})}^{2}}$

To solve for K_P we substitute the partial pressure of each species at equilibrium
Determining the total number of moles of the gas mixture

$n_{total} = n_{({NO}_{2})} + n_{NO} + n_{(O_{2})}$

$n_{total} = 0.200 mol + 1.700 mol + 1.100 mol$

$n_{total} = 3.000 mol$

Determining the partial pressure for each gas using its mole fraction and total pressure

NO₂

$P_{{NO}_{2}} = \frac{n_{{NO}_{2}}}{n_{total}} \times P_{total}$

$P_{{NO}_{2}} = \frac{0.200 mol}{3.000 mol} \times 6.00 atm$

$P_{{NO}_{2}} = 0.400 atm$

$P_{NO} = \frac{n_{NO}}{n_{total}} \times P_{total}$

$P_{NO} = \frac{1.700 mol}{3.000 mol} \times 6.00 atm$

$P_{NO} = 3.40 atm$

O₂

$P_{O_{2}} = \frac{n_{O_{2}}}{n_{total}} \times P_{total}$

$P_{O_{2}} = \frac{1.100 mol}{3.000 mol} \times 6.00 atm$

$P_{O_{2}} = 2.20 atm$

Substituting the partial pressures into the equation for K_P

$K_{P} = \frac{{(3.40)}^{2} \times 2.20}{{(0.400)}^{2}}$

$K_{P} = \frac{11.56 \times 2.20}{0.16}$

KP=25.4320.16

KP=159

Note that K_P is a unitless value

Examiner Tips and Tricks

When writing a K_c expression, always be sure to include the brackets, as brackets around a species represent the concentration of that species. However, when writing a K_P expression never use brackets as this expression involves partial pressures not concentrations.

Make sure that the values substituted into a K_c expression have the unit mol/L and values substituted into a K_P expression have the unit atm.

Remember that although the values substituted into a K_cor K_P expression have units, the equilibrium constant is a unitless value.

Last updated: 25 August 2024

You've read 0 of your 10 free study guides

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

Test yourself

Did this page help you?

Previous:The Reaction QuotientNext:Magnitude of the Equilibrium Constant

Calculating the Equilibrium Constant (College Board AP® Chemistry)

Study Guide

Calculating the Equilibrium Constant

Worked Example

Worked Example

Examiner Tips and Tricks

You've read 0 of your 10 free study guides

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

Author: Fallon

Author: Stewart Hird