Properties of Photons (College Board AP® Chemistry): Revision Note

Photons

• One of the main characteristics of light is its wave-particle duality

• This means that light can behave as a wave, but also as a particle

• As seen in previous sections, a light wave has frequency, wavelength and energy

• However, the discovery of the photoelectric effect suggested that light can also behave as a particle

• The photoelectric effect was observed when some metals emit electrons when light was shone on them

  • The results of this experiment suggested that light must come into particles

• A light particle is called a photon

Absorption and emission of photons

• Electrons move around the nucleus of the atoms in energy levels

• Atoms and molecules can absorb photons, and the electrons will jump to higher energy levels

  • When this occurs, the energy of the atom or molecule increases by an amount equal to the energy of the photon

• The excited atom or molecule can then emit a photon as the electron falls back to a lower energy level, often all the way to the lowest one, the ground state

  • When this occurs, the energy of the atom or molecule will decrease by an amount equal to the energy of the photon

Absorption and emission diagram

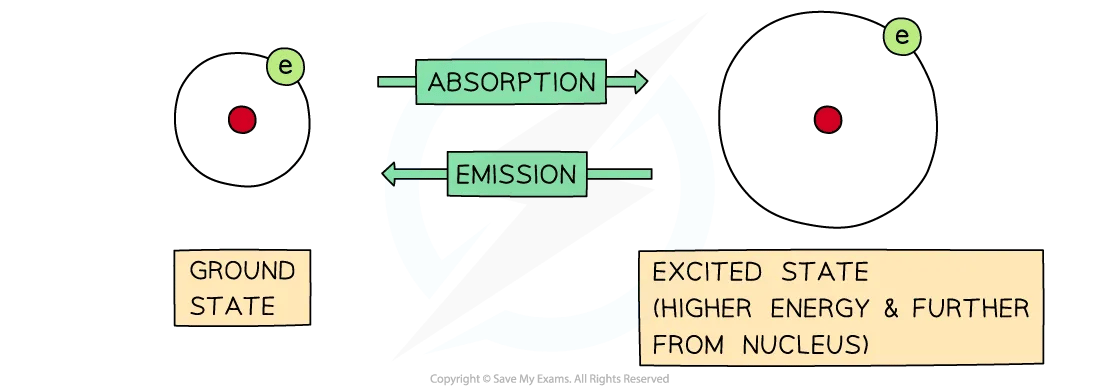
The difference between absorption and emission depends on whether electrons are jumping from lower to higher energy levels or the other way around

The hydrogen emission spectrum

• The hydrogen emission spectrum is a set of separate lines; Niels Bohr’s model explained each line as a photon released when an electron falls from a higher energy level to a lower one

• Bohr suggested that electrons can only exist in fixed energy levels

  • This means that electronic transitions can only occur when photons with a specific amount of energy are absorbed or released

• Each electronic transition has to do with photons from specific regions of the electromagnetic spectrum

Electron transitions in the hydrogen atom 

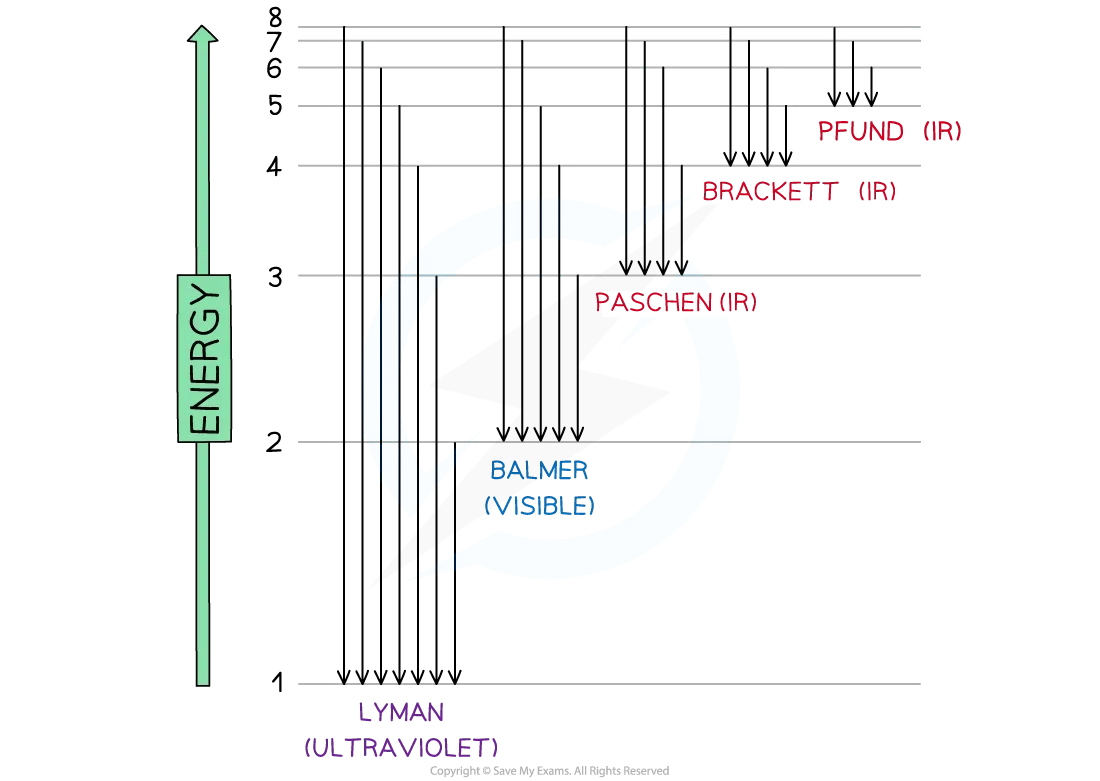
Electron jumps in the hydrogen spectrum

• The table summarizes these jumps:

Electron transitions summary

Energy of Photons

Frequency, wavelength and energy

• As the electromagnetic spectrum shows, the lower the frequency of an electromagnetic wave, the longer its wavelength

  • Frequency (ν) and wavelength (λ) are inversely proportional

  • The equation that links them is:

 $c=\lambda v$

• In this equation, c is the speed of light, 2.998 × 10⁸ m s^-1

• The lower the frequency, the lower the energy of each photon

  • Frequency (ν) and energy (E) are directly proportional

  • The equation that links the energy emitted or absorbed by a photon and its frequency is:
                                                    $E=hv$

  • In this equation, h is Planck’s constant, 6.626 × 10^-34 J s

• These two equations can be used to calculate frequency, wavelength or energy when one of them is given