Molarity (College Board AP® Chemistry) : Study Guide
Molarity
A solution may be described qualitatively or quantitatively
Qualitatively, the terms dilute and concentrated are used to describe the amount of solute in a given quantity of the solvent
A solution with a relatively small concentration of solute is said to be dilute
A solution with a large concentration of solute is said to be concentrated
Quantitatively, a solution is described in terms of its concentration
Molarity
Molarity (M) expresses the concentration of a solution as the number of moles of solute in a litre of solution:
M = n / V
A 1.00 molar solution (1.00 M) contains 1.00 mol of solute in every 1.0 L of solution
A solution can be prepared to a specified molarity by weighing out the calculated mass of solute and dissolving it in enough solvent to form the desired volume of solution
For example, to prepare 250.0 mL of a 1.00 M solution of CuSO4:
Determine the number of moles of the solute (CuSO4) required
In this case, this is given as n = Molarity × Volume
n = 1.00 × 0.250 = 0.250 mol
Determine and weigh the corresponding mass of CuSO4 required
Using the expression: Mass = n × Mr
The molar mass (Mr) of CuSO4 is calculated as:
Mr = (Mr)cu + (Mr)S + 4(Mr)O
Mr = 63.6 + 32.0 + 4 (16) = 159.6 g/mol
Mass = 0.250 mol × 159.6 g/mol = 39.9 g
Transfer 39.9 g of CuSO4 into a 250 mL flask and add some quantity of water to dissolve the solute
Add more water until the solution reaches the calibrated mark of the flask
Preparing a Solution
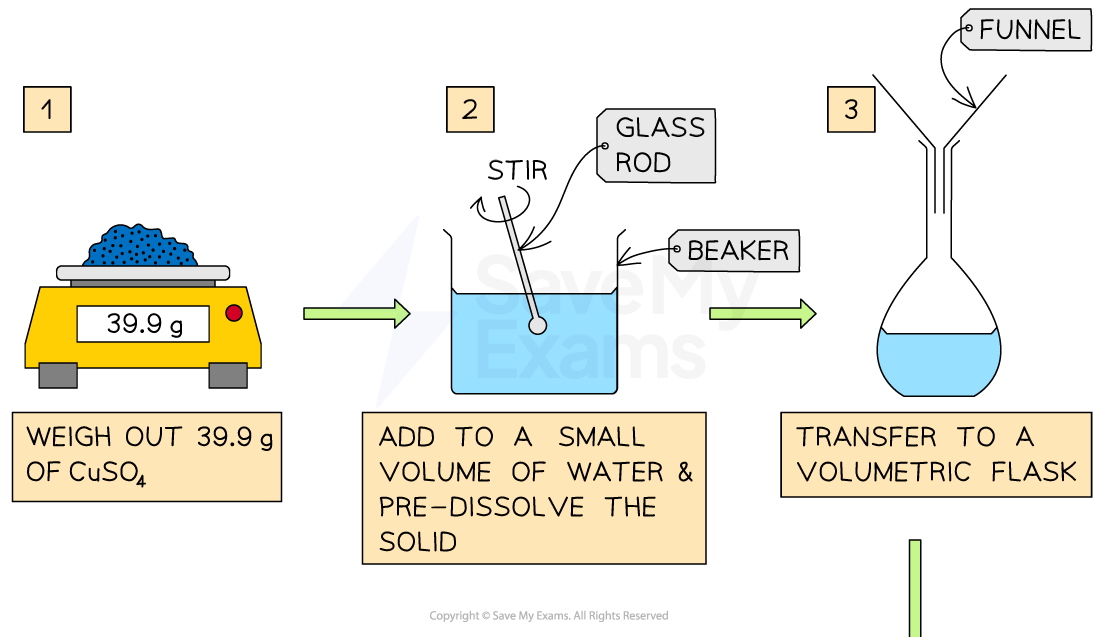
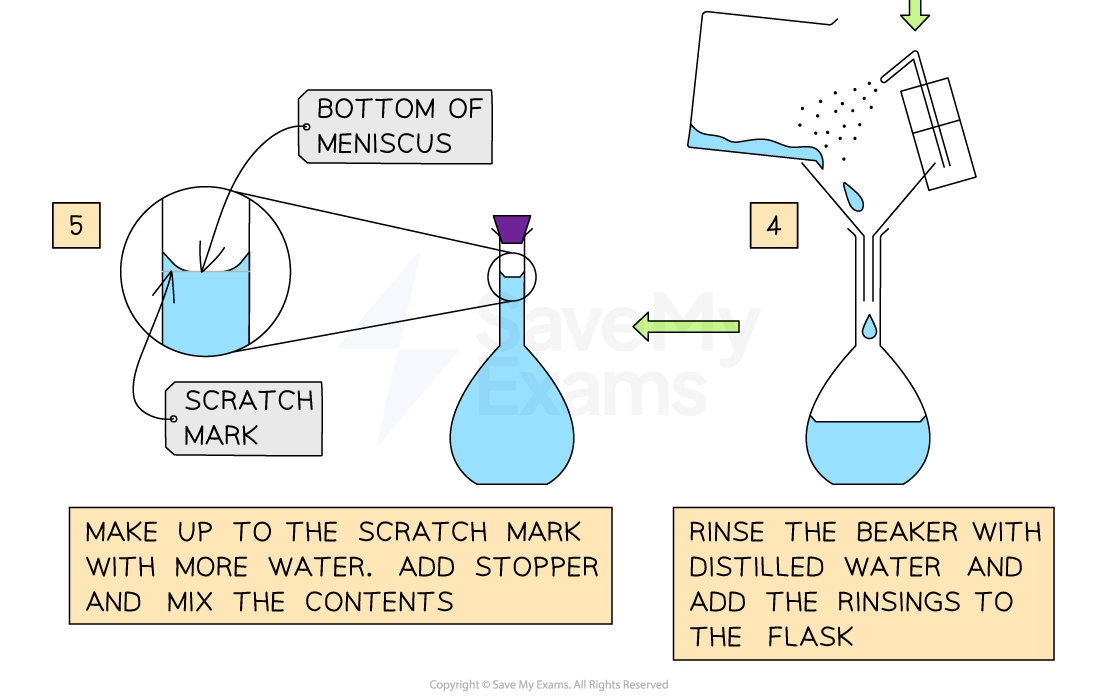
How to prepare a 250mL of 1.00 M solution of CuSO4
Dilution
Alternatively, you can start with a more concentrated solution, called the stock solution, and dilute it with water to give a solution of the desired molarity
The calculations are straightforward if you keep a simple point in mind: Adding solvent cannot change the number of moles of solute
That is:
nsolute (stock solution) = nsolute (dilute solution)
In both solutions, n can be found by multiplying the molarity, M, by the volume in litres, V
Hence, a dilution expression can be obtained:
Ms × Vs = Md × Vd
Where the subscripts s and d stand for stock and dilute solutions, respectively
Worked Example
Calculate the molarity of a solution made by dissolving 5.00 g of glucose (C6H12O6) in sufficient water to form exactly 100 mL of solution. (Mr glucose = 180.2 g/mol)
Answer:
Analyse: We are provided with the mass of glucose and the volume of solution and asked to determine the molarity of the solution
Plan: To determine the molarity of the solution the following steps are required:
Step 1: Convert the mass of glucose to moles and the volume of the solution to litres
n = Mass/Molar Mass
n = 5.00/180.2
n = 0.0277 moles
Volume = 100/1000
Volume = 0.1L
Step 2: Substitute the values of the moles of glucose and the volume of the solution
Molarity (M) = number of moles/volume of solution
Molarity (M) = 0.0277/0.1
Molarity (M) = 0.277 M
Worked Example
How many grams of Na2SO4 are there in 15 mL of 0.50 M Na2SO4?
How many millilitres of 0.50 M Na2SO4 solution are needed to provide 0.038 mol of this salt?
Analyse:
In part a, we are given the volume of the solution and the concentration (Molarity)
We are then asked to determine the mass of the solute(Na2SO4) required
In part b, we are given the number of moles of solute and the molarity of the solution
We are then asked to determine the volume of solution in millilitres
Plan:
For part a:
Use the molarity expression to determine the number of moles of the solute (n)
Convert this number of moles to mass
For part b:
Use the molarity expression to determine the volume of solution in litres
Convert to millilitres using the appropriate conversion factor
Answer:
Part a:
Step 1: Convert volume in mL to L:
Volume of solution = 15/1000 = 0.015L
Step 2: Rearrange the concentration expression in terms of the number of Na2SO4 (n):
n = M × V
n = 0.50 × 0.015
n = 0.0075 moles
Step 3: Using the mole-molar mass expression, convert the moles of solute to mass:
MNa2SO4 = n × Mr
MNa2SO4 = 0.0075 × 142.04
MNa2SO4 = 1.1g
Part b:
Step 1: Rearrange the concentration expression in terms of the volume of solution:
V = n/M
V = 0.038/0.50
V = 0.076 L
Step 2: Convert volume in L to mL:
V = 0.076 × 1000
V = 76 mL
Worked Example
How many millilitres of 5.0 M Na2Cr2O7 solution must be diluted to prepare 250 mL of 0.10 M solution? How much water needs to be added to this concentrated solution?
Analyse:
We are given the volume and concentration of the diluted solution and asked to determine the volume of the concentrated solution required to prepare
We are then asked the volume of water required to make the dilute solution
Plan:
Using the dilution formula, we can calculate the volume of the concentrated solution required
Then subtract that volume from the volume of the dilute solution to obtain the volume of water required
Answer:
Step 1: Using M1V1 = M2V2
Rearrange the equation to V1 = (M2V2)/ M1
V1 = ?
M1 = 5.0 M
V2 = 250 mL
M2 = 0.10 M
So, V1 = (0.10 × 250)/5.0
V1 = 5.0 mL
Step 2: The volume of water added will be:
VH2O = 250 - 5.0
VH2O = 245 mL

You've read 0 of your 5 free study guides this week
Unlock more, it's free!
Did this page help you?

