A LevelPhysicsOCRExam Questions5. Newtonian World & Astrophysics5.8 Planetary Motion

Planetary Motion (OCR A Level Physics)

Exam Questions

39 mins8 questions

Select a question set to download for
5.8 Planetary Motion

Select an answer set to view for
5.8 Planetary Motion

Medium
Hard
1a2 marks
a)
The diagram below shows the Earth in space.

 
 
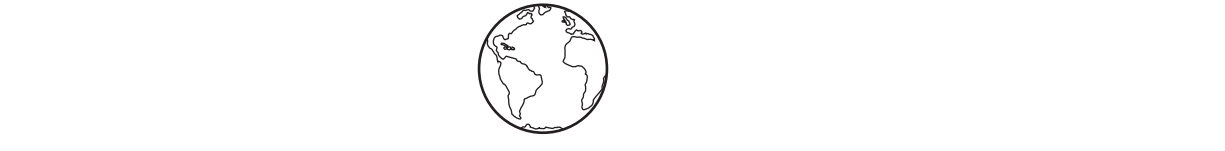
q20a-paper-1-nov-2021-ocr-a-level-physics
 
 
 
i)
On the diagram above, draw a minimum of four gravitational field lines to map out the gravitational field pattern around the Earth.

[1]

ii)
On the same diagram above, show two different points where the gravitational potential is the same. Label these points X and Y.

[1]

How did you do?

1b6 marks
b)
A satellite is in a circular geostationary orbit around the centre of the Earth. The satellite has both kinetic energy and gravitational potential energy.

The mass of the satellite is 2500 kg and the radius of its circular orbit is 4.22 × 107m.
The mass of the Earth is 5.97 × 1024 kg.

• Describe some of the features of a geostationary orbit.
• Calculate the total energy of the satellite in its geostationary orbit.

[6]

How did you do?

Did this page help you?

2a6 marks
a)
Phobos is one of the two moons orbiting Mars. Fig. 17.1 shows Phobos and Mars.

q17a-paper-1-june-2018-ocr-a-level-physics
Fig. 17.1

The orbit of Phobos may be assumed to be a circle. The centre of Phobos is at a distance 9380 km from the centre of Mars and it has an orbital speed 2.14 × 103 m s−1.



i)
On Fig. 17.1, draw an arrow to show the direction of the force which keeps Phobos in its orbit.

[1]
ii)
Calculate the orbital period T of Phobos.


T = ....................................................... s [2]

 

iii)
Calculate the mass M of Mars.


M = ..................................................... kg [3]

How did you do?

2b6 marks
b)
The gravitational field strength at a distance r from the centre of Mars is g.

The table below shows some data on Mars.
g / N kg−1 r / km lg (g / N kg−1) lg (r / km)
1.19 6000 0.076 3.78
0.87 7000    
0.67 8000 −0.174 3.78
0.53 9000 −0.276 3.95
0.43 10000 −0.367 4.00


i)
Complete the table by calculating the missing values.
[1]



ii)
Fig. 17.2 shows the graph of lg (g / N kg−1) against lg (r / km).

q17b-ii-paper-1-june-2018-ocr-a-level-physics

Fig. 17.2

1
Plot the missing data point on the graph and draw the straight line of best fit.
[2]

2
Use Fig. 17.2 to show that the gradient of the straight line of best fit is −2.

[1]

3
Explain why the gradient of the straight line of best fit is −2.

[2]

How did you do?

2c4 marks
c)
In July 2018, the closest distance between the centre of Mars and the centre of Earth was 5.8 × 1010 m.
 
Fig. 17.3 shows the variation of the resultant gravitational field strength g between the two planets with distance r from the centre of the Earth.

q17c-paper-1-june-2018-ocr-a-level-physics
Fig. 17.3

i)
Explain briefly the overall shape of the graph in Fig. 17.3.

 [2]

ii)
Use the value of r when g = 0 from Fig. 17.3 to determine the ratio
 
fraction numerator m a s s italic space o f italic space E a r t h over denominator m a s s italic space o f italic space M a r s end fraction

 

fraction numerator m a s s italic space o f italic space E a r t h over denominator m a s s italic space o f italic space M a r s end fraction = ......................................................... [2]

How did you do?

Did this page help you?

1a7 marks

A binary star is a pair of stars which move in circular orbits around their common centre of mass.

In this question consider the stars to be point masses situated at their centres.

a)
 Fig. 3.1 shows a binary star where the mass of each star is m. The stars move in the same circular orbit.

q3a-paper-3-june-2018-ocr-a-level-physics
Fig. 3.1

i)
Explain why the stars of equal mass must always be diametrically opposite as they travel in the circular orbit.

[2]

ii)
The centres of the two stars are separated by a distance of 2R equal to 3.6 × 1010 m, where R is the radius of the orbit. The stars have an orbital period T of 20.5 days. The mass of each star is given by the equation

m equals fraction numerator 16 space straight pi squared space R cubed over denominator G T squared end fraction

where G is the gravitational constant.

Calculate the mass m of each star in terms of the mass MΘ of the Sun.

1 day = 86400 s
MΘ = 2.0 × 1030 kg





m = .......................................... MΘ [3]

iii)
The stars are viewed from Earth in the plane of rotation.

The stars are observed using light that has wavelength of 656 nm in the laboratory. The observed light from the stars is Doppler shifted.

Calculate the maximum change in the observed wavelength Δλ of this light from the orbiting stars. Give your answer in nm.





Δλ = .................................. nm [2]

How did you do?

1b3 marks
b)
Fig. 3.2 shows a binary star where the masses of the stars are 4m and m.

q3b-paper-3-june-2018-ocr-a-level-physics

Fig. 3.2

i)
The centre of mass of the binary star lies at the surface of the star of mass 4m. Draw on Fig. 3.2 two circles to represent the orbits of both stars.

[1]

ii)
Explain why the smaller mass star travels faster in its orbit than the larger mass star.

[2]

How did you do?

Did this page help you?