A LevelPhysicsOCRRevision Notes6. Particles & Medical PhysicsRadioactivityHalf-Life

Half-Life (OCR A Level Physics): Revision Note

Exam code: H556

Written by: Katie M

Reviewed by: Caroline Carroll

Updated on 7 October 2026

Guided study available on this topic

Understand this topic with deeper explanations and understanding checks.

Half-Life

Half life is defined as:

The time taken for the number of undecayed nuclei to halve

This means that when a time equal to the half-life has passed, the activity of the sample will be half of its original value
This is because activity is proportional to the number of undecayed nuclei, $A \propto N$

Graph of activity A against time t showing an exponential decay curve starting at A₀. After one half-life, t½, the activity is A₀/2, and after two half-lives, 2t½, it is A₀/4.

When a time equal to the half-life passes, the activity falls by half, when two half-lives pass, the activity falls by another half (which is a quarter of the initial value)

To find an expression for half-life, start with the equation for exponential decay:

$N = N_{0} e^{- λ t}$

Where:
- N = number of nuclei remaining in a sample
- N₀ = the initial number of undecayed nuclei (when t = 0)
- λ = decay constant (s^-1)
- t = time interval (s)

When time $t$ is equal to the half-life $t_{1 / 2}$ , the activity $N$ of the sample will be half of its original value, so $N = \frac{1}{2} N_{0}$

$\frac{1}{2} N_{0} = N_{0} e^{- λ t_{1 / 2}}$

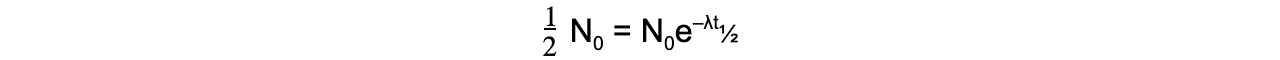
The formula can then be derived by first dividing both sides by $N_{0}$ :

$\frac{1}{2} = e^{- λ t_{1 / 2}}$

Then, taking the natural log of both sides:

$\ln (\frac{1}{2}) = \ln (e^{- λ t_{1 / 2}})$

Apply the property of logarithms:
- $\ln (e^{x}) = x$ , the natural log is the inverse of the exponential

$\ln (e^{- λ t_{1 / 2}}) = - λ t_{1 / 2}$

Simplify:

$\ln\left(\frac{1}{2}\right)\ =\ \ln\left(2^{-1}\right)\ =\ -\ln\left(2\right)$

$- λ t_{1 / 2} = - \ln 2$

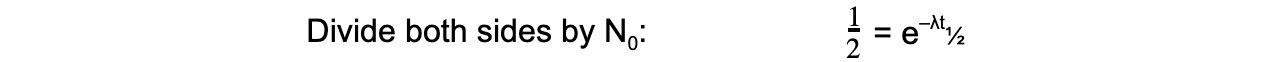
Therefore, half-life $t_{1 / 2}$ can be calculated using the equation:

$t_{1 / 2} = \frac{\ln 2}{λ} ≃ \frac{0.693}{λ}$

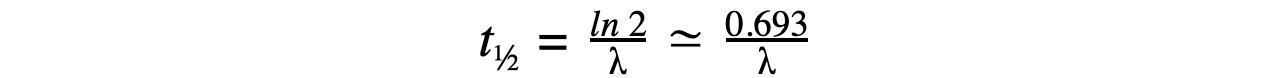
This equation shows that half-life $t_{1 / 2}$ and the radioactive decay rate constant λ are inversely proportional
- Therefore, the shorter the half-life, the larger the decay constant and the faster the decay

Worked Example

Strontium-90 is a radioactive isotope with a half-life of 28.0 years. A sample of strontium-90 has an activity of 6.4 × 10⁹ Bq.

Calculate the decay constant $λ$ , in s^–1, of strontium-90.

Answer:

Step 1: Convert the half-life into seconds

$t_{1 / 2}$ = 28 years = 28 × (365 × 24 × 60 × 60) = 8.83 × 10⁸ s

Step 2: Write the equation for half-life

$t_{1 / 2} = \frac{\ln 2}{λ}$

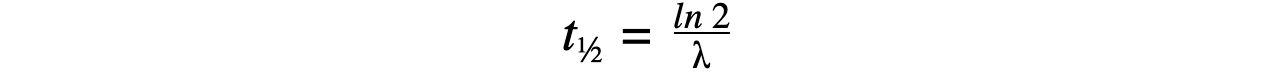
Step 3: Rearrange for λ and calculate

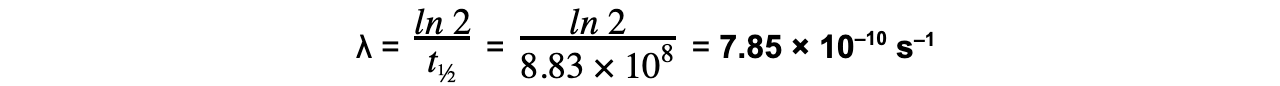
$λ = \frac{\ln 2}{8.83 \times 10^{8}} = 7.85 \times 10^{- 10} s^{- 1}$

Examiner Tips and Tricks

Make sure you are confident with the meanings of all the definitions and symbols in this unit. It is easy to get confused when completing an examination question.

Determining the Half-Life of an Isotope

Aim of the experiment

The aim of this experiment is to determine the half-life of an isotope such as protactinium

Variables:

Independent variable = time, $t$ (s)
Dependent variable = corrected count rate, $C$
Control variables:
- Radioactive source
- Distance of GM tube to source
- Location / background radiation

Equipment list

Equipment	Purpose
sealed bottle containing uranium salt solution and protactinium-234	to use as a source of radioactive emission
Geiger-Muller tube and counter or datalogger	to measure the count rate of the radioactive source
stopwatch	to measure the same time interval for each count rate reading
tray lined with paper towel	to avoid contamination should the bottle leak

Method

Apparatus for determining the half-life of protactinium-234

A setup with a sealed bottle containing an organic layer with protactinium on the top and a uranyl nitrate layer on the bottom. A GM tube connected to a Geiger counter is aligned with the organic layer of the bottle.

In the lower layer of the sealed bottle, uranium-238 decays to thorium-234 and then to protactinium-234. Shaking the bottle separates the isotopes as protactinium dissolves in the organic solvent, whereas thorium does not.

Connect the Geiger-Müller tube to the counter and, without any sources present, measure background radiation over a period of one minute
Repeat this three times, and take an average. Subtract this value from all subsequent readings
Shake the bottle gently for 15 seconds to dissolve the protactinium in the organic layer, then wait for it to float to the top
- This is done because the uranium salt in the aqueous layer decays to form protactinium, which is soluble in the organic solvent but insoluble in the aqueous layer
Align the GM tube with the organic layer and immediately start a stopwatch
Record the number of counts in 10 seconds, and repeat every 30 seconds

A suitable table of results might look like this:

Time, $t / s$	Count rate $/ counts s^{- 1}$	Corrected count rate, $C / counts s^{- 1}$
0
30
60
90
120
150

Analysis of results

The count rate of the source decreases exponentially according to

$C = C_{0} e^{- λ t}$

Taking the natural logs of both sides

$\ln C = \ln (C_{0}) - λ t$

Compared to the equation of a straight line $(y = m x + c)$ :
- y-axis variable, $y = \ln C$
- x-axis variable, $x = t$
- gradient = $- λ = - \frac{\ln 2}{t_{1 / 2}}$
- y-intercept = $\ln (C_{0})$

Subtract the background radiation from each count rate reading to give the corrected count rate $C$
Plot a graph of $\ln C$ against time $t$
Determine the half-life $t_{1 / 2}$ from the gradient of the best-fit line

$t_{1 / 2} = - \frac{\ln 2}{gradient}$

Log graph of corrected count rate against time

Graph of ln C versus time, showing a negative linear slope. The initial value is ln C₀ and the gradient is labelled as negative lambda.

By plotting a log graph of ln C against time, the decay constant and half-life can be found directly from the gradient

Evaluating the experiment

Systematic Errors:

Align the GM tube with the top (organic) layer, close to the bottle but not touching it
- The protactinium is in the top layer, so the count rate is mainly due to beta particles from the protactinium; alpha particles from the uranium are absorbed by the bottle wall

Random Errors:

Radioactive decay is random, so repeat readings are vital in this experiment
The half-life of protactinium is short, so more frequent readings of count rate may be necessary

Safety considerations

The short half-life of protactinium-234 means its activity falls quickly, but the bottle also contains long-lived uranium, so it must still be handled with care
Ensure the bottle remains sealed at all times to prevent leakage
Handle the bottle with long tongs
Safety clothing such as a lab coat, gloves and goggles must be worn

Unlock more, it's free!

Join the 100,000+ Students that ❤️ Save My Exams

the (exam) results speak for themselves:

I would just like to say a massive thank you for putting together such a brilliant, easy to use website.I really think using this site helped me secure my top gradesin science and maths. You really did save my exams! Thank you.

Beth
IGCSE Student

This website is soooo useful and I can’t ever thank you enough for organising questions by topic like this. Furthermore, the name of the website could not have been more appropriate as it literally did SAVE MY EXAMS!

Fathima
A Level Student

Incredible! SO worth my money, the revision notes have everything I need to know and are so easy to understand. I actually enjoy revising! It makes me feel a lot more confident for my GCSEs in a few months.

Kate
GCSE Student

Absolutely brilliant, both my girls used it for A levels and GCSE. It's saves on paper copies, also beneficial exam questions ranked from easy to hard. It's removed a lot of stress from the exams.

Sameera
Parent

Just to say that your resources are the best I have seen and I have been teaching chemistry at different levels for about 40 years

Mark
Chemistry Teacher

Excellent