Electric Potential Energy (OCR A Level Physics)

• When a mass with charge moves through an electric field, work is done
• The work done in moving a charge q is given by:

∆W = q∆V

• Where:
  • ∆W = change in work done (J)
  • q = charge (C)
  • ∆V = change in electric potential (J C⁻¹)

• This change in work done is equal to the change in electric potential energy (E.P.E)
  • When V = 0, then the E.P.E = 0

• The change in E.P.E, or work done, for a point charge q at a distance r₁ from the centre of a larger charge Q, to a distance of r₂ further away can be written as:

• Where:
  • Q = charge that is producing the electric field (C)
  • q = charge that is moving in the electric field (C)
  • r₁ = first distance of q from the centre of Q (m)
  • r₂ = second distance of q from the centre of Q (m)

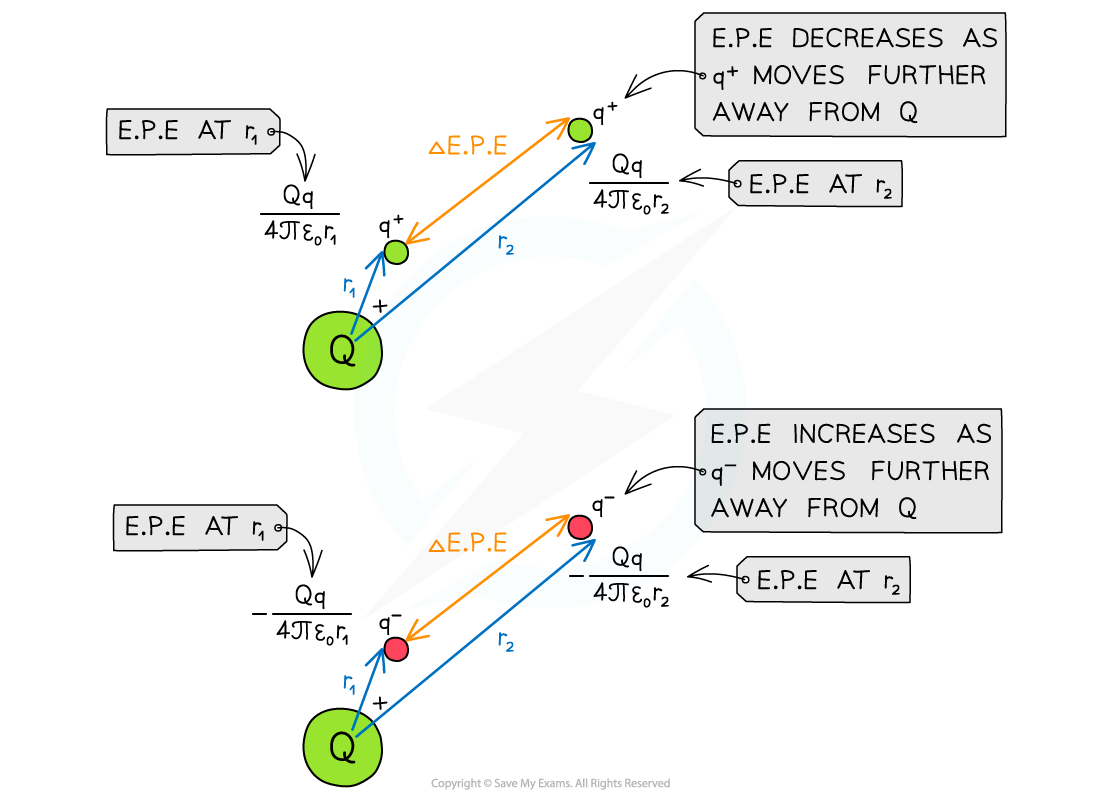
Work is done when moving a point charge away from another charge

• Work is done when a positive charge in an electric field moves against the electric field lines or when a negative charge moves with the electric field lines

Step 1: Write down the known quantities

• • p.d. at R, V₁ = 675 V
  • p.d. at S, V₂ = 850 V
  • Charge, q = +3.0 nC = +3.0 × 10⁻⁹ C

Step 2: Write down the work done equation

W = qΔV

Step 3: Substitute in the values into the equation

W = (3.0 × 10^-9) × (850 - 675) = 5.3 × 10⁻⁷ J